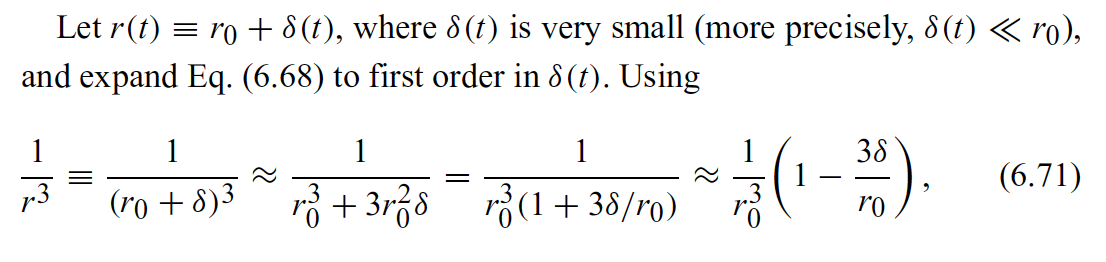
From Morin's Classical Mechanics, on the chapter of Small Oscillations in Lagrangian Mechanics, he does this approximation on the last equality, I don't understand what happened there.
I get the first approximation, where we neglect the terms of order higher δ^2.