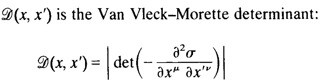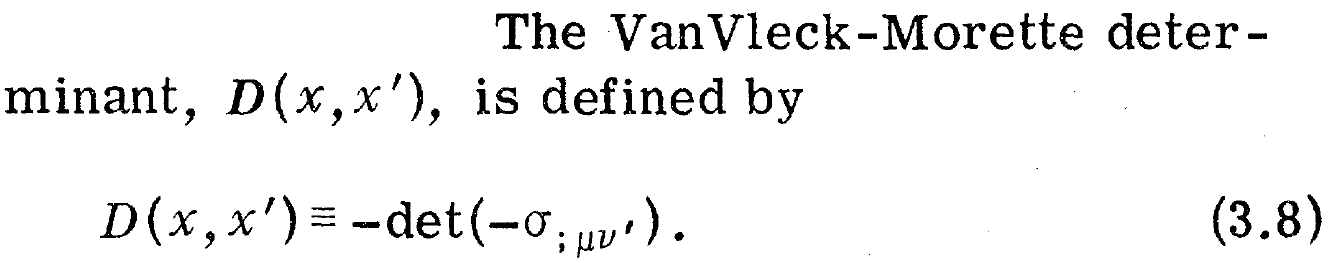1
$\begingroup$

Let $M$ be a Riemannian manifold and $\sigma$ the world function. The Van-Vleck-Morette determinant $D$ is defined by $$D(x,x')=\det(-\sigma_{;\mu\nu{}'})$$ Regarding the semi-colon: In chapter $4.1$ of [K] it is claimed that it "denotes differentiation with respect to the Levi-Civita connection", but in other references it is simply interpreted as partial differentiation$^1$: $$\sigma_{;\mu\nu{}'}=\frac{\partial^2\sigma}{\partial x^\mu\partial(x')^\nu}$$ In any case, it is not clear to me why $D$ is supposedly coordinate-independent (even if we restrict ourselves to normal coordinates), as it is claimed here.

$^1$

From chapter $4.1$ of [K]:

enter image description here

From page $38$ of [B]:

enter image description here

From [C]:

enter image description here

References

[K] Klaus Kirsten, Spectral Functions in Mathematics and Physics

[B] Barvinsky and Vilkovisky, The generalized Schwinger-Dewitt technique in gauge theories and quantum gravity

[C] Steven Christensen, Vacuum expectation value of the stress tensor in an arbitrary curved background: The covariant point-separation method

Improve this question
$\endgroup$
10
  • 1
    $\begingroup$ The semicolon is a somewhat common notation for the covariant derivative w.r.t. the LC connection. Other sources should not interpret this as "partial differentiation" - I assume they write what you wrote for $\sigma_{;\mu\nu'}$ inside of $\mathrm{det}$ and then there should be factors of $\sqrt{g}$ in front of the determinant corresponding to the difference between the partial and the covariant derivative. Is this correct? If not, please name at least one of these "other references" explicitly. $\endgroup$
    – ACuriousMind ♦
    Commented Apr 16, 2023 at 11:28
  • $\begingroup$ @ACuriousMind "The semicolon is a somewhat common notation for the covariant derivative w.r.t. the LC connection." - Could you please explain what that means? AFAIK the LC connection acts on vector fields and $\sigma$ is scalar-valued... $\endgroup$
    – Filippo
    Commented Apr 16, 2023 at 12:12
  • $\begingroup$ Most texts extend the covariant derivative to act on arbitrary tensor fields, not just vector fields, with the covariant derivative of scalars coinciding with their ordinary derivative (cf. e.g. Wikipedia). When you have an expression $f_{;\mu\nu}$, then the first derivative will be just a partial derivative but since the second one then acts on the vector field $f_{;\mu}$, the difference matters. $\endgroup$
    – ACuriousMind ♦
    Commented Apr 16, 2023 at 12:19
  • 2
    $\begingroup$ @ACuriousMind … but since the second one then acts on the vector field $f_{;μ}$, the difference matters No. $σ$ is a biscalar, i.e. it depends on two points $(x,x')$, the first differentiation is for the first point argument, while the second diff is for the second point argument, so $σ_{;μ}$ is not a covector at $x'$. $\endgroup$
    – A.V.S.
    Commented Apr 16, 2023 at 12:35
  • $\begingroup$ @ACuriousMind I agree that I can apply the Levi-Civita-connection to the differential of $\sigma$, but the expression $(\nabla\mathrm{d}\sigma)_{\mu\nu{}'}$ does not make sense (since we are considering two tangent vectors in different points), does it? $\endgroup$
    – Filippo
    Commented Apr 16, 2023 at 12:44

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.