Do microstates "transition" during quasi-static processes?

Question

I am currently reading Reif's Fundamentals of Statistical and Thermal Physics and I am unfortunately rather baffled at the end of Chapter 2 which Reif wraps up with a discussion of quasi-static processes. At the bottom of page 75, a quasi-static process with respect to some system $A$ is defined as (I paraphrase)

a process (involving the performance of work, exchange of heat, or both) which is carried out slowly relative to the relevant relaxation times for $A$ such that $A$ is approximately in the equilibrium appropriate to the external parameters and conditions enforced on $A$ at that instant.

Reif then goes on (on page 75) to show that the infinitesimal work $\delta W$ can be computed using the mean generalized forces conjugate to the relevant external parameters; but, in this derivation, it is assumed that a given microstate remains in that state (evolves adiabatically in the quantum mechanical sense). In contrast, Reif remarks just later that, even in a quasi-static process with no thermal interactions (only work), it is not true that evolution occurs such that states do not transition (again, not true that evolution occurs adiabatically in the quantum sense). These seem to be at opposition/there seems to exist a contradiction since the derivation assumed that microstates do not transition?

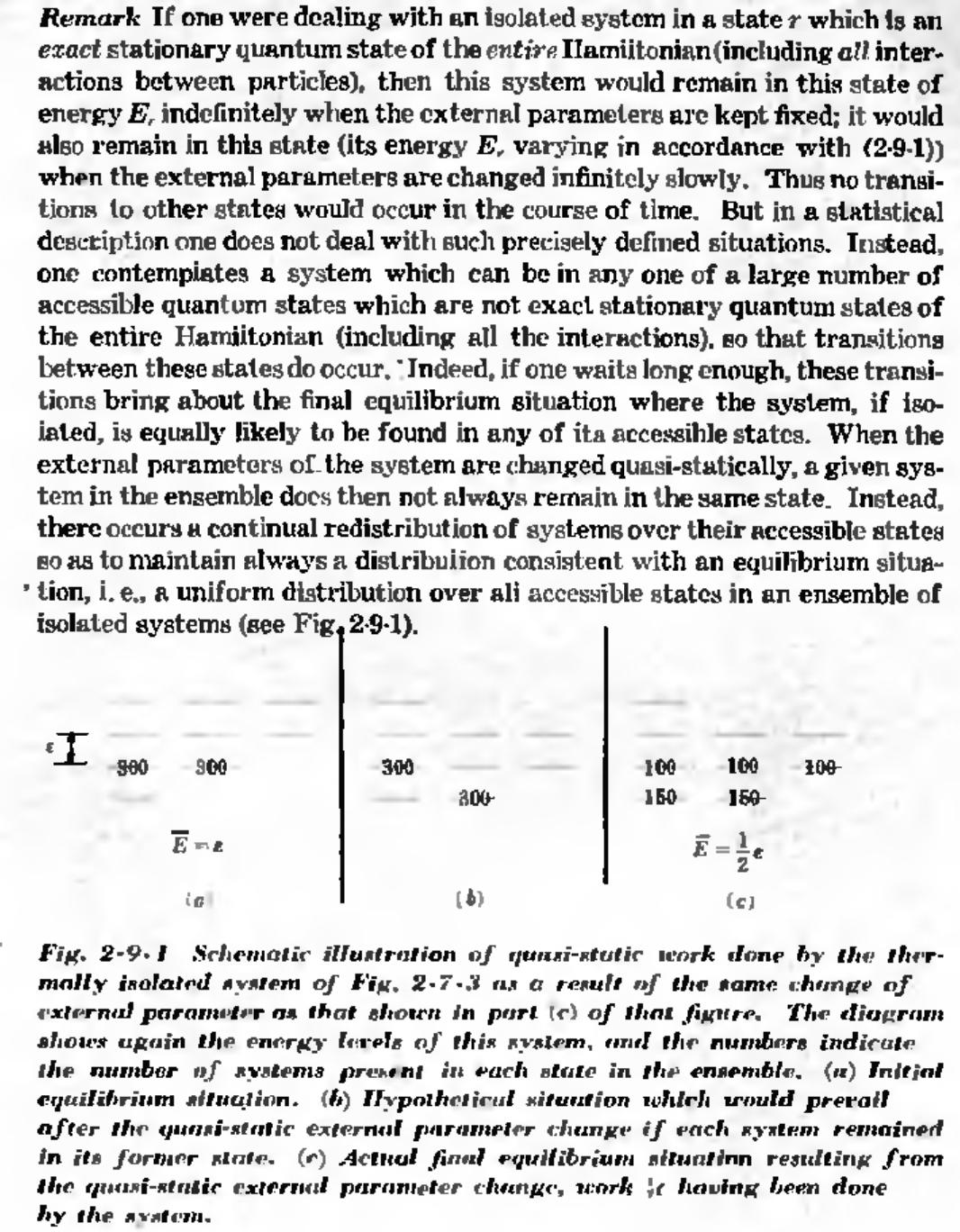
If my discussion above was not clear, I have attached the relevant passages below. The seeming contradiction lies in the sentence above (2.9.3) and then the closing (gray box) remark:

Answer 1

I'd just sidestep that derivation and refer you to Callen, and also Feynman on stat therm. Feynman's very cavalier throwaway criticism is that, if we really believe in infinite time limit, then the molecules of any gas will erode the chamber that confines them. All of physics thus should be seen as a compromise between things in a rather particular way---we say that something is in thermal equilibrium when the "fast" degrees of freedom has equilibrated, but the "excruciatingly slow" degrees of freedom is still taking its own sweet time. In that case, we have a long period of time where the macrostates seem to be completely stationary, and it is in the extraction of the behaviour of these things that we are trying to take things out.

Callen's point is also that any physical measurement we are taking are also in this limit. Anything that the human body can detect, cannot be happening so fast that we cannot even register the change. It must last long enough for us to perform the measurement.

Therefore, it is not any single microstate that we are making any measurement. It is a whole collection of microstates compatible with an imprecise spread in the macrostates suitable for a characterisation of a system, that we actually measure. Note that while there would be a lot of microstates involved, it may well take too long for this averaging of the true underlying process to reach all the way to the entire macrostate. As such, it is possible that the ensemble average might differ from actual measurements. That this difference kinda does not matter is an assumption, very related to the ergodic hypothesis.

Now, the quotation from Reif you have given is actually not as bad as you think it is. What the author actually wrote about is not that we are assuming a lack of transitions. For any system in the thermodynamic limit, i.e. large, there would always be minute transitions. What Reif is saying is that mathematically, if we happen to start with this gigantic system in an energy eigenstate, unlikely as it may be, then a process can be called quasistatic if during the implementation of the change, the change is so slow that under quantum theory, we can show that the system would stay within its eigenstate throughout the change. That is badly named the adiabatic limit (because really it should be quasistatic limit, adiabatic is just how they originally misunderstood it to be). However, that is a totally different physical requirement (namely, a definition of what it means for a change to be called implemented quasistatically), whereas you were confused about actually doing measurements. Since these are different things, it is just that you were confused about two separate things. There is no serious problems.

Answer 2

Considering statistical mechanics from the point of view of classical mechanics, an equilibrium macrostate as an ensemble, is time-invariant in the sense the probability of each microstate does not change with time. But obviously, the microstate (position / momentum) constantly changes.

Considering statistical mechanics from the point of view of quantum mechanics, the microstate can itself be time-invariant, meaning an eigenstate of the energy. The "adiabatic" theorem would imply that any slow process would keep the system in an eigenstate of the energy.

I think of a monoatomic ideal gas as a simple test case. I have no idea what an eigenstate of a many particle system that interact via elastic collisions may look like. Also it does not sound very realistic that we wait for equilibrium long enough for such a thing to happen. A possible conceptual simplification is to assume each individual molecule is in an eigenstate of its own Hamiltonian: three quantum numbers. See : Ideal gas. We can say a microstate is described by an eigenstate for each molecule.

Any time a collision happens, energy is exchanged between two molecules. Thus the microstate is constantly doing transitions. But the probability of each microstate is overall kept steady. I cannot say for sure it is what Reif means.

Answer 3

Statistical physics assumes existence of residual interactions, responsible for equilibration between microstates, and thus for establishing thermodynamic equilibrium. These interactions influence how the system relaxes to a microstate and how fast we reach the equilibrium, but they do not affect what the equilibrium looks like (more precisely, they can be neglected, like when we consider a non-interacting gas.)

Extending this to quasistatic processes, where the system at every point remains in thermodynamic equilibrium, we see that these residual interactions affect the speed at which the process can be carried out to remain quasistatic, as they ensure that the probabilities of microstates quickly relax to their equilibrium value.

The microstates do evolve in a process - which is the same as saying that they are not the same microstates (which is more revealing, since *evolution implies some degree of continuity.)

E.g., let as consider an expansion of an ideal gas: we split the whole volume $V$ into cells of size $v$ and each microstate corresponds to a different way of distributing $N$ molecules among $V/v$ cells. Now, if the volume changes to $V+dV$, we have a different number of cells and different set of microstates - some of these microstates may be the same as before, but some of them are new (or have vanished, if $dV<0$) and hence transitions between them must take place.