4
$\begingroup$

In establishing ADM or 3+1 decomposition, one starts with choosing a foliation $\Sigma_t$ where t is a scalar function and $\Sigma_t$ is demanded to be a spacelike slice, i.e. with time-like normal vector field.

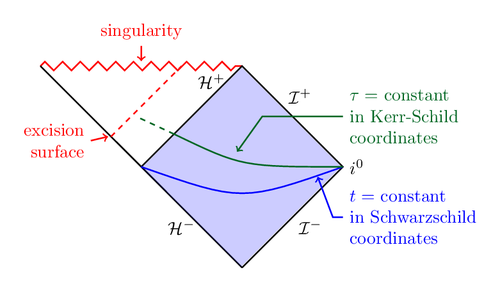
In the case of Schwarzschild black hole, the constant time slice (coordinate $t$ in Schwarzschild metric) seems (to me) a natural choice. But recalling that $t$-coordinate inside the Schwarzschild horizon turns into space-like while $r$-coordinate turns into time-like. I'm really puzzled in thinking about ADM decomposition together with black hole, especially inside the horizon.

One might say ADM decomposition is mostly concerned with isolating system and hence large distance, then shall one need to impose an inner boundary at the horizon? Appreciate any comments!

Improve this question
$\endgroup$

2 Answers 2

Reset to default
0
$\begingroup$

In general, one needs to use a set of horizon-penetrating coordinates. These can be illustrated using a Penrose diagram of a Schwarzschild black hole: Source: Jonah Miller, https://texample.net/tikz/examples/spacetime/

(If you do not know what a Penrose diagram is, you should start with covering the Schwarzschild space-time with Kruskal-Szerekes coordinates.)

The $t = const.$ slices go all the way to the horizon and then go through a branch cut at the horizon (a non-smooth change of meaning). On the other hand, coordinates such as the time coordinate in Kerr-Schild coordinates (also known as Eddington-Finkelstein in Schwarzschild space-time) stay smooth at the horizon, while still blowing up at the singularity. Interestingly, Kruskal-Szekeres "$T$" gives you a coordinate and foliation that is everywhere regular, but hits the singularity "all at once" at a late time. This sort of tells you why theorists say that the problem of BHs is a "late time" problem and why numerical relativity can really postpone dealing with the Schwarzschild singularity.

Without going into other technical details, variations of these coordinates (such as the isotropic coordinate) can be used to deal with 3+1 formalisms applied to black hole space-times.

Improve this answer
$\endgroup$
1
  • $\begingroup$ In the case of Schwarzschild spacetime, this might handle the problem. But what about the more important Kerr spacetime? In the ergosphere, the coordinate t also becomes spacelike (and the asymptotically timelike Killing vector also). The metric there is of signature (+,+,+,+), right? There's no way to deal with that with a 3 +1 formalism, I guess. Or am I wrong? $\endgroup$
    – Attila Janos Kovacs
    Commented Jun 8 at 21:10
-1
$\begingroup$

I feel like it should be improbable to decompose a black hole in these constraints. Given that that the space like hypersurfaces cannot circumvent the singularity in the black hole metric, it would not be possible to construct smooth foliations. Furthermore, the time parameterization would not be a 1-1 map through each constant time hypersurfaces due to the singular nature of space around the black hole.

These are my thoughts, however, would appreciate your consult on this. Thank you!

Improve this answer
$\endgroup$
1
  • $\begingroup$ Your answer could be improved with additional supporting information. Please edit to add further details, such as citations or documentation, so that others can confirm that your answer is correct. You can find more information on how to write good answers in the help center. $\endgroup$
    – Community Bot
    Commented May 10, 2023 at 11:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.