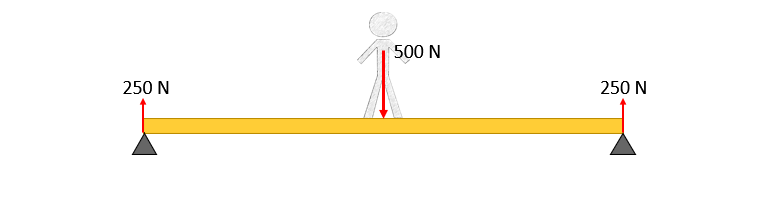
Consider the classic scenario of an equilibrium with a man standing at the centre of the plank, with the plank being held up by two trestles, say the man exerts a force of 500N at the point he is standing at, my book claims that the sum of the upward forces exterted by the trestles must be equal to 500N since the configuration is in an equilibrium, why is this the case? I would understand that the sum of forces in one direction equals the some of forces in the opposite direction about the same point, but here we are considering three different points the centre point and the two endpoints at which the trestles are located…
$\begingroup$
$\endgroup$
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
2
For a system of particles, a rigid body being a special case, the vector sum of the external forces equals the total mass times the acceleration of the center of mass (CM), regardless of the positions at which the forces are applied. See a basic physics textbook, such as one by Halliday and Resnick. Therefore, in equilibrium the vector sum of the external forces is zero.
(The system can have rotational motion determined by the sum of the external torques, even if the CM is stationary.)
answered Feb 13, 2023 at 14:09
-
$\begingroup$ Could you please give intuition for why that is the case? $\endgroup$ Commented Feb 13, 2023 at 14:19
-
$\begingroup$ This is surprising to most people studying basic physics. Internal forces do not matter, and the CM motion is the vector sum of the total external force. The intuition as to why requires understanding how forces on particles affect the CM and that is gained by looking at proofs in a physics textbook. Other useful generalization using the CM are: the change in angular momentum about the CM is equal to the total external torque, even if the CM is accelerating, and the total kinetic energy is that of the CM plus motion of the system with respect to the CM. The CM is special! $\endgroup$ Commented Feb 13, 2023 at 15:39
$\begingroup$
$\endgroup$
3
The plank is assumed to be rigid and the conditions for static equilibrium of the plank are that the net force on the plank is zero and the net torque on the plank is zero.
So it is not just the sizes of the forces which are important but where they act on the rigid body.
A way of analyzing the situation is as follows.
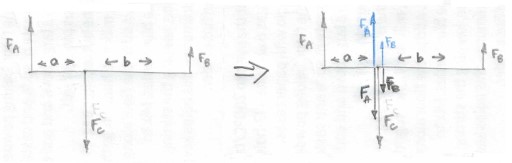
The diagram on the left shows the original configuration of forces.
Now add two forces $F_{\rm A}$ and $\color{blue} F_{\color {blue}{\rm A}}$ at the centre of mass $C$ noting that the resultant of these two forces is zero.
Add another two forces $F_{\rm B}$ and $\color{blue} F_{\color {blue}{\rm B}}$ at the centre of mass $C$ again noting that the resultant of these two forces is zero.
Thus at the centre of mass, $c$, you have $\color{blue} F_{\color {blue}{\rm A}}$ and $\color{blue} F_{\color {blue}{\rm B}}$ acing upwards and force $F_{\rm C}$ acting downwards.
Thus, all those forces are acting on the same point and if their sum is zero that is the first part of the static equilibrium conditions satisfied.
The two grey forces $F_{\rm A}$ which are equal in magnitude and opposite in direction are what is called a couple and produce a torque equal to $F_{\rm A}\,a$ in a clockwise direction and the two grey forces $F_{\rm B}$ which are equal in magnitude and opposite in direction produce a torque equal to $F_{\rm B}\,b$ in a counter-clockwise direction If the sum of those two torques is zero (think moments about the centre of mass) then the second of the two conditions for static equilibrium is satisfied.
-
$\begingroup$ Could you please give intuition for why that is the case? $\endgroup$ Commented Feb 13, 2023 at 14:18
-
$\begingroup$ Why can we assume the forces FA and FB remain unchanged at the centre of mass? $\endgroup$ Commented Feb 13, 2023 at 15:20
-
$\begingroup$ The forces have been chosen to equal in magnitude to the original forces. $\endgroup$– FarcherCommented Feb 13, 2023 at 19:03
$\begingroup$
$\endgroup$
2
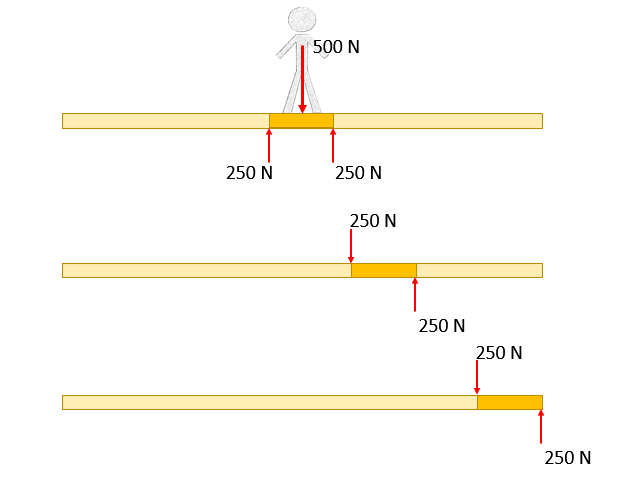
There is a concept that when a system is in static equilibrium, you can slice any part of the system off and the remaining part must be in equilibrium also.
As a result, the part of the plank where the fellow is standing and nearby must be in equilibrium. To do so there are internal forces of 250 N as seen below in the first line.
Then consider the part if the plank next to it, it too must have internal forces of 250N each to as an equal and opposite force from the middle part (Newton's 3rd law).
And so move all the way to the supports (third line above) and you will see that the plank needs 250N force from the supports to be in equilibrium.
As a simplification, you can consider the plank as a rigid body and sum of the forces acting on it to balance out
The balance equations are the net force and net moment (about an arbitrary point A, not shown) have to equal to zero.
$$ \boldsymbol{F}_{\rm net} = 0 \\ \boldsymbol{M}_{{\rm net},A} = 0 $$
answered Feb 13, 2023 at 19:23
-
$\begingroup$ Interesting answer much appreciated, why must the segment of the plank exert a force on the adjacent segment? $\endgroup$ Commented Feb 13, 2023 at 19:32
-
$\begingroup$ Newton's 3rd law. Each slice you make must have equal and opposite forces on both sides of the slice. $\endgroup$ Commented Feb 13, 2023 at 20:17
$\begingroup$
$\endgroup$
The center point is where the force is mainly taking place and the man has a downward force of 500N , the system is said to be in equilibrium means that the system is not moving anywhere , and is not breaking etc. Hence the net force must be 0 and due to that the contact force(upward force) equals 500N as said in the book
