Measurement of an Inertial frame according to two other inertial frames at the same position

Question

I have a question related with measurements in special relativity. I know that, if we want to compare times between different Inertial Frames, then we must have both clocks at the same exact location to get rid of any desynchronization. Now, if we have two inertial frames, one at rest and the other at a velocity close to $c$, and both of them happen to coincide at a certain point in space, will their measurements regarding another Inertial Frame (which isn't at the same spot, with a velocity also close to the speed of light) coincide at the time they are in the same position?

I would intuitively say that they won't because time dilation, but my fellow insists in that their measurements will be the same because they aren't desynchronized.

EDIT: I clarified the comparing times bit. Also, I will use an example to hopefully make my doubt clearer:

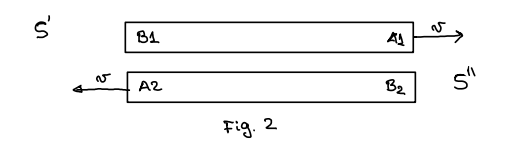
Imagine two bars that are about to coincide with each other, each one with a speed $v$, parallel, but with opposite directions, like so: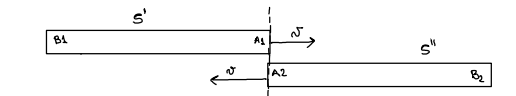

We will call the first bar $S'$, the second bar $S''$.

Now, in the next picture consider an observer at rest in A1 whose space and time origins coincide with $S'$ and $S''$. My doubt then is, in the second figure, where $S'$ and the observer are at the same place, will the observer and $S'$ measurements of $B_2$'s clock's time coincide?

Hope this made it clearer and thanks.

Answer 1

This can be solved in a straightforward (but not particularly simple) manner using the Lorentz transforms. Here there are 3 reference frames of interest, the reference frame of the drawings $S$, the reference frame of the bar moving to the right in the drawings $S'$, and the reference frame of the bar moving to the left in the drawings $S''$. The origin of all three frames is the event where $A1$ meets $A2$. The bars have length $L$ in $S$. For convenience I will use units where $c=1$.

First, I will write the worldlines of the labeled points in $S$. The worldlines represent the $(t,x,y,z)$ coordinates but since $y=z=0$ I will just drop the last two coordinates and report only $(t,x)$: $$A1=\left( t, v t \right)$$$$B1=\left( t, v t -L \right)$$$$A2=\left( t, -v t \right)$$$$B2=\left( t, -v t +L \right)$$ To calculate the worldlines in $S'$ we simply multiply the above by the Lorentz transform matrix $$\Lambda(v) = \left( \begin{array}{cc} \frac{1}{\sqrt{1-v^2}} & -\frac{v}{\sqrt{1-v^2}} \\ -\frac{v}{\sqrt{1-v^2}} & \frac{1}{\sqrt{1-v^2}} \\ \end{array} \right)$$ After doing that we wind up with the coordinates in $S'$, but expressed in terms of $t$, the time in $S$. We can solve to get an expression for $t$ in terms of $t'$, the time in $S'$, and then we can substitute back to get the coordinates in $S'$ expressed in terms of $t'$. Doing so gives us: $$A1'=\Lambda(v) A1 = \left(t',0 \right)$$$$B1'=\Lambda(v) B1 = \left(t',-L\gamma \right)$$$$A2'=\Lambda(v) A2 = \left(t',\frac{2 t' v \gamma^2}{1-2 \gamma^2} \right)$$$$B2'=\Lambda(v) B2 = \left(t',\frac{\gamma(L-2t' v \gamma)}{2\gamma^2-1} \right)$$ And working similarly for $S''$ we get:$$A1''=\Lambda(-v) A1 = \left(t'', \frac{2t''v \gamma^2}{2\gamma^2-1} \right)$$$$B1''=\Lambda(-v) B1 = \left(t'', \frac{\gamma(2t''v \gamma-L)}{2\gamma^2-1} \right)$$$$A2''=\Lambda(-v) A2 = \left(t'', 0\right)$$$$B2''=\Lambda(-v) B2 = \left(t'', L \gamma\right)$$ Now that we have all of the various worldlines correctly calculated in all of the frames, it is easy to answer questions about anything we like.

In particular, for your question we will solve for $t'$ when $A1'=B2'$ and $t''$ when $A1''=B2''$. When we do that we get $$t'=\frac{L}{2v\gamma}$$ and $$t''=\frac{L(2\gamma^2-1}{2v\gamma}$$ So the final conclusion is that no, the clocks do not match.