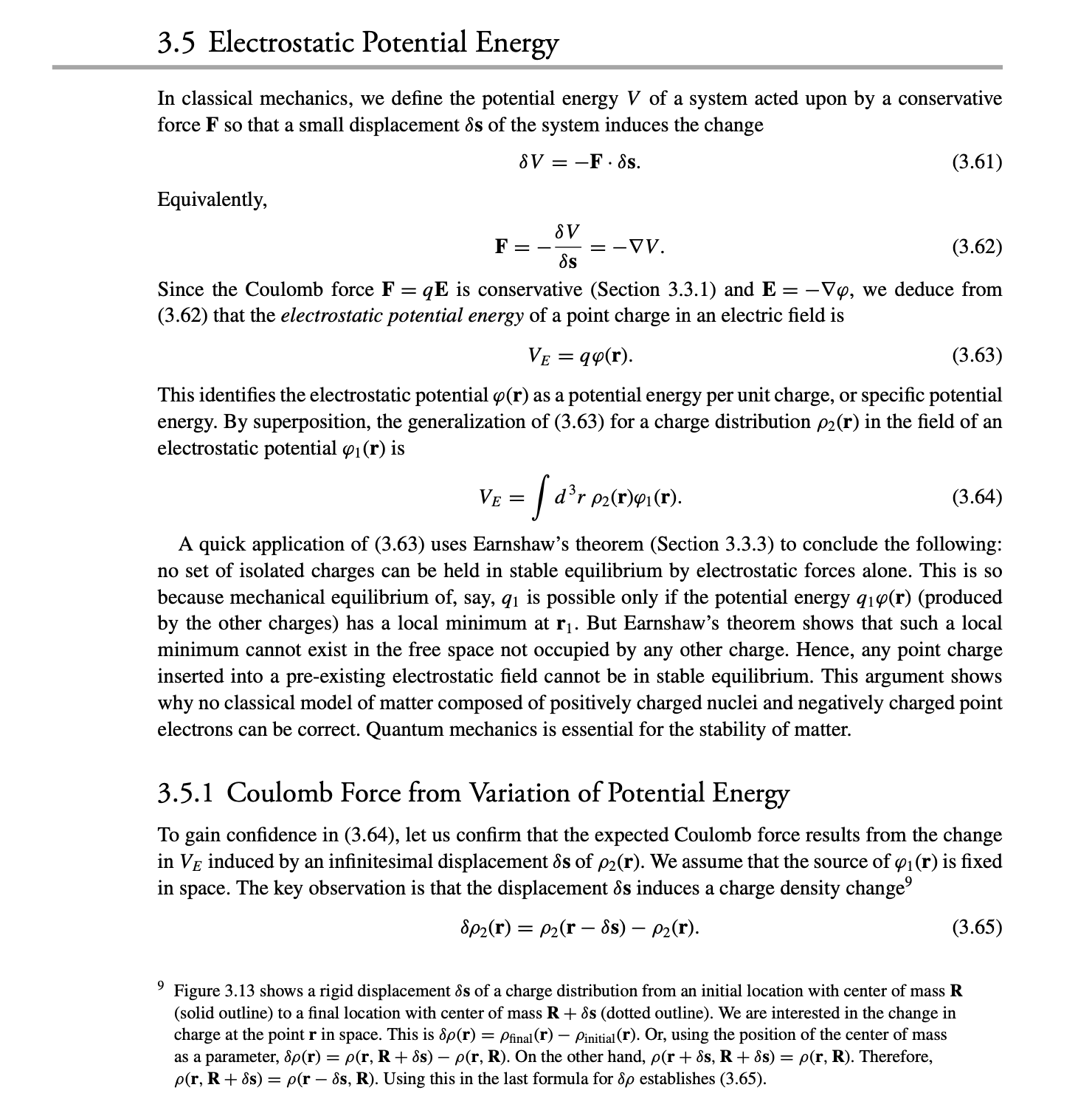
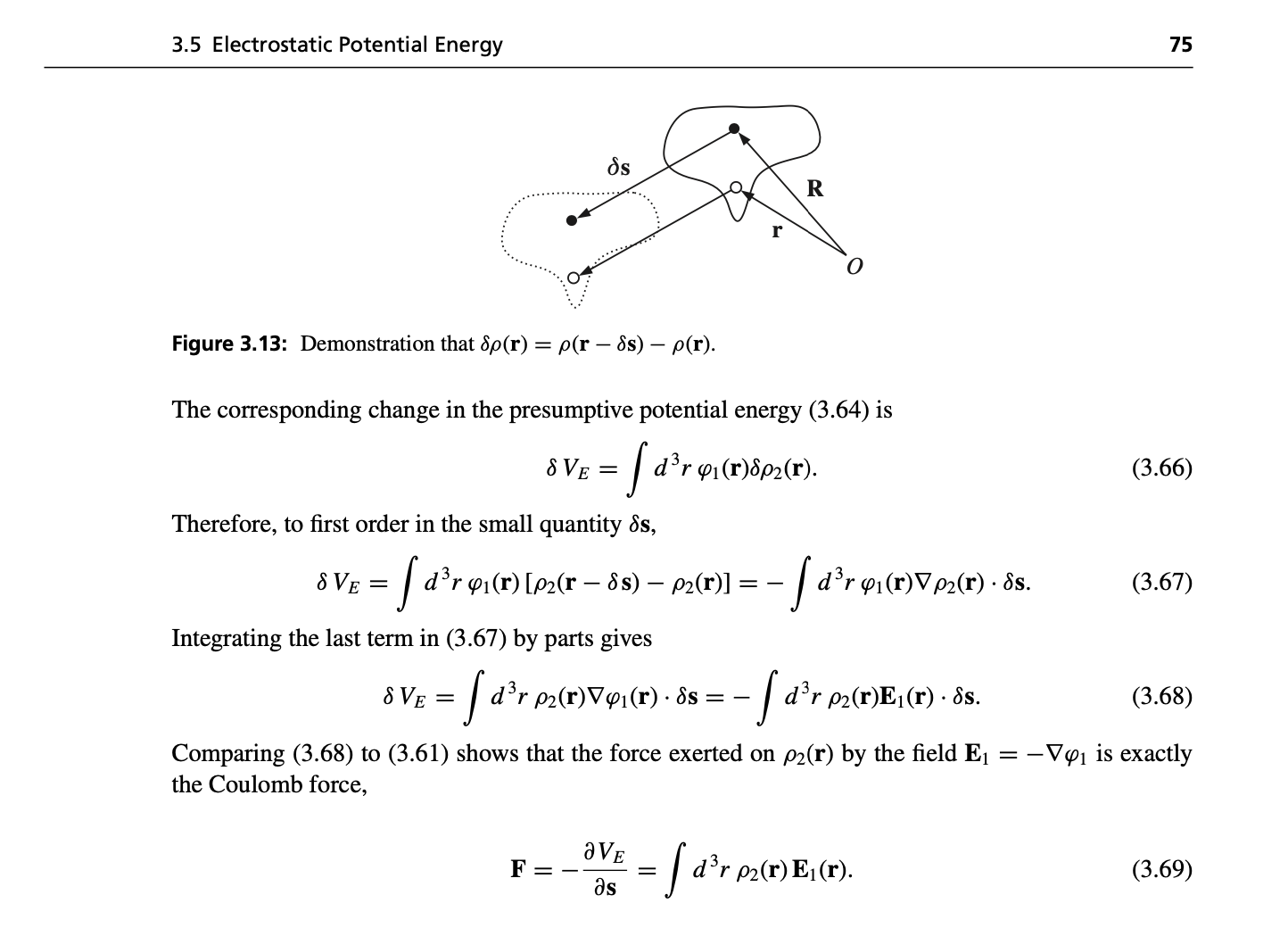
See the attached discussion from Zangwill's Modern Electrodynamics, and in particular footnote 9. The point of this question is to understand how to recover Coulomb’s force law from an assumed form for potential energy.
I have three questions about this calculation:
How general is the calculation? In particular, footnote 9 clearly dictates that we are computing a slight perturbation in the potential energy, $\delta V_E$, due to a rigid shift of the charge distribution $\rho_2$ (i.e. due to a shift in the centre of mass coordinate $\textbf{R}$). But one could conceive of more general motions of the distribution -- e.g. to a deformation of the distribution. Thus why should we expect our calculation of the Coulomb force $\textbf{F}$ in 3.69 (which is of course correct by way of other derivations) to be correct in the most general case?
I'm a bit confused with the logic that Zangwill uses in 3.69. First, I think from 3.68 on the RHS that we can easily conclude that minus the integral on the RHS of 3.68 is the gradient of the potential energy with respect to these centre of mass coordinates, since only the gradient obeys the equation (for the total differential of $V_E$) $\delta V_E = (\nabla_\textbf{R}V_E) \cdot\delta\textbf{s}$ for all possible perturbations of the centre of mass $\delta \textbf{s}$. Fair enough: so this establishes the RHS equality in 3.69, where I believe Zangwill is using the notation $ \nabla_\textbf{R}V_E \equiv \frac{\partial V_E}{\partial \textbf{S}}$. Is this correct? Further, to establish that these are each equal to the force $\textbf{F}$ on system 2, I suppose we appeal to some theorem from classical mechanics which says that the force on an extended system equals (minus) the gradient with respect to the centre of mass coordinate of the potential energy of the system -- is this true? I am not familiar enough with classical mechanics to know.
Finally, it should be possible to compute $ \nabla_\textbf{R}V_E$ given the proposed form 3.64 for $V_E$ and to recover the Coulomb force $\textbf{F}$ in 3.69. That is to say, thinking of $V_E$ as a function of the centre of mass coordinate $\textbf{R}$ for a fixed background potential $\varphi_1$, and of $\rho_2 = \rho_2(\textbf{r},\textbf{R})$ as a function of the centre of mass coordinate $\textbf{R}$ as well, we find $$-\nabla_\textbf{R}V_E=-\nabla_\textbf{R}\int d^3r \ \rho_2(\textbf{r},\textbf{R})\varphi_1(\textbf{r})=-\int d^3r \ \nabla_\textbf{R}\rho_2(\textbf{r},\textbf{R})\varphi_1(\textbf{r}) $$ but I struggle to go any further than this. I think I've got to somehow express $\textbf{r}$ in terms of $\textbf{R}$ so that I can use integration by parts to transfer the derivative over to $\varphi_1$, but I can't quite see how. Perhaps I was wrong to write $\rho_2 = \rho_2(\textbf{r},\textbf{R})$. I suppose I can perhaps observe that for every $\textbf{r}$, $\textbf{r} = \textbf{R}+\textbf{s}$ for some $\textbf{s}$, so that $$-\nabla_\textbf{R}V_E=-\nabla_\textbf{R}\int d^3r \ \rho_2(\textbf{r})\varphi_1(\textbf{r})=-\int d^3s \ \nabla_\textbf{R}\rho_2(\textbf{R}+\textbf{s})\varphi_1(\textbf{R}+\textbf{s}) = -\int d^3s \ (\nabla_\textbf{R}\rho_2(\textbf{R}+\textbf{s}))\varphi_1(\textbf{R}+\textbf{s}) + \int d^3s \ \rho_2(\textbf{R}+\textbf{s})\textbf{E}_1(\textbf{R}+\textbf{s})$$ but the last expression is not correct (does not equal $\textbf{F}$) since the first term does not vanish. Where am I going wrong?