0
$\begingroup$

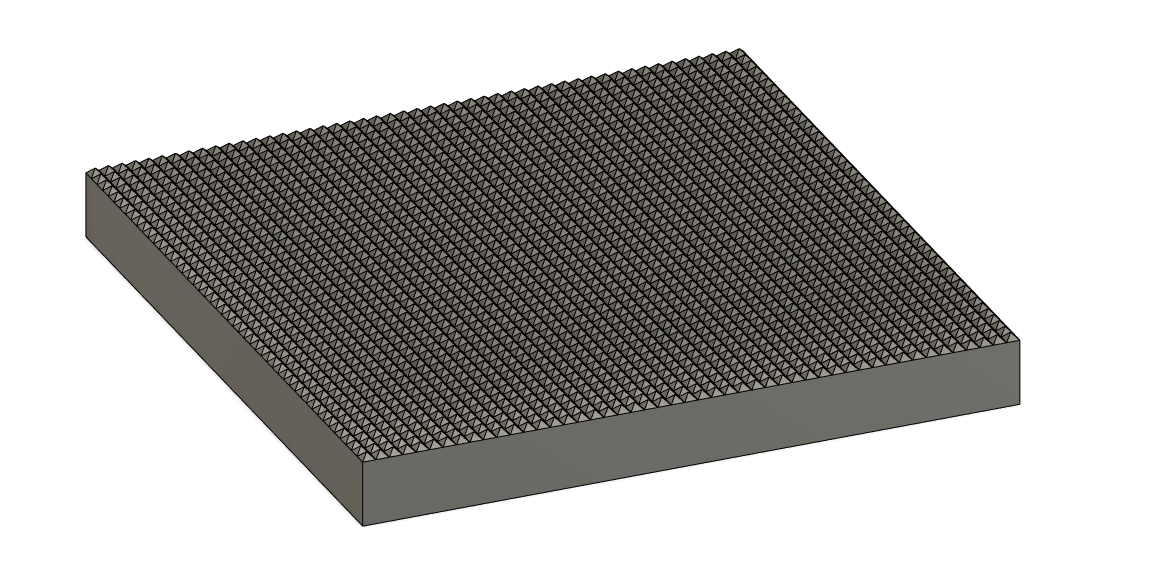
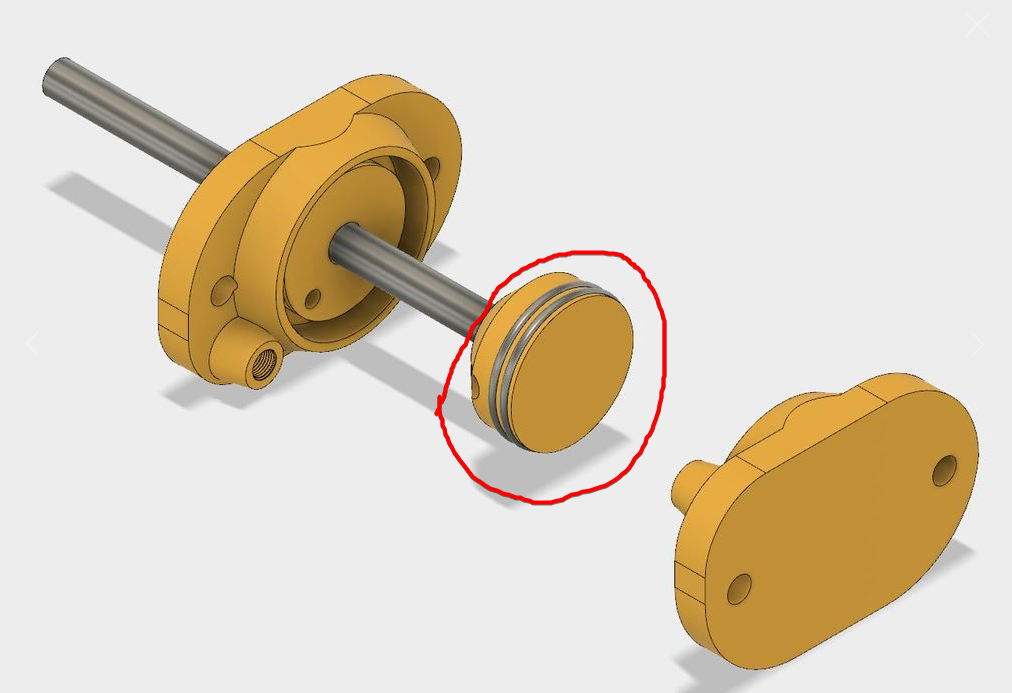
Would it be practical to texture the surface of a piston in a pneumatic cylinder to increase the pressure area and therefore the thrust force? enter image description here

F = P * A

For example, according to the soundproofing method, we can cover the surface with pyramids.

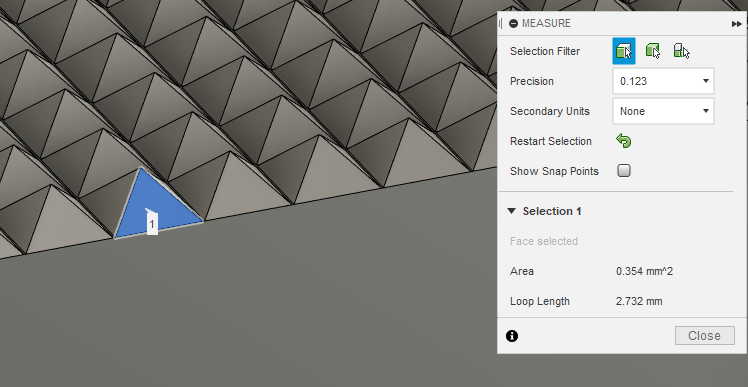
enter image description here

enter image description here

In this case, the smooth surface area of 50*50 mm will be 2500 mm^2, with the addition of 1 mm pyramids it will become 3540 mm^2 (0.354 * 4 * (50 * 50)).

This can increase the final strength by almost one and a half times, but would it be worth doing in terms of durability and strength? Is pyramid design the most efficient or should other geometric designs be used?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Welcome Rooter to Physics Stack Exchange. What makes you think that texturing the surface of the piston will increase the thrust? (Hint: Force components perpendicular to the direction of motion don't contribute to the thrust.) $\endgroup$
    – David Bailey
    Commented Dec 29, 2022 at 16:13
  • $\begingroup$ I just looked at the formula for force versus pressure and thought that artificially increasing the area will give an increase in thrust. But that doesn't seem to work) $\endgroup$
    – Rooter
    Commented Dec 29, 2022 at 16:20

2 Answers 2

Reset to default
0
$\begingroup$

You're thinking in terms of the formula $F = pA$. The problem with using it here is that force isn't a scalar, it's a vector, meaning it has direction. In your pyramidal design, the air will not push on the piston in the direction it can move, but it will push in the direction perpendicular to the surface of the pyramids, which is oblique and not the same all over the surface of the piston. A part of the force will cancel itself out, and it turns out that this part is exactly the part you thought you gained.

Improve this answer
$\endgroup$
0
$\begingroup$

No, it will not. The pressure exerted on the fluid by the piston depends on the component of the surface area that is normal to the direction of the piston's motion. for a deeply textured 3-dimensional piston surface this winds up being equal to the "ordinary" area of the piston top.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.