Can a photon have a radius?

Question

I have seen someone answering this question by saying that a photon can has a radius as big as its wavelength, is that true? If it is true Does this then mean a photon of the radio radiation, for example, can have a radius up to a tenth of kilometers?!

Answer 1

There is some truth in the statement but it's a lot more complicated than the photon having a radius.

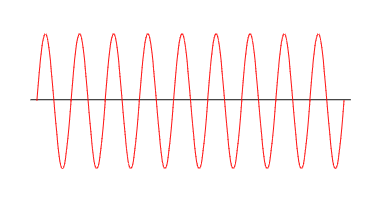
We think of a light beam as being a very long wave looking something like this:

where in principle the wave extends out to infinity in both directions. When we have a single photon this means the photon is spread out over a very large distance - we say the photon is delocalised over a large distance.

But it's possible to localise the photon into a smaller region to get a wave packet instead of an infinite wave. This looks something like:

In this case we can think of the photon having a size of about the length of the wave packet, which in this example is about five wavelengths.

But there's a wrinkle with this. It turns out that to create a wave packet we have to create a mixture of different photons with different wavelengths. So the wave packet in the diagram above is actually a mix of different photons. Decreasing the size of the photon has turned it into a mixture of different photons.

The smaller we make the wave packet the more mixed up the photons in the packet become, and at some point we can no longer usefully describe it as a single photon any more. So there is a lower limit to the size we can make the photon as below this limit it isn't even approximately a single photon any more.

This lower limit is rather vaguely defined but it is going to be around the wavelength of the photon. It is in this sense that we could say the minimum radius of a photon is about its wavelength. However I must emphasise that a photon is not like a little ball of light and doesn't really have a radius, so you need to treat statements about the size of a photon with some care.

If you're interested to know more about what a photon is then see my answer to Do photons truly exist in a physical sense or are they just a useful concept like $i = \sqrt{-1}$? Also see the answers to What is Size of Photon? where the concept of a photon "size" is examined.

Answer 2

I consider photons to be quantized excitations of electromagnetic field 3d spatial modes. The decomposition of the (classical or quantum) electron into spatial modes is not always unique but sometimes boundary conditions lead to a canonical decomposition that we can use to define the "shape" of a photon. But this is only in some special circumstances. I would say, in general, since the field decomposition is not unique, we can not generally assign size to photons.

In, for example, an optical cavity, the modes can be decomposed into a few different families of modes such as Hermite Gaussian, Or Laguerre Gaussian modes. The TEM00 modes are Gaussian modes that look like laser beams. These modes have a beam waist that varies throughout the length of the cavity but for some cavity geometries it is relatively constant. So the spatial mode certainly has at worst a loosely defined radius.

Classically we can pump energy into this cavity. Quantum mechanically we can do the same but the excitations are quantized (though we can pump excitations of different superpositions of numbers of excitations so that the expected value of energy in the cavity is continuous). In any case. If we pump one excitation into the cavity then I say there is one photon in the cavity and it has a radius given by the beam waist.

However, electromagnetic energy does not only exists in linear cavities and waveguides with well defined confined spatial modes. It also lives in free space. Here the mode decomposition is not unique. We can decompose the EM field into plane waves (infinite radius) or Hmite Gaussian beams (well defined radius but varies along the z-coordinate) or other mode families (e.g. spherical vector multipole solutions). So in this case I would say we can not assign a unique radius to a photon.

That said, once we pick a mode decomposition, we can decompose the quantum spatial field into these modes which each have "shapes" and we can talk about how many photons are in each mode. For some shapes (like beams) it makes sense to talk about the radius of the shape, but for some shapes (like spherical dipoles or something) it doesn't make sense to talk about the radius.

Note that the distribution of photons and shapes will vary depending on the mode decomposition even for the same quantum electromagnetic field. That is if our field is a laser beam that we decompose into Gaussian modes then we might find this decomposition yields one photon (per second) in a mode with a fixed Gaussian radius but if we decompose the mode into plane waves we will find it is a superposition of modes with sub-unity occupation all with infinite radius.