Wick contraction in quantum field theory

Question

I am reading Anthony Zee's "Quantum Field Theory in a Nutshell" (1st edition). On page 47, when evaluating the 4-point Green's function $G_{ijkl}^{(4)}$ to order $\lambda$ using Wick contraction, he writes: $$\begin{align}G_{ijkl}^{(4)}=&\int_{-\infty}^{+\infty}\left(\prod_m dq_m\right)e^{-\frac{1}{2}q\cdot A\cdot q}q_iq_jq_kq_l\left[1-\frac{\lambda}{4!}\sum_nq_n^4+O(\lambda^2)\right]/Z(0,0)\cr =&(A^{-1})_{ij}(A^{-1})_{kl}+(A^{-1})_{ik}(A^{-1})_{jl}+(A^{-1})_{il}(A^{-1})_{jk}\cr &-\lambda\sum_n(A^{-1})_{in}(A^{-1})_{jn}(A^{-1})_{kn}(A^{-1})_{ln}+O(\lambda^2).\end{align}\tag{I.7.10}$$ I don't understand why, when using Wick contraction, in the order $\lambda$ term, there is no term like $$\sum_n(A^{-1})_{nn}(A^{-1})_{nn}(A^{-1})_{ij}(A^{-1})_{kl}\tag{1}$$ since I can "connect" $n$ to $n$, $n$ to $n$, $i$ to $j$ and $k$ to $l$. Can someone explain it?

Answer 1

That diagram would be a vacuum bubble, which is cancelled by the normalization factor $1/Z(0,0)$. It corresponds to a renormalization of the vacuum state in the interacting theory relative to the free theory.

This is covered in Peskin and Schroeder, I believe in Chapter 4. I don't remember offhand if Zee discusses this or not.

Answer 2

Yes, OP is right. OP's term (1) [which corresponds to Fig. I.7.1c] together with e.g.

$$\sum_n(A^{-1})_{in}(A^{-1})_{nn}(A^{-1})_{nj}(A^{-1})_{kl} \tag{2}$$

[which corresponds to Fig. I.7.1b] are in principle also there. [This is mentioned 2 paragraphs below eq. (I.7.10) in the 2nd edition.] Such terms correspond to diagrams with self-loops.

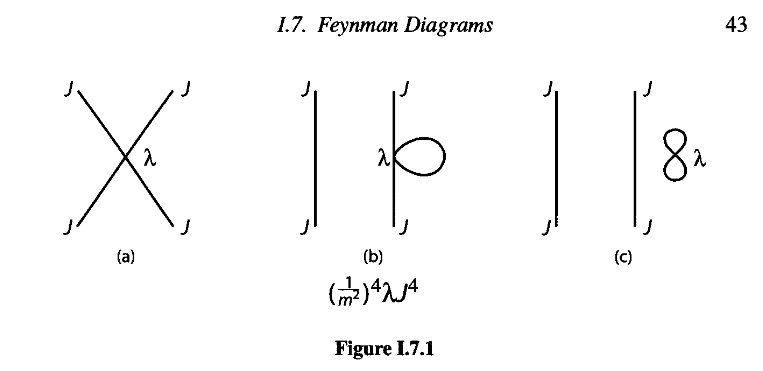
$\uparrow$ Fig. I.7.1 (Source: Ref. 1.)

1. The simplest fix to remove self-loops (in the operator formalism) is to normal-order the interaction term $$S_{\rm int}~=~\frac{\lambda}{4!} \sum_n :q_n^4: $$ see e.g. Ref. 2.

2. However Ref. 1 is instead using the path integral formalism in 0+0D. It turns out it is enough to only study 1PI correlation functions, such as e.g., the self-energy, as all other diagrams can be built from these. However 1PI correlation functions still contain diagrams with self-loops. These self-loops are removed via renormalization/counterterms.

References:

1. A. Zee, QFT in a nutshell, 2010; section I.7.

2. C. Itzykson & J.B. Zuber, QFT, 1985; p.271.