2
$\begingroup$

I was reading about a Bell’s inequality experiment from the paper https://arxiv.org/abs/quant-ph/0205171.

In the paper it gives a set of recorded values:

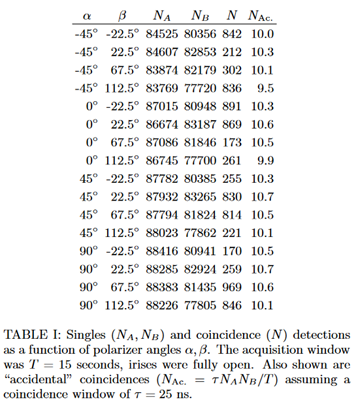
Blockquote

I understand how they got the value for S, but I am having trouble understanding how to use this formula to find the error:

Blockquote

The final answer should be: $S = 2.307 ± 0.035$

What should $N_i(\partial S/\partial N_i)^2$ be?

Improve this question
$\endgroup$
2
  • $\begingroup$ Eqs 21 and 25 tell you how to compute $S$ in terms of $N_i$, and the $N_i$ are tabulated in the second-to-last column of Table I. To get $\partial S/\partial N_i$, you just do a partial derivative of the expression for $S$. As the paper says, there will be a lot of terms. Do you have a specific step you are stuck on? $\endgroup$
    – Andrew
    Commented Nov 10, 2022 at 0:40
  • 1
    $\begingroup$ @kcoding Your last edit accidentally erased most of your question. $\endgroup$
    – David Bailey
    Commented Nov 10, 2022 at 15:40

1 Answer 1

Reset to default
1
$\begingroup$

Rewriting Equation 21 in different notation,

\begin{equation} S = E(N_1,N_2,N_3,N_4) - E(N_5, N_6, N_7, N_8) + E(N_9, N_{10}, N_{11}, N_{12}) - E(N_{13}, N_{14}, N_{15}, N_{16}) \end{equation} where (rewriting Equation 25 in different notation) \begin{equation} E(N_i, N_j, N_k, N_l) = \frac{N_i + N_j - N_k - N_l}{N_i + N_j + N_k + N_l} \end{equation} So for example \begin{equation} \frac{\partial S}{\partial N_1} = \frac{1}{N_1 + N_2 + N_3 + N_4} - \frac{N_1+N_2-N_3-N_4}{\left(N_1 + N_2 + N_3 + N_4\right)^2} \end{equation}

WARNING The above is just meant to show you how the partial derivative would work. To actually figure out what entries in Table I correspond to $N_1, N_2$, etc, you need to be careful about whether the pairs of angles should be perpendicular or not, or whether the angles $a, a', b, b'$ are being used, following the original notation in Eqs 21 and 25 carefully.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you. Is the $N_1,N_2,N_3,N_4$ in the final equation you wrote the uncertainity in each values of $N_1,N_2,N_3,N_4$ or are they just the values $\endgroup$
    – Jack Jack
    Commented Nov 10, 2022 at 12:38
  • $\begingroup$ @kcoding Just the values. As an aside, in the last equation in your question, you see that the uncertainty is related to the value by $\sigma_{N_i}^2=N_i$, which follows from Poisson statistics. $\endgroup$
    – Andrew
    Commented Nov 10, 2022 at 13:12
  • $\begingroup$ wolframalpha.com/… $\endgroup$
    – Andrew
    Commented Nov 10, 2022 at 13:38

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.