Understanding page 141 of Blundell’s Concepts in thermal physics

Question

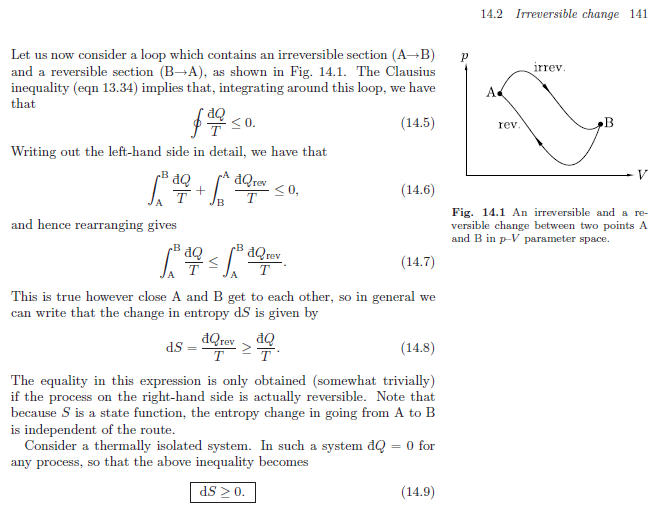
On this page (in the second edition), there is a figure containing two states A and B of a system:

There are two paths between A and B: one is an irreversible change, and the other is a reversible change. However, on the same page it says that entropy stays the same for a reversible change, and that entropy increases for an irreversible change. The first seems to imply that the entropy of A and B are the same; and the latter seems to imply that the entropy of B is larger than the entropy of A. This is a contradiction.

Am I missing something, or is this a mistake in the book?

Answer 1

on the same page it says that entropy stays the same for a reversible change,

yes, it says that

and that entropy increases for an irreversible change

yes, it says that

and the latter seems to imply that the entropy of B is larger than the entropy of A.

No, this does not follow from what the authors say.

They say that the heat along the reversible path and the the heat along the irreversible path satisfy the Clausius equation: $$\int_A^B \frac{dQ}{T} \leq \int_A^B \frac{dQ_\text{rev}}{T} \tag{14.7}$$ It then says that since $dS = dQ_\text{rev}/{T}$ we must have $$dS = \frac{dQ_\text{rev}}{T} \geq \frac{dQ}{T} \tag{14.8}$$ It follows that for an adiabatic process ($dQ=0$) we must have $$dS \geq 0 \tag{14.9}$$ which is a statement of the second law.

The result does not imply that $\Delta S_{A,B}$ along the irreversible path is larger than $\Delta S_{AB}$ along the reversible path, if by $\Delta S$ we are referring to the entropy change of the system. The statement would be correct if we are referring to the entropy change of the universe.

Answer 2

They might be referring to an adiabatic change, in which case, for a reversible path, the entropy change is zero. If B is the final state for an adiabatic reversible change, there is no adiabatic irreversible path starting at A for which B can be the final state. However, if the irreversible path is not adiabatic, the entropy of B can be the same as that for the reversible path. In the latter case, the overall entropy change of the system is the result of both entropy exchange with the surroundings plus entropy generated within the system.

In general, for a non-adiabatic reversible change of thermodynamic state, the change in entropy of the system does not have to be zero. And, for all changes between the same two thermodynamic equilibrium states (A tp B), whether reversible or irreversible, the change in entropy is the same.