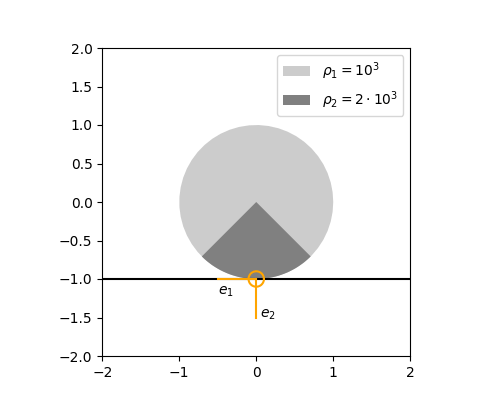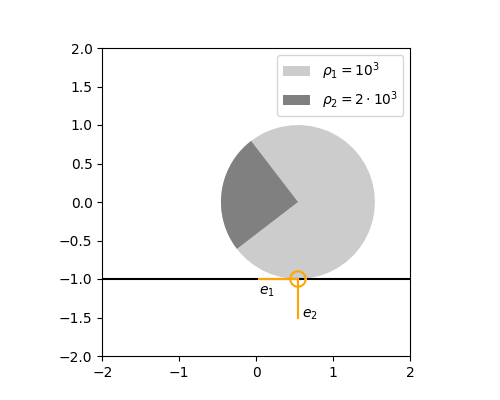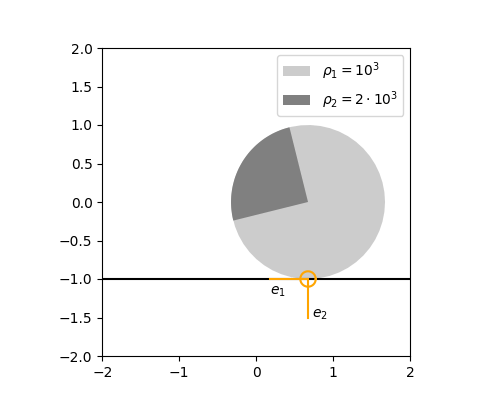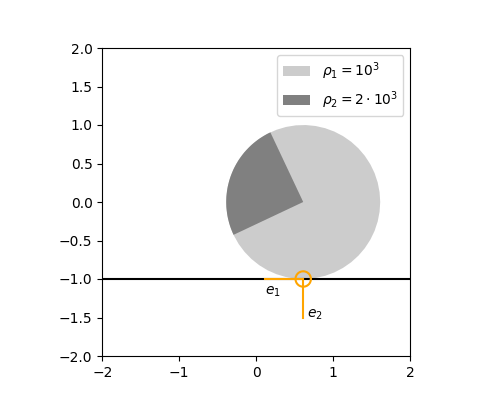Definition of time derivative of angular momentum (Wittenburg, "Dynamics of Multibody Systems")

Question

In Wittenburg, "Dynamics of Multibody Systems", 2e, 2008, p.45, (3.33), the definition of time derivative of angular momentum with respect to a reference coordinate system, in its most general form, is given as

$\dot{\mathbf{L}}^0=m[\mathbf{r}_A \times \ddot{\mathbf{r}}_C + \dot{\mathbf{r}}_A \times (\dot{\mathbf{r}_A + \mathbf{\omega \times \mathbf{\rho_C}}}) + (\mathbf{\omega} \times \mathbf{\rho_C}) \times \dot{\mathbf{r}}_A + \mathbf{\rho_C} \times \ddot{\mathbf{r}}_A] + J^A \dot{\mathbf{\omega}} + \mathbf{\omega} \times J^A \mathbf{\omega},$

based on the definition of angular momentum (3.15):

$ \mathbf{L}^0 = \mathbf{r}_A \times (\dot{\mathbf{r}}_A + \mathbf{\omega} \times \mathbf{\rho}_C)m + \mathbf{\rho}_C \times \dot{\mathbf{r}}_Am + J^A \mathbf{\omega}, $

where $\mathbf{r}_A$ is an arbitrary body reference point, $\mathbf{\omega}$ is a rotation vector, $\mathbf{\rho_C}$ denotes the center of mass with respect to $A$, $m$ is the body's mass, and $J^A$ is the inertia tensor with respect to $A$.

My question:

Applying

$ d_t \mathbf{p}^{(1)} = \mathbf{\omega} \times \mathbf{p}^{(2)} + d_t \mathbf{p}^{(2)}, $

where the superscript indicates the chosen basis, $\mathbf{p}$ is an arbitrary vector, and $d_t$ is the absolute time derivative, shouldn't the absolute time derivative of the angular momentum rather be

$ \begin{eqnarray} \dot{\mathbf{L}}^0 & = & \mathbf{\omega} \times (\mathbf{r}_A \times (\dot{\mathbf{r}}_A + \mathbf{\omega} \times \mathbf{\rho}_C)m + \mathbf{\rho}_C \times \dot{\mathbf{r}}_Am + J^A \mathbf{\omega})^{(2)} + d_t (\mathbf{r}_A \times (\dot{\mathbf{r}}_A + \mathbf{\omega} \times \mathbf{\rho}_C)m + \mathbf{\rho}_C \times \dot{\mathbf{r}}_Am + J^A \mathbf{\omega})^{(2)}, \\ & = & (\omega \times \mathbf{r}_A \times \dot{\mathbf{r}}_C + \omega \times \mathbf{\rho}_C \times \dot{\mathbf{r}}_A)^{(2)}m + \omega \times J \omega \\ && + (\mathbf{r}_A \times \ddot{\mathbf{r}}_C + \mathbf{\rho}_C \times \ddot{\mathbf{r}}_A)^{(2)}m + J^A \dot{\omega} \end{eqnarray} $

where the base corresponding to $(2)$ is chosen to be a body-fixed coordinaten system? (Note that $\dot{\mathbf{r}}_A + \mathbf{\omega} \times \mathbf{\rho}_C = \dot{\mathbf{r}}_C$)

Explicitly, what about the term

$ (\omega \times \mathbf{r}_A \times \dot{\mathbf{r}}_C + \omega \times \mathbf{\rho}_C \times \dot{\mathbf{r}}_A)^{(2)}m $

that seems to be missing in the above given definition of the timely derivative of the angular momentum?

---

To get a grip on the problem, I worked on problem 3.5 on page 46 of the same textbook:

Problem 3.5. In Fig. 3.4 an inhomogeneous circular cylinder of radius $R$, mass $m$ and moment of inertia $J^C$ about an axis through the center of mass $C$ is shown. The center of mass is located at the radius $b$. The cylinder is rolling without slipping on a horizontal plane. Formulate the equation of motion for the angular coordinate $\phi$ by using as reference point $A$ in (3.35) (i) the center of mass $C$, (ii) the geometric center $M$ and (iii) the point of contact with the plane.

The referenced figure 3.4 is this one:

For sakes of completeness, equation (3.35) is

$ m \mathbf{\rho}_C \times \ddot{\mathbf{r}}_A + J^A \dot{\mathbf{\omega}} + \mathbf{\omega} \times J^A \mathbf{\omega} = \mathbf{M}^A, $

but I did not use it. Instead I inserted (3.33) into Euler's theorem

$ \dot{\mathbf{L}}^0 = \mathbf{r}_A \times \mathbf{F}, $

and ended up with the differential equation

$ m(\mathbf{r}_C \times \ddot{\mathbf{r}}_C) + J^C \ddot{\phi} + \dot{\phi} \times (J^C \dot{\phi}) = \mathbf{r}_C \times (-m \mathbf{g}) $

describing the cylinder's motion if the reference point $A$ is chosen to be the center of mass $C$ which corresponds to case (i) of the exercise.

I integrated the differential equation for an example of an inhomogeneous cylinder, where a quarter of the cross-section has homogeneous density $\rho_2$, and the rest has $\rho_1$.

A visualization is shown here for $\phi_0 = 0, \dot{\phi}_0 = 10$ which is below the "break-out" angular velocity. Exceeding "break-out" angular velocity makes the cylinder travel along the floor, instead of oscillating about an equilibrium state.

This result shows that (3.33) yields plausible results, but I still don't know why the extra term mentioned above cancels out.

Answer 1

I received an answer from Prof Wittenburg which I'll put into my own perspective so, hopefully, I'm well aligned with his reply.

Let's start out with the definition of the time derivative of a physical quantity in a rotating reference frame. I found this post very helpful, and will basically copy it here, adapting notation. The quantity will be denoted $x$ in the inertial coordinate system and $x'$ in the rotating system, $R$ is the time dependent rotation matrix. I'm using d to denote the total time derivative operator.

$ \begin{eqnarray} x & = & Rx'\\ \text{d}x & = & \text{d}(Rx') \\ & = & \text{d}(R)x' + R\text{d}(x') \\ & = & (R\tilde{\omega})x' + R\text{d}(x') \\ & = & R (\omega \times x') + R\text{d}(x') \tag{*} \end{eqnarray} $

According to Prof Wittenburg, equation (*) is only used for quantities whose coordinates are known in the rotating reference frame. These are the center of mass with respect to the body-fixed point $A$, denoted $\rho_C$, and the quantity $J^A \omega$. Deriving $\rho_C$ over time in a rotating reference frame, as in (*):

$$ \begin{eqnarray} \text{d}\rho_C & = & R (\omega \times \rho_C') + R\text{d}(\rho_C') \\ & \stackrel{(a)}{=} & R (\omega \times \rho_C') \\ & \stackrel{(b)}{=} & (R \omega) \times (R \rho_C') \\ & \stackrel{(c)}{=} & \omega \times \rho_C, \label{1} \tag{1} \end{eqnarray} $$

where we're using the facts that $(a)$ the location of the center of mass does not change over time in the rotating system, $(b)$ the cross product is invariant under proper rotations about the axis defined by a × b, and $(c)$ that the rotational axis is the same in the inertial system and the rotational system $\omega = \omega'$.

Now deriving $J^A \omega$ over time in a rotating reference system yields

$$ \begin{eqnarray} \text{d}(J^A \omega) & = & R (\omega \times (J^A \omega)') + R\text{d}(J^A \omega)' \\ & = & (R \omega) \times (R(J^A \omega)')) + R(\text{d}(J^A)' \omega) + (J^A)' \text{d}\omega) \\ & = & \omega \times (J^A \omega) + R (J^A)' \text{d}\omega \\ & = & \omega \times (J^A \omega) + J^A \text{d}\omega. \label{2} \tag{2} \end{eqnarray} $$

Yet another identity that helps to simplify the final expression is the position of the center of mass in the inertial system, denoted $\mathbf{r}_C$ which is, by definition,

$$ \mathbf{r}_C = \mathbf{r}_A + \rho_C, $$

and its total time derivative, using \eqref{1},

$$ \begin{eqnarray} \text{d}\mathbf{r}_C & = & \text{d}(\mathbf{r}_A + \rho_C) \\ & = & \dot{\mathbf{r}}_A + \dot{\rho}_C \\ & = & \dot{\mathbf{r}}_A + \omega \times \rho_C \label{3} \tag{3}. \end{eqnarray} $$

Eventually, we're doing a straight-forward total time derivative of (3.15) by applying the product rule repeatedly:

\begin{eqnarray} \text{d}\mathbf{L}^{(0)} & = & \underbrace{\text{d}(\mathbf{r}_A \times (\dot{\mathbf{r}}_A + \mathbf{\omega} \times \mathbf{\rho}_C))}_{\text{expression A}}m + \underbrace{\text{d}(\mathbf{\rho}_C \times \dot{\mathbf{r}}_A)}_{\text{expression B}}m + \underbrace{\text{d}(J^A \mathbf{\omega})}_{\text{expression C}}, \end{eqnarray}

where expression A is

\begin{eqnarray} \text{d}(\mathbf{r}_A \times (\dot{\mathbf{r}}_A + \mathbf{\omega} \times \mathbf{\rho}_C)) & \stackrel{\eqref{3}}{=} & \dot{\mathbf{r}}_A \times (\dot{\mathbf{r}}_A + \mathbf{\omega} \times \mathbf{\rho}_C) + \mathbf{r}_A \times \text{d}(\dot{\mathbf{r}}_C) \\ & = & \dot{\mathbf{r}}_A \times \mathbf{\omega} \times \mathbf{\rho}_C + \mathbf{r}_A \times \ddot{\mathbf{r}}_C, \end{eqnarray}

expression B is

\begin{eqnarray} \text{d}(\mathbf{\rho}_C \times \dot{\mathbf{r}}_A) & = & \dot{\mathbf{\rho}}_C \times \dot{\mathbf{r}}_A + \mathbf{\rho}_C \times \ddot{\mathbf{r}}_A \\ & = & \omega \times \mathbf{\rho}_C \times \dot{\mathbf{r}}_A + \mathbf{\rho}_C \times \ddot{\mathbf{r}}_A, \end{eqnarray}

and expression C is (see \eqref{2})

$$ \text{d}(J^A \omega) = \omega \times (J^A \omega) + J^A \text{d}\omega. $$

$(A + B)m + C$ yields the right-hand side of equation (3.33).