Is the Euclidean metric a necessary accessory of Lorentzian spacetime?

Question

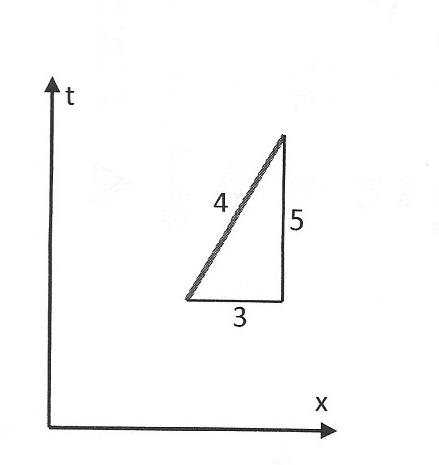
Spacetime manifolds, as well as their simplified form, twodimensional spacetime diagrams, are always Lorentzian (Lorentzian metric $ds^2 = dt^2 - dx^2$). Normally, the Lorentzian metric is hidden because spacetime is represented on a Euclidean support such as a sheet of paper, and we can measure the corresponding Euclidean distance ($ds^2 = dt^2 + dx^2$) even though it is completely meaningless. For instance, the Lorentzian metric in the diagram is $4$, but the measurement of the worldline yields the meaningless distance of $5.83$.

Is there a way to get rid of this superfluous Euclidean metric of the support of observation (such as a sheet of paper), or is the Euclidean metric a necessary accessory of the Lorentzian spacetime manifold?

Answer 1

Here is the attempt of an answer:

The Lorentzian metric of a spacetime diagram is $ds^2= dt^2 - dx^2$ (c=1), and by this, it is a function of the coordinates dt and dx. The Euclidean metric $ds^2= dt^2 + dx^2$ has the same arguments, and for every Lorentzian spacetime interval we may define a Euclidean interval, simply by replacing the minus sign with a plus sign. That means that the Lorentzian spacetime implies automatically the possibility of the existence of a Euclidean metric, and there is no way to "get rid" of this possibility.

The resulting Euclidean metric is not completely meaningless: It is part of a Euclidean manifold which corresponds to the sheet of paper on which is sketched the spacetime diagram. This Euclidean geometry is not referring to the underlying physical reality (which is described by the Lorentzian metric) but it is describing the "interface" of observation. The same is true for fourdimensional spacetime manifolds, we may always define a Euclidean metric where the minus sign of the Lorentzian metric is substituted with a plus sign. By consequence, we may distinguish two metrics with respect to spacetime: The Euclidean metric of the observer and the Lorentzian metric of the underlying observed universe.

Your comments are welcome.