Video: Fan with one blade missing rotates while running.
The fan worked just fine until my friend tried to stop the spinning blades with her finger and knocked one off. Now it always rotates counterclockwise when running. Can someone explain in details why? Does this have something to do with the shape of the blades?
From the video, it looks like that the axis of the fan rotor is not orthogonal to the vertical direction.
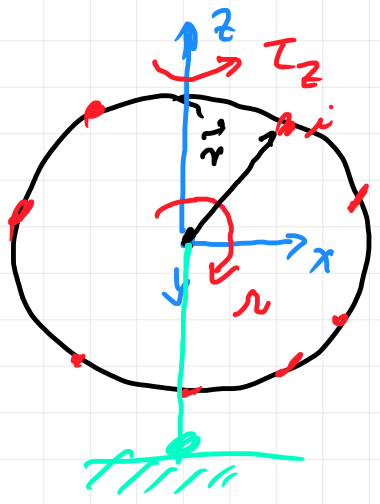
When a motor-driven rotor spins in a direction, a torque acts on it due to aerodynamic drag in the opposite direction, i.e. the torque can be represented by a vector orthogonal to the plane of the rotor, that can be approximately written as
$\mathbf{M}^{aero} = - \dfrac{1}{2} \, C_T \rho R^3 |\mathbf\Omega| \mathbf{\Omega} = - \dfrac{1}{2} \, C_T \rho R^3 |\Omega| \Omega \,\mathbf{\hat{n}}$ ,
being
$C_T$ the torque coefficient depending on the parameters of the blades, of the rotor as a whole and non-dimensional coefficients describing the motion, like Reynolds number
$\rho$ is the air density
$R$ the radius of the rotor
$\mathbf{\Omega} = \Omega \, \mathbf{\hat{n}}$ is the angular velocity of the rotor around its axis, following the right-hand rule for angular velocity. If we choose $\mathbf{\hat{n}}$ so that $\Omega > 0$, from what I get from the video, $\mathbf{\hat{n}}$ points slightly downward, so that we can write $\mathbf{\hat{n}} \cdot \mathbf{\hat{z}} = \sin \alpha < 0$, defining the angle $\alpha$ as the angle between $\mathbf{\hat{n}}$ and the horizontal plane. Now,
if the axis of the fan is orthogonal to the vertical direction $\mathbf{\hat{z}}$, the rotor doesn't introduce any torque around the $\mathbf{\hat{z}}$-axis, since $\mathbf{\hat{z}} \cdot \mathbf{\hat{n}} = 0$ and this aerodynamic action is balanced by normal reactions exchanged by the table and the base of the fan
if the axis of the fan is not horizontal (as it looks like from the video), the aerodynamic torque has a vertical component
$ M_z^{aero} = \mathbf{\hat{z}} \cdot \mathbf{M}^{aero} = - \dfrac{1}{2} \, C_T \rho R^3 |\Omega| \Omega \,\mathbf{\hat{n}} \cdot \mathbf{\hat{z}} = - \dfrac{1}{2} \, C_T \rho R^3 |\Omega| \Omega \sin \alpha > 0$,
that makes the fan rotate around a vertical axis, in the counter-clockwise direction if we look at the fan from above, if the friction between the base and the table is not enough to balance the external action.
This process is independent from the missing blade, but only on the inclination of the rotor plane. You could test this explanation if you have a fan with all the blades, and play around with the direction of the axis of rotation. Even if you only have the broken one, you can change the orientation of the axis, so that it point upwards and see if the fan rotates around z-axis in the opposite direction.
Other contributions (I guess minor, but I currently have no time to do the calculations) could come from the unbalanced rotor.
the red points are the blades center of mass . the rotation about the y-axes ,cause a wind force $~F_w~$ towards the y-axes. the torque about the z-axes ,$~\tau_z~$ cause the ventilator to rotate .
with \begin{align*} \begin{bmatrix} \tau_{xi} \\ \tau_{yi} \\ \tau_{zi} \\ \end{bmatrix} =\begin{bmatrix} r_{xi} \\ r_{yi} \\ r_{zi} \\ \end{bmatrix}\times \begin{bmatrix} 0 \\ F_w \\ 0 \\ \end{bmatrix}\quad\Rightarrow \end{align*}
$$\tau_{zi}=r_{xi}\,F_w\quad\text{hence }\\ \tau_z=F_w\,\sum_{i=1}^n\,r_{xi}$$
the torque $~\tau_z~$ is zero only if $~\sum_{i=1}^n\,r_{xi}=0~$. obviously is for this ventilator not the case
