The $t$ corresponds to the wristwatch time (the proper time) of the inertial observer drawing the spsacetime diagram where this observer is at rest.
In special and general relativity, that $t$ is not a universal time since (as @PM2Ring said in a comment).
Structurally speaking, $t$ is first a property of the worldline of the inertial observer at rest in her spacetime diagram. Some further construction (e.g. radar measurements or some other procedure) is needed to use this $t$ to help label
and assign coordinates to events not this worldline... to figuratively "spread time over space".
update:
I was going to add a comment in response to @kiriloff ,
but then felt it was better to update my initial response.
Given any worldline, $\tau$ is the proper time along that worldline, which is analogous to the arc-length of a curve. As $\tau$ advances along that worldline, the corresponding event on the worldline varies its $(t,x,y,z)$ coordinates, where the coordinates are those set up by the inertial observer (call her Alice) at rest in the spacetime diagram.
So, for Alice's worldline [which is vertical through the origin in her spacetime diagram], as her $\tau_{Alice}$ advances, the corresponding event on Alice's worldline varies its $t$-component (with $t=\tau_{Alice}$) but its $(x,y,z)$ coordinates remain $(0,0,0)$ since
Alice is at rest at origin in her own spacetime diagram.
Here is the radar-measurement procedure to assign coordinates to events in spacetime, especially to events not on her worldline. For a distant event $Q$,
Alice arranges to send a light signal to $Q$. She notes the time $\tau_{send}$ on her wristwatch.
Alice waits for the reflected echo light signal from $Q$. She notes the time $\tau_{receive}$ on her wristwatch.
Alice defines
\begin{align}
t_Q &=\frac{\tau_{receive}+\tau_{send}}{2}\\
x_Q &=c\frac{\tau_{receive}-\tau_{send}}{2}\\
\end{align}
(When $Q$ is on her worldline, then $\tau_{receive}=\tau_{send}$. So, $x_Q=0$ and $t_Q=\tau_{send}=\tau_{receive}$.)
So, by using the light-signals, this is how Alice assigns a time coordinate to a distant event $Q$---essentially assigning "the wristwatch reading $\tau$" of the event on her worldline that Alice says is simultaneous with the distant event $Q$.
Update2:
Note that $\tau_{receive}=(t_Q +x_Q/c)$ and $\tau_{send}=(t_Q -x_Q/c)$
so that
$$\tau_{receive}\tau_{send}=(t_Q +x_Q/c)(t_Q -x_Q/c)=t_Q^2-(x_Q/c)^2.$$
Every inertial observer through the origin event
will generally assign different coordinates to event $Q$.
But they will find (by experiment or by using the Bondi k-calculus, for example)
that the product of their radar times
$\tau_{receive}\tau_{send}$ will be equal (i.e. an invariant... the invariant squared-interval).
In terms of their rectangular coordinates, this product equals
$t_Q^2-(x_Q/c)^2$.
Update3:
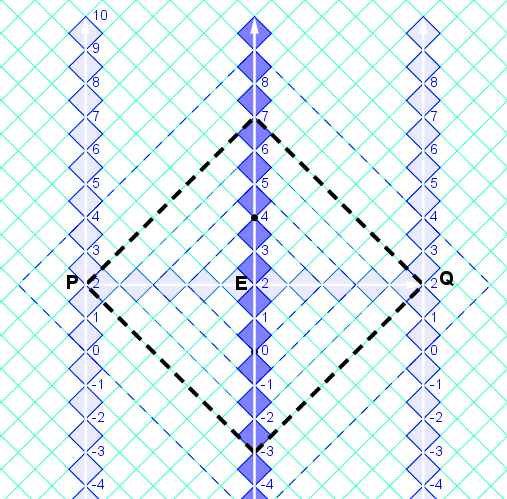
Here's a spacetime diagram of the radar measurement,
in particular, how the Blue inertial observer uses Blue's wristwatch and radar
to assign the time-coordinate $t=2$ to events not on Blue's worldline.
$$\tau_{receive}=7 \quad \tau_{send}=-3 \qquad\mbox{so }\tau_{receive}\tau_{send}=-21 $$
$$t_Q=\frac{7+(-3)}{2}=2\quad x_Q=\frac{7-(-3)}{2}=5 \qquad\mbox{so }
t_Q^2-x_Q^2=(2)^2-(5)^2=-21$$
Here is that radar experiment viewed from a different inertial frame
where Blue has, say, $v_{Blue\ wrt\ Red}=(3/5)c$ (so, Doppler $k=2$).
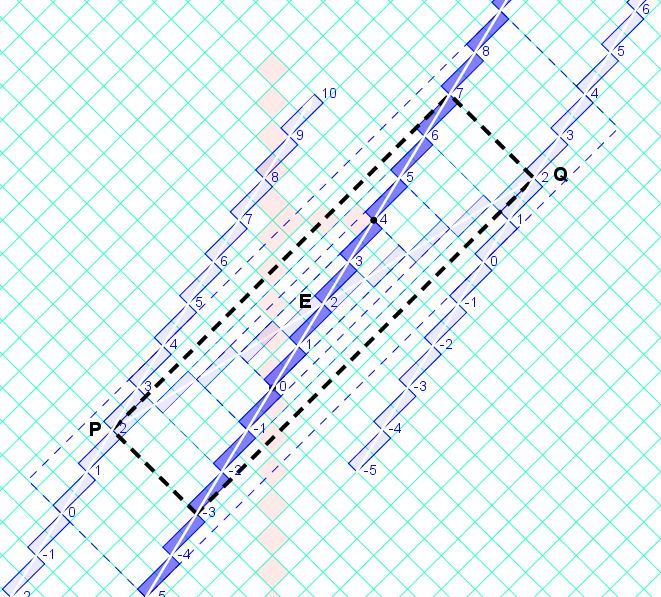
By the way, although not drawn in, Red can also measure $Q$ with radar
$$\tau_{red,receive}=14 \qquad \tau_{red,send}=-3/2 \qquad \mbox{so }\tau_{red,receive}\tau_{red,send}=-21 $$
$$t_{rQ}=\frac{14+(-3/2)}{2}=\frac{25}{4}=6.25\quad x_{rQ}=\frac{14-(-3/2)}{2}=\frac{31}{4}=7.75$$
$$\qquad\mbox{so }
t_{rQ}^2-x_{rQ}^2=(6.25)^2-(7.75)^2=-21$$


