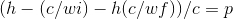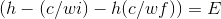The question asks about the time dependence of the function
$$f(t) := \langle\psi(t)|(\Delta \hat{x})^2|\psi(t)\rangle
\langle\psi(t)|(\Delta \hat{p})^2|\psi(t)\rangle,$$
where
$$\Delta \hat{x} := \hat{x} - \langle\psi(t)|\hat{x}|\psi(t)\rangle, \qquad
\Delta \hat{p} := \hat{p} - \langle\psi(t)|\hat{p}|\psi(t)\rangle, \qquad
\langle\psi(t)|\psi(t)\rangle=1.$$
We will here use the Schrödinger picture where operators are constant in time, while the kets and bras are evolving.
Edit: Spurred by remarks of Moshe R. and Ted Bunn let us add that (under assumption (1) below) the Schroedinger equation itself is invariant under the time reversal operator $\hat{T}$, which is a conjugated linear operator, so that
$$\hat{T} t = - t \hat{T}, \qquad \hat{T}\hat{x} = \hat{x}\hat{T}, \qquad \hat{T}\hat{p} = -\hat{p}\hat{T}, \qquad \hat{T}^2=1.$$
Here we are restricting ourselves to Hamiltonians $\hat{H}$ so that
$$[\hat{T},\hat{H}]=0.\qquad (1)$$
Moreover, if
$$|\psi(t)\rangle = \sum_n\psi_n(t) |n\rangle$$
is a solution to the Schrödinger equation in a certain basis $|n\rangle$, then
$$\hat{T}|\psi(t)\rangle := \sum_n\psi^{*}_n(-t) |n\rangle$$
will also be a solution to the Schrödinger equation with a time reflected function $f(-t)$.
Thus if $f(t)$ is non-constant in time, then we may assume (possibly after a time reversal operation) that there exist two times $t_1<t_2$ with $f(t_1)>f(t_2)$. This would contradict the statement in the original question. To finish the argument, we provide below an example of a non-constant function $f(t)$.
Consider a simple harmonic oscillator Hamiltonian with the zero point energy $\frac{1}{2}\hbar\omega$ subtracted for later convenience.
$$\hat{H}:=\frac{\hat{p}^2}{2m}+\frac{1}{2}m\omega^{2}\hat{x}^2
-\frac{1}{2}\hbar\omega=\hbar\omega\hat{N},$$
where $\hat{N}:=\hat{a}^{\dagger}\hat{a}$ is the number operator.
Let us put the constants $m=\hbar=\omega=1$ to one for simplicity. Then the annihilation and creation operators are
$$\hat{a}=\frac{1}{\sqrt{2}}(\hat{x} + i \hat{p}), \qquad
\hat{a}^{\dagger}=\frac{1}{\sqrt{2}}(\hat{x} - i \hat{p}), \qquad
[\hat{a},\hat{a}^{\dagger}]=1,$$
or conversely,
$$\hat{x}=\frac{1}{\sqrt{2}}(\hat{a}^{\dagger}+\hat{a}), \qquad
\hat{p}=\frac{i}{\sqrt{2}}(\hat{a}^{\dagger}-\hat{a}), \qquad
[\hat{x},\hat{p}]=i,$$
$$\hat{x}^2=\hat{N}+\frac{1}{2}\left(1+\hat{a}^2+(\hat{a}^{\dagger})^2\right), \qquad
\hat{p}^2=\hat{N}+\frac{1}{2}\left(1-\hat{a}^2-(\hat{a}^{\dagger})^2\right).$$
Consider Fock space $|n\rangle := \frac{1}{\sqrt{n!}}(\hat{a}^{\dagger})^n |0\rangle$
such that $\hat{a}|0\rangle = 0$. Consider initial state
$$|\psi(0)\rangle := \frac{1}{\sqrt{2}}\left(|0\rangle+|2\rangle\right), \qquad
\langle \psi(0)| = \frac{1}{\sqrt{2}}\left(\langle 0|+\langle 2|\right).$$
Then
$$|\psi(t)\rangle = e^{-i\hat{H}t}|\psi(0)\rangle
= \frac{1}{\sqrt{2}}\left(|0\rangle+e^{-2it}|2\rangle\right),$$
$$\langle \psi(t)| = \langle\psi(0)|e^{i\hat{H}t}
= \frac{1}{\sqrt{2}}\left(\langle 0|+\langle 2|e^{2it}\right),$$
$$\langle\psi(t)|\hat{x}|\psi(t)\rangle=0, \qquad
\langle\psi(t)|\hat{p}|\psi(t)\rangle=0.$$
Moreover,
$$\langle\psi(t)|\hat{x}^2|\psi(t)\rangle=\frac{3}{2}+\frac{1}{\sqrt{2}}\cos(2t), \qquad
\langle\psi(t)|\hat{p}^2|\psi(t)\rangle=\frac{3}{2}-\frac{1}{\sqrt{2}}\cos(2t),$$
because $\hat{a}^2|2\rangle=\sqrt{2}|0\rangle$. Therefore,
$$f(t) = \frac{9}{4} - \frac{1}{2}\cos^2(2t),$$
which is non-constant in time, and we are done. Or alternatively, we can complete the counter-example without the use of above time reversal argument by simply performing an appropriate time translation $t\to t-t_0$.