Electrodynamics - Faraday law in moving circuit (Jackson's ED)

Question

I have had this issue with Jackson's derivation of Faraday law in a moving circuit for quite some time and have not been able to resolve it. The relevant parts of the book is p. 211-212 in 2nd edition, and p. 209-211 in the 3rd ed.

Introduction:

So, let's have an open surface $S$, its normal $\vec{n}$ and its boundary $C\equiv\partial S$ as in the image:

Here, I will be using $\mathrm{d}S$, instead of $\mathrm{d}a$ and let this surface be in a magnetic field $\vec{B}$. And let this surface be moving with some constant velocity $\vec{v}$ with respect to a laboratory system.

The first claim of Jackson is the general statement of the Faraday law in the form:

$$ \mathcal{E} = -k\frac{\mathrm{d}F}{\mathrm{d}t}\tag{1}\,, $$ where: $F = \int_S \vec{B}\cdot\vec{n}\;\mathrm{d}S\,,$ and $\mathcal{E} = \oint_C \vec{E}'\cdot\mathrm{d}\vec{l}$.

Later in the text, Jackson states:

The electric field $\vec{E}'$ is the electric field at $\mathrm{d}\vec{l}$ in the coordinate system or medium in which $\mathrm{d}\vec{l}$ is at rest, since it is that field which causes current to flow if a circuit is actually present.

Then, however, he expands the derivative of the flux as:

$$ \frac{\mathrm{d}}{\mathrm{d}t}\int_S\vec{B}\cdot\vec{n}\;\mathrm{d}S = \int_S\frac{\partial \vec{B}}{\partial t} \cdot\vec{n}\;\mathrm{d}S + \oint_C (\vec{B}\times\vec{v})\cdot\mathrm{d}\vec{l}\tag{2}\,, $$

and claims that he can recast (1) as: $$ \oint_C [\vec{E}' -k (\vec{B}\times\vec{v})]\cdot\mathrm{d}\vec{l} = -k\int_S\frac{\partial \vec{B}}{\partial t} \cdot\vec{n}\;\mathrm{d}S\tag{3}\,. $$

And then he claims that if we think of the surface as instantaneously at certain position in space in the laboratory system, we can also write the Faraday law for the same circuit as:

$$ \oint_C \vec{E}\cdot\mathrm{d}\vec{l} = -k\int_S\frac{\partial \vec{B}}{\partial t} \cdot\vec{n}\;\mathrm{d}S\tag{4}\,. $$

Question:

In going to Eq. (3), he made the assumption that we are in the system connected with the moving circuit $C$, but then, I do not understand how he arrives at the right hand side of (2), since the velocity in that system is zero and the last term on the right hand side of (2) should not be there.

Bonus question: He only uses convective derivative inside the integral of the flux, but the surface over which the integration is being done also depends on time. I am missing a term related to that but my guess is that it will be zero due to the constant velocity. However, does someone have a rigorous derivation of that missing term?

Addendum

Here are actual excerpts from the 2nd edition:

Answer 1

A clean presentation of Faraday's law for moving circuits is one of the most critical parts of electromagnetism. Even popular textbooks contain presentations that are not particularly clear for novices and in some cases, misleading.

In my experience, the clearest introduction of Faraday's law in its full generality requires understanding that the so-called motional emf is just a way of rewriting the contribution to the time derivative of the flux, coming from the time dependence of the boundary of the surface integral at constant magnetic field: $$ \frac{{\rm d}\Phi}{{\rm d}t}=\frac{{\rm d}}{{\rm d}t}\int_{S(t)} dS ~\vec n \cdot\vec B = \oint_{C(t)} (\vec B \times \vec v)\cdot d\vec l. $$ Here $\vec v$ is the local velocity of the circuit element $d\vec l$ in the reference frame where the circuit is moving.

In general, the total time derivative of the flux contains another term corresponding the flux at fixed circuit, of the partial time derivative of the $\vec B$ field. This term is related, with a minus sign, to the line integral of the induced electric field in the reference frame where the circuit is moving. Thus, the full expression of the flux variation can be written as $$ -\frac{{\rm d}\Phi}{{\rm d}t}=\oint_C (\vec E + \vec v \times \vec B)\cdot d\vec l. $$

Only at this point, it could be helpful to observe that the right-hand side of such an expression is the line integral of the field $\vec E'$, in the instantaneous reference frame where the circuit element $d \vec l$ is at rest.

In the original reference frame, it is nothing but the line integral of the total force (electric end magnetic) acting per unit charge (the emf).

Answer 2

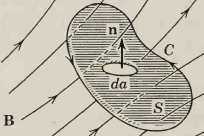
In going to Eq. (3), he made the assumption that we are in the system connected with the moving circuit CC, but then, I do not understand how he arrives at the right hand side of (2), since the velocity in that system is zero and the last term on the right hand side of (2) should not be there.

The equation (2) does not involve changing frames, all terms refer to the original lab frame. It is a mathematical theorem for any divergence-less field, and it is easy to see in the Eulerian picture (see Jackson's footnote on p.212, 2nd edition) that it is true for magnetic field in regular circumstances (motion of the loop is continuous, magnetic field finite everywhere, line integral remains defined, and so on).

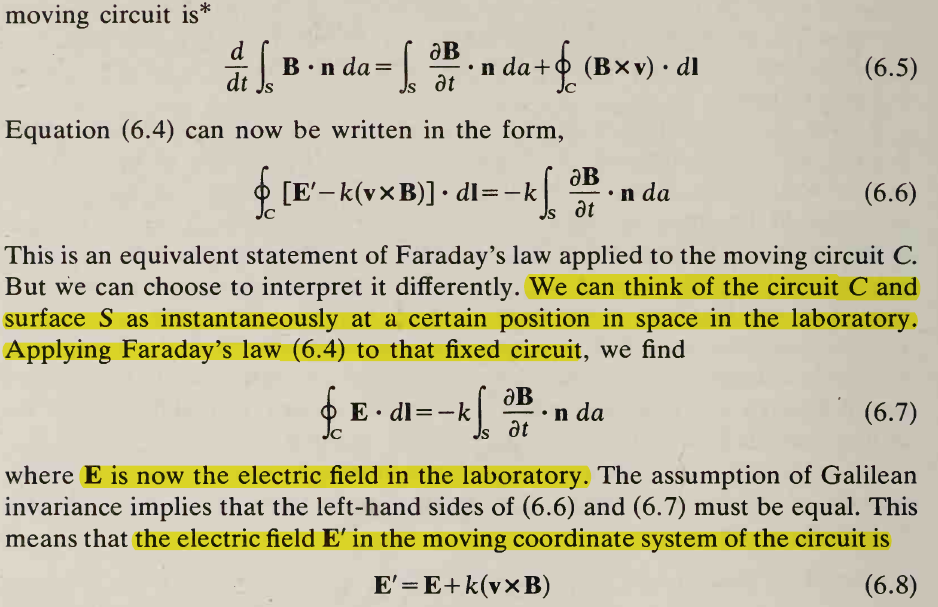
There is also a more complicated way to derive it formally, like similar theorems in continuum mechanics; the flux integral for a moving surface is a function of time both because $\mathbf B$ can change, and because the loop $C$ changes. To perform the time derivative on the integrand, one must first fix the integration domain. So a change of variables of integration is made. Imagine the loop is made of matter. Then any loop point $k$ has not only a definite position $\mathbf r_k(t)$ but also definite velocity $\mathbf v_k(t)$. The position can be expressed as $\mathbf r_k(t) = \mathcal{F}_t(\mathbf r_{k,0})$, where $\mathcal{F}_t$ is a continuous function of time and initial positions $\mathbf r_{k,0}$. It takes material point position at time 0 and returns its position at time $t$.

When the flux integral is expressed using the new integration variables $\mathbf r_{k,0}$, the integration domain becomes fixed - it is the domain occupied by the loop surface at the initial time, which does not depend on time. Now, time derivative can be brought into the integrand, and $\mathcal{F}_t$ gets differentiated, together with Jacobi's determinant of the variable transformation, and this is where velocity $\mathbf v$ and cross product with $\mathbf B$ come from. In the final step, the resulting integral is re-expressed back into the original coordinates, as integral over the coordinates of the surface attached to the loop at time $t$. Also the fact that $\nabla \cdot \mathbf B = 0$ is used (as mentioned in Jackson's footnote).

A critique of Jackson's exposition.

I get an uneasy feeling from it.

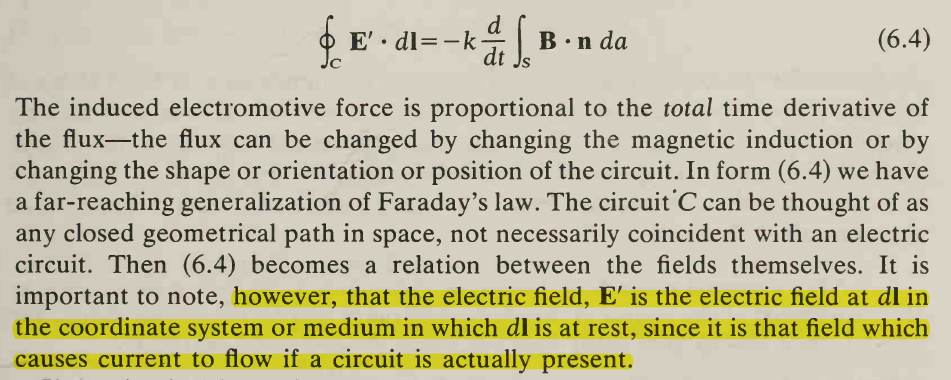
Jackson's claims and results until and including formula 6.7 are correct, but his way of presenting them is probably very confusing to students, because despite the name of the section 6.1 "Faraday's law of induction", it is not about explaining this law; instead it is about stating and using this law, in its generalized formulation for any moving loop, to derive some implications, such as transformation properties of electric field, or to show how one of Maxwell's equation is arrived at. These are related things but not the core of the topic implied by the title of the section. I think this can confuse the student who does not yet understand the Faraday law in its full generality.

He bases his derivations on a tacit non-trivial assumption: that Faraday's law is about a very specific concept of loop emf:

$$ \mathscr{E} = \oint_C \mathbf E' \cdot d\mathbf l $$

where $\mathbf E'$ is electric field in the co-moving frame of the loop element $d\mathbf l$, instead of the more intuitive definition

$$ \mathscr{E} = \oint_C \mathbf E_{imp} \cdot d\mathbf l $$ where $\mathbf E_{imp}$ is net impressed force per unit charge in the lab frame, acting on mobile charges in the circuit. This impressed force has contributions due to external electric field, external magnetic field, and lattice acting on the mobile charges to keep them inside the conductor.

According to this specific definition adopted by Jackson, emf in Faraday's law is a frame-independent quantity (assuming the Galilei transformation), and due to Faraday's law, also magnetic flux is a frame-independent quantity. Jackson offers no justification for this assumption. We should have doubts on this at this stage, since if electric field is not the same in two mutually moving frames, why should magnetic field or magnetic flux be the same?

It turns out that all this is correct, at least in non-relativistic motions, but it is also pulled out of the thin air as a tacit plausible assumptions, lacking motivation or justification.

Then there is the strange argumentation for formula 6.8; Jackson suggests that assumption of Galilean invariance somehow implies the relation between electric fields in two frames

$$ \mathbf E' = \mathbf E + \mathbf v \times \mathbf B. $$

I strongly disagree; because all equations work with integrals over closed paths, the only thing his argument (using the above assumptions) shows is that integral over any closed path $C$

$$ \oint_C \big( \mathbf E' - (\mathbf E + \mathbf v \times \mathbf B)\big) \cdot d\mathbf l = 0, $$ i.e. we have

$$ \mathbf E' = \mathbf E + \mathbf v \times \mathbf B + \mathbf K(\mathbf v) $$

where $\mathbf K$ is some unknown conservative field, possible a function of velocity distribution on the loop.

I don't see how his ideas imply that $\mathbf K$ must vanish. For example, if the whole circuit moves with the same velocity $\mathbf v$, it could be that $\mathbf K =A\mathbf v$. My conclusion is that one can't derive transformation properties of electric field just from Faraday's law, which is not that surprising since it does not care for conservative part of the field. One would better base such derivation on invariance of the Lorentz force formula on any single particle. Net EM force manifests total external EM field, not just effect of non-conservative part of it.