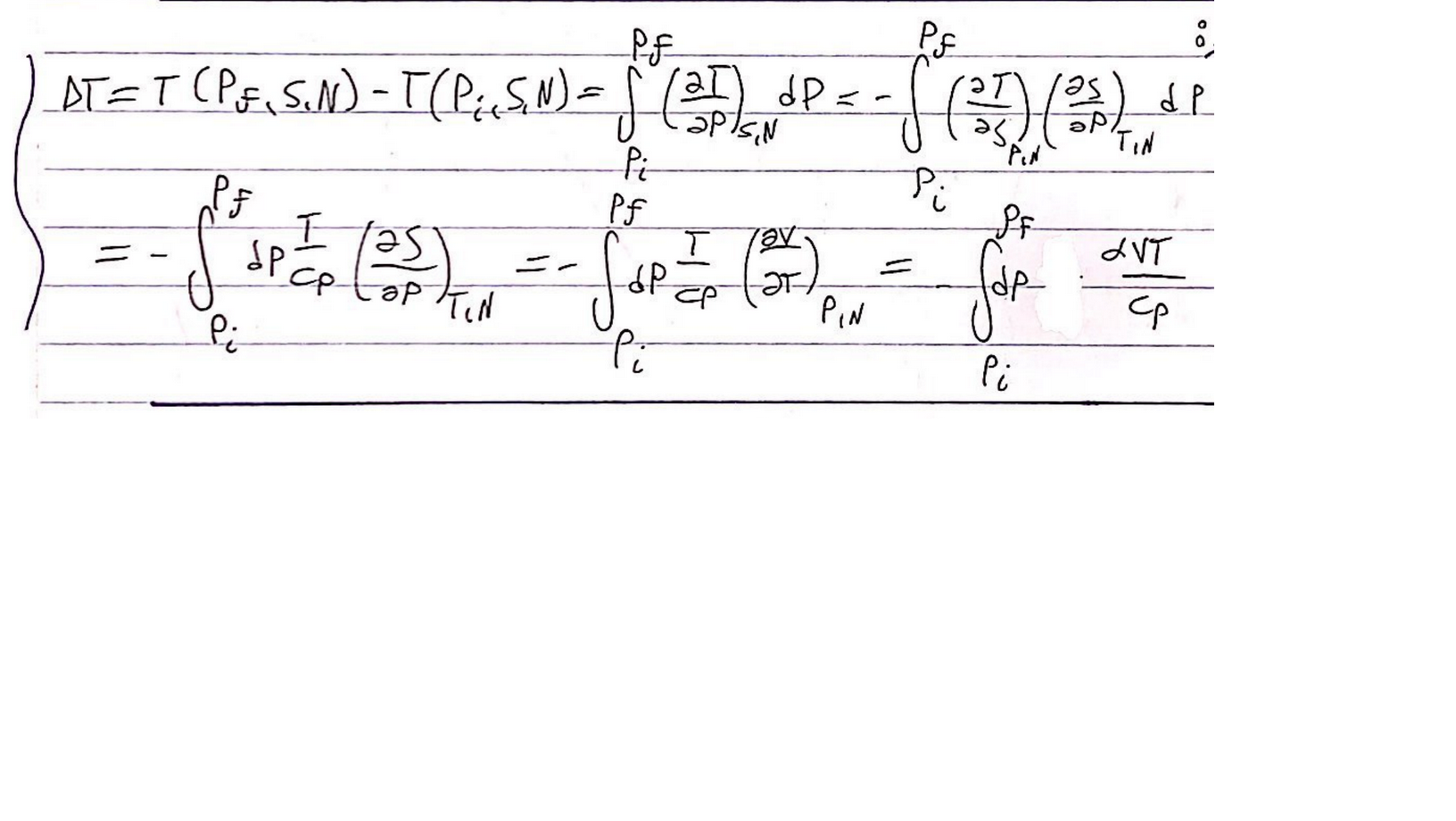
In my homework assignment there's the following question:
A general thermodynamic system is being compressed isentropically from pressure $P_i$ to $P_f$ while keeping the number of particles constant. write down the temperature change of the system using an integral. reduce the partial derivative in the integrand to the measurable quantities: $T,V,\alpha$, $C_p$.
The solution to the question is the following:
My question is: What was used in the transition from $\Delta T$ to the first integral over $dp$? how would one approach this question and get the correct result as seen above?