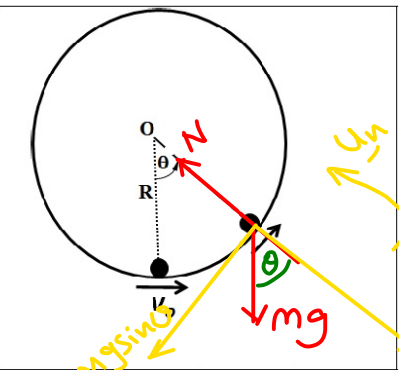
If we have a body like the one below , What will be the minimum initial velocity $V_0$ to complete one revolution, My assumption was that it has to reach $\theta=180$ ,But how do I describe this mathematically and why?
$\begingroup$
$\endgroup$
6
-
$\begingroup$ A fee body follows a straight line, Newtons first law. en.wikipedia.org/wiki/Newton%27s_laws_of_motion a force is needed to make a circular path, $\endgroup$– anna vCommented Jun 7, 2022 at 9:29
-
$\begingroup$ @annav By free here I mean that it can leave the path, it's not guided(Like a ball in a closed circular tube). $\endgroup$– PoliceProgrammerCommented Jun 7, 2022 at 9:45
-
$\begingroup$ So you mean that a radial force will act to keep it on the circle inwardly, but not outwardly? And you want the minimum velocity for it to not leave the path at the top? $\endgroup$– sqekCommented Jun 7, 2022 at 10:59
-
$\begingroup$ @annav how come it ? specify more like for example if at $\theta=180$ and the normal reaction $N=0$ how will the body not become a projectile instantly ? $\endgroup$– PoliceProgrammerCommented Jun 7, 2022 at 11:47
-
$\begingroup$ @sqek no there is no radial force we want to launch the ball with a initial launch velocity to make it complete a revolution without returning or falling off $\endgroup$– PoliceProgrammerCommented Jun 7, 2022 at 11:51
|
Show 1 more comment
2 Answers
$\begingroup$
$\endgroup$
From what I think you mean from
Like a ball in a closed circular tube
the radial or normal force from the tube, $N$ in the diagram above, can only be positive. If $N$ is negative, the ball will fall away from the wall of the tube.
Radially, the ball needs a centripetal acceleration of $\frac{V^2}{R}$. So using $f=ma$ in the radial direction, $N-mg\cos\theta=m\frac{V^2}{R}$.
Assuming no friction, conservation of energy (kinetic + gravitational potential) gives
$\frac{1}{2}mV^2-mgR\cos\theta=$constant$=\frac{1}{2}mV_0^2-mgR$
So $mV^2=mV_0^2+2mgR(\cos\theta-1)$
So $N=\frac{1}{R}mV_0^2+3mg\cos\theta-2mg$
$N$ is at its minimum when $\theta=180^o$, when $\cos\theta=-1$. The limiting speed is when $N=0$ at this instant - $N$ can't be negative because the tube can't pull the ball outward, so if $N<0$ the ball falls away. So $N_{min}=\frac{1}{R}mV_{0,min}^2-5mg\ge0$ (one of the $mg$ is from gravity pulling the ball down, the rest is from the change in velocity from $V_0$ as the ball has risen $2R$)
So $V_{0,min}=\sqrt{5gR}$
$\begingroup$
$\endgroup$
To do a loop over the top the velocity at the top ($v_{top}$) has to be such that the centripetal acceleration is at least $g$ (otherwise it will fall down). This is:
$$a= v^2 / r$$
So:
$$g = {v_{top}}^2 / r$$ $${v_{top}}^2 = gr$$
In turning the half circle, the ball will gain potential energy $2mgr$. That must translate to a loss of kinetic energy, so:
$$\frac 1 2 m{v_{0}}^2 - \frac 1 2 m{v_{top}}^2 = 2mgr$$ $${v_{0}}^2 - {v_{top}}^2 = 4gr$$ but ${v_{top}}^2 = gr$, so $${v_0}^2 - gr = 4gr$$ $${v_0} = \sqrt{5gr}$$
