Limitations of spring and dashpot models in terms of strain meaningfulness

Question

It is common to explain viscoelastic materials with spring and dashpot 1D constructions, e.g:

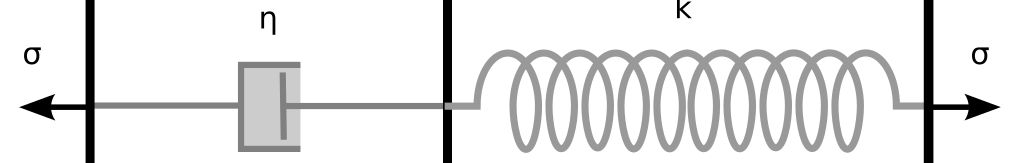
which represents a Maxwell rheology, usually explained by saying that $ \sigma = \eta \dot{\epsilon}_1 = k \epsilon_2$, and $\epsilon = \epsilon_1+\epsilon_2$. Differentiating the $\epsilon_2$ part and summing, one does get $\frac{\eta}{k}\dot{\sigma}+\sigma=\eta\dot{\epsilon}$

While I have well been aware of the caveats of these convenient representations before extrapolating to 2 or 3D continuum materials, I am surprised to realise now that there seems to be a first one which holds even when keeping very close to what is represented: strain rates shouldn't sum...?

If we relate strain rates with the length $l_i$ of each element ($i=1,2$), $\dot{\epsilon}_i = \dot{l}_i/l_i$ while $\dot{\epsilon}=\frac{\dot l_1+\dot l_2}{l_1+l_2}$, which I find difficult to reduce to an approximation of $\dot{\epsilon}_1+\dot{\epsilon}_2$ with reasonable assumptions.

I am aware that the overall answer is that these are only toys to help conceive how models derived otherwise do work, however I'm wondering if there's any more precise reason why they work so relatively well in these conditions. Can one state explicitly the limits of validity of these toy models? Is one e.g. reduced to consider that we are around some reference state $l_i=l_i^0$, and if so how can such a model provide information on liquid-like systems?

Answer 1

You've essentially discovered a failure mode (or edge case, or limitation) of applying certain lumped-component models: They're all assumed to have the same length, or alternatively, it's not meaningful to talk about their length, or alternatively, $\sigma$–$\varepsilon$ doesn't represent a real stress–strain conjugate pair.

For example, if two springs are placed in series, we conventionally have $\varepsilon_1=\frac{\Delta L_1}{L_1}$ and $\varepsilon_2=\frac{\Delta L_2}{L_2}$ (for lumped-component modeling, these would be $\frac{\Delta L_1}{L}$ and $\frac{\Delta L_2}{L}$, respectively, if the strain terms can be said to have any deeper meaning). But for the lumped-component framework to be consistent, the total strain must be not $\frac{\Delta L_1+\Delta L_2}{L_1+L_2}$ but $\frac{\Delta L_1+\Delta L_2}{L}=\varepsilon_1+\varepsilon_2.$ In other words, we must maintain that every spring combination has the same length $L$.

The reason is that we would like two idealized spring units placed in series to have half the spring constant, to maintain symmetry with the way that placing two units in parallel doubles the spring constant. This behavior also recapitulates the way that a series combination of two real springs has half the spring constant of each individual spring.

But in any case, note that $k$ cannot truly be a spring constant because $\frac{\sigma}{\varepsilon}$ is a material property—Young's modulus, $E$—that remains identical if we combine multiple springs into an assembly. (After all, the spring material itself remains unchanged.) This tells us that we can't treat these idealized lumped models as real objects with finite geometry—at least without breaking some other rules of how real objects behave and how we define mechanical terms.

Answer 2

The spring-and-dashpot models, when they are used to model the stress-strain relationship, should be understood and applied only at the linear viscoelastic limit. As has been pointed out by others, considering the deformation of a spring-and-dashpot model as "uniaxial tension", the strain addition whenever needed would be like $\varepsilon_1 +\varepsilon_2=\frac{L_2\Delta L_1+L_1\Delta L_2}{L_1L_2}$, while the total strain should be $\varepsilon=\frac{\Delta L_1+\Delta L_2}{L_1+L_2}$. The two expressions equal only when the strain are infinitesimally small, i.e. when $\Delta L_1\to 0$ and $\Delta L_2\to 0$, the difference between $\varepsilon_1+\varepsilon_2$ and $\varepsilon$ vanishes.

In fact, the constitutive equation of linear viscoelasiticity:

$$\boldsymbol{\tau}\left(t\right)=\int_{-\infty}^{t}G\left(t-t^\prime\right)2\mathbf{D}\left(t^\prime\right)\mathrm{d}t^\prime$$

is also valid (in the sense that it is exactly the result of the Boltzmann superposition principle) only at the linear viscoelastic limit, i.e. $\mathbf{F}\rightarrow\mathbf{I}$. Relaxing this limiting requirement, Boltzmann superposition principle gives at most the following:

$$\boldsymbol{\tau}\left(t\right)=\int_{-\infty}^{t}G\left(t-t^\prime\right)\dot{\mathbf{B}}\left(t^\prime\right)\mathrm{d}t^\prime$$

This means that requiring the interpretation to be confined under linear viscoelastic limit is not an ad hoc accommodation specifically for the spring-and-dashpot models to be sounder, but an intrinsic prerequisite of the theory of linear viscoelasticity.

But I prefer introducing spring-and-dashpot models not for modeling directly the stress-strain relation, but for the form of stress relaxation modulus $G\left(t\right)$. For example, the Maxwell model gives $G\left(t\right)=\frac{\eta^2}{E}e^{-Et/\eta}$. The Kelvin--Voigt model gives $G\left(t\right)=E+\eta\delta\left(t\right)$. There are more admissible forms of $G\left(t\right)$ that cannot be represented by spring-and-dashpot models with finite number of elements, for example the stretched exponential form. Although to arrive at these forms one still needs to consider a "stress relaxation on the spring-and-dashpot models", and the problem of the scalar-valued strains and stresses is still involved. But these are only heuristic concept only for the establishment of the correspondence between $G\left(t\right)$ and a spring-and-dashpot diagram. After such correspondence is arrived the scalar-valued strains and stresses are not relevant any more. The resulted $G\left(t\right)$ should be used in a constitutive equation of viscoelasticity in any application.