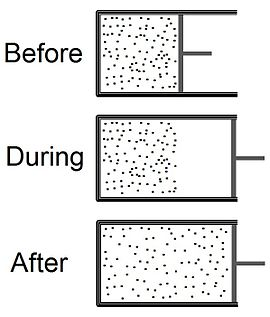
Given an free expansion of a gas to twice the volume. How exactly is the work 'lost' related to the entropy 'gained'?
Proposed solution:
The change in entropy would be, however I don't really understand this formula:
$$ \Delta S = nR \ln \frac{V}{V_0}= nR \ln 2$$
Work that could've been done is:
$$ W= \int_{V_0}^V P dV= \int_{V_0}^V \frac{nRT}{V} dV = nRT \ln \frac{V}{V_0 }= nRT \ln 2$$
So the energy (work) 'lost' to the universe is for example 300 Joules. Then the entropy gained is this energy lost per kelvin (e.g. at 30 kelvin, 10 joules per kelvin.). Why is entropy not just defined by energy 'lost' but specifically energy lost per kelvin?
Why is the entropy gained not related to temperature looking at to the work 'lost' in this process?
Is there a corresponding way to look at heat and entropy?
$$ U_{\text{lost}} = nRT \ln 2 $$