Are water molecules at the surface closer or farther apart than the molecules inside?

Question

My lecturer says that since the energy of the molecules on the surface is higher (less negative), then at equilibrium there will be less molecules on the surface, hence the molecules on the surface are farther apart.

On the other hand, in Khanacademy, in this video for example , he says at 1:50 that the molecules will get a little more close to their neighbors. And also in here "closer spacing at the surface".

And also there is this video:

On the third hand, my lecturer also said that water is not compressible. Doesn't it mean that the spacing between the molecules will stay the same?

Answer 1

The usual attempt to explain surface tension shows two molecules in a surface and in the bulk, where the surface molecule only experiences attractive forces from one side, while the bulk molecule experiences attractive forces from all sides. This image is misleading because it makes us believe that the understanding of static forces was sufficient to understand what is really happening on the molecular level.

Actually, the Lennard-Jones-Potential is a good model for understanding the balance between short-ranged repulsive and long-ranged attractive intermolecular forces, but it implies that, statically, the molecules would be in equilibrium as soon as they get into contact with each other at the equilibrium distance, just like sticky spheres. From that point of view, there would be no inclination to believe that the molecules in the surface have a greater or smaller distance from each other, than the molecules in the bulk, just because that distance is (supposedly) equal to the corresponding unchanged distance value $r_m$ of the Lennard-Jones-Potential.

However, the static picture is not the whole story. Otherwise, what would be the difference between a liquid and a solid? They both are characterized by molecules getting in more or less tight contact with each other due to attractive forces. But in the liquid, the kinetic energy of the molecules is enough to make them constantly swap their places, as opposed to the solid, where molecules largely stay in their determined lattice sites. So, undoubtedly, molecular motion is essential for understanding the liquid state.

As soon as motion of the molecules comes into play, it is rather easy to understand what its effect on the relative distance between molecules will be. If you just consider two molecules with a Lennard-Jones potential, they will somehow oscillate in this potential. But contrary to a movement in a purely harmonic (or at least a symmetric) potential, whose average position is strictly in the center where the potential also has its static minimum, the Lennard-Jones potential is "extremely" asymmetric, resulting in the fact that any motion will shift the average position away from the static equilibrium to greater distances, where only the weaker attractive forces have to be surmounted. This is shown in the following graph (note, that the "average distances" are only roughly depicted in the center of the horizontal bars, while the asymmetry of the motion itself would also shift the average yet a little more to the right)

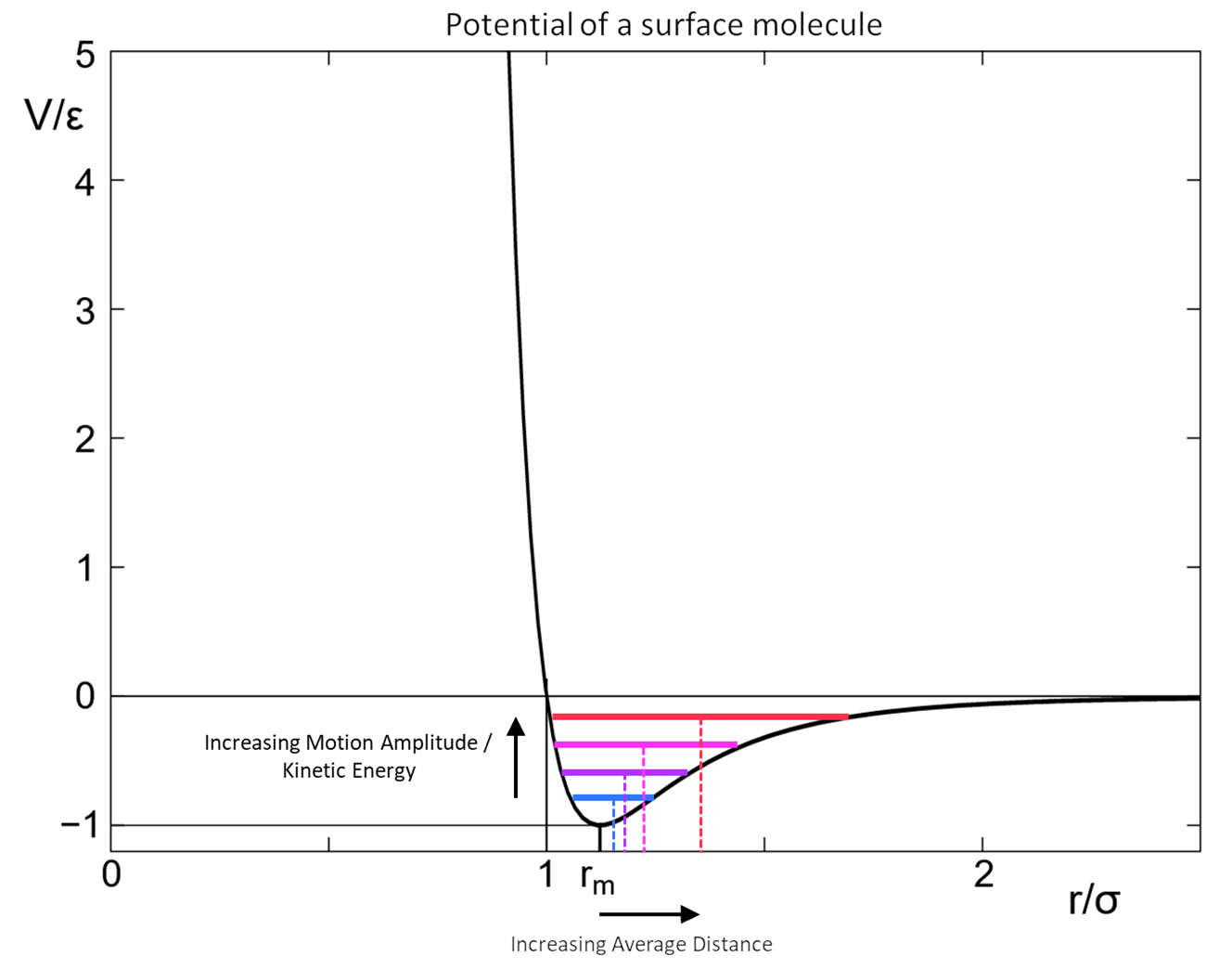
In the bulk liquid, molecules experience collisions with other molecules from all sides, which limit their motion, and hence, also limit the Lennard-Jonesy increase of their relative distance to each other. But at the surface, the repulsive collision part of the forces is missing on one side, and so, the molecules there are only kept to the liquid due to attractive forces. The corresponding greater liberty of motion leads to an increase of average intermolecular distance at the surface. Since all this depends on collisions (or lack thereof), i.e. a dynamic (mass-dependent) effect, this once more underlines that any static picture of the liquid state is insufficient.

What remains to be clarified is the relation between this picture and surface tension. For an answer, compare a planar region of the surface with a positively curved region of the surface. A molecule located at the curved surface region will clearly have less neighbours than its cousin located at the plane surface region, just because of the curved geometry. But there are also two kinds of neighborhood: the neighborhood for the short-ranged repulsive forces is narrower ("sharper"), while the neighborhood for the long-ranged attractive forces is wider ("more blurry"). The consequence of this is that, as curvature increases, the number of repulsive neighbors decreases faster than the number of attractive neighbors. Therefore, for curved surface regions, the attractive forces will dominate, and hence, the molecules there will tend to get drawn into the bulk, even though the local surface region still shows lower density (but a little less so than for the plane surface). The bottom line is that there is no contradiction between lower surface density and curved regions getting pulled into the bulk. You don't need to invent a pre-tensioned membrane concept to explain surface tension.

Consider the analogy, that you are dancing pogo in a crowd at a punk-rock concert. Your desire to be part of the group is the analogy to the attractive forces between molecules, which keeps the group together, although there are always collisions which keep you and your fellow dancers apart. If you are inside the bulk of dancers, you will not notice any anisotropy that would draw your motion preference into one or the other direction. But if you are at the boundary of the dancing group, you are competing with the other dancers at the boundary for a place inside (wouldn't punks actually defy the laws of capitalist competition, what an irony...), which, by the way, also constitutes a kind of surface tension because it favors a spherical boundary of the dancing group. And albeit attraction keeps the group together, there is still the effect that the density at the boundary is a little lower, because you are always bumped at from inside the crowd.

Answer 2

The molecules in the surface are further apart. That's why the surface is under tension. [Consider the shape of the intermolecular force curve.]

But why are the surface molecules further apart than those in the bulk? The molecules are in constant motion, swapping places with their neighbours. Less energy is needed for a surface molecule to move 'down' into the bulk than vice versa, because a surface molecule doesn't have to do work against attractive forces from molecules above it. So if a surface is newly created the rate of transfer of molecules from surface to bulk will be greater than vice versa. (Dynamic) equilibrium is established when there are fewer molecules in the surface layer.

Answer 3

There are good answers already, but I wanted to respond to your last paragraph:

my lecturer also said that water is not compressible. Doesn't it mean that the spacing between the molecules will stay the same?

Nothing is absolutely incompressible. Water is difficult, not impossible, to compress. Its bulk modulus is large, not infinite.

Liquids and solids have an equilibrium density; if they're denser, they're under pressure, and if less dense, they're under tension. The water in the pipe feeding your home is denser than it would be if it wasn't pressurized, though only very slightly, since the bulk modulus is so high. (The bulk modulus of water is about 300,000 psi, and residential water pressure is about 60 psi, so the water is about 60/300000 = 0.02% denser.)

The three videos you linked are wrong, and it's a surprisingly elementary mistake, since tension never works that way: it's always associated with a higher intermolecular spacing (lower density). Perhaps they're thinking of the tension as a separate force acting against the usual intermolecular force, as though the water was inside a rubber balloon. Really, there are no other forces (at least if it's a droplet in vacuum), and the tension and the usual intermolecular force are one and the same.

Answer 4

Background

Consider for this discussion that we can view across a plane of infinitesimal thickness as a hypothetical construction. Consider also that we are not interested in metastable events such as suspending drops on water surfaces or relaxation times for surface events such as formation or change; we are interested only in the (dynamic but stable) equilibrium state.

• Surface tension can be explained directly as a mechanical phenomena. In a molecular view, forces that arise laterally within the surface plane are due to intermolecular interactions within the plane. The force needed to pull molecules apart is obtained from a derivative of the bond potential energy $F = dU/dr$. We have no need to know the position for the surface plane, the organization of molecules within the surface plane, the density of molecules in the surface layer, or the thickness for the actual surface plane. We also seem to have no need to know whether the bond potential curve is symmetric (Hook's law) or asymmetric (Lennard-Jones, ionic, Mie ...). All forces start at zero an increase as we pull on the bond. The distinction in an asymmetrical potential is that molecules move further apart as temperature increases. So, at finite temperature, the initial force between molecules in an asymmetric bond potential is attractive (while in a Hook’s law bond potential the initial force between molecules at any temperature is always zero).

• Surface tension can also be explained by at least two other approaches. One is to integrate the difference between perpendicular and lateral pressures going from the bulk liquid to the bulk gas. The other is to apply first principles to the combined laws in thermodynamics (e.g. $dU, dH, dA, dG$) to include surface tension $\gamma dA$ as an equivalent to $\mu_j dn_j$ (chemical potential). Again, we have no need to know the position for the surface plane .... or the thickness for the actual surface plane.

$\Rightarrow$ The above statements tell us that molecules in a real substance at finite temperature are further apart than the equilibrium lowest potential energy distance because the bond potential is asymmetric. This is true throughout the bulk and surface. The arguments give us no reason to agree on forming a surface "skin" layer with molecules more closely packed.

• Forces that act solely perpendicular to a plane do not cause molecules in that plane to pack closer together or move further apart laterally within that plane.

• The net interaction force on all molecules in a liquid surface plane is an attractive force perpendicular to the plane that pulls the surface plane closer toward the bulk liquid planes below it.

• The next plane below the surface planes has no net force that should pull that plane or molecules in that plane up to the surface.

$\Rightarrow$ These statements tell us that molecules in a surface plane for a liquid are never induced to move closer or further apart laterally within the surface plane solely due to unbalanced perpendicular forces between the bulk liquid plane below and the empty gas plane above. We can also reason that to create a plane where molecules are more densely packed laterally will require external forces (e.g. the equivalent of $pV$ work in a bulk) and is entropically disfavored (will not happen spontaneously without applying such external work). The second and third statements tell us that the surface plane is closer to the bulk layer below it.

Summary

What do we know? Asymmetric bond potentials predict that molecules are further apart throughout the entire bulk and surface. All molecules in the bulk and surface are therefore on average under a net attractive force that is countered by the molecular kinetic energy at finite temperatures. The internal forces do not cause changes to the molecular structure (packing density) laterally in the plane. Finally, we anticipate that liquid molecules are organized at the surface plane in the same lateral area number density as in the bulk liquid but that the surface plane is spaced more closely to the bulk layer below it than what is typically found for spacings between layers in the bulk liquid (in solids, this phenomena is called relaxation).

Reality

Let's make the surface plane have a finite thickness. What happens? The molecules in the surface plane are now free to move individually closer to (or further from) the bulk layer below. And they do so in dynamic equilibrium. This individual movement gives rise to changes in density distribution as we move perpendicular to the surface going from the bulk liquid to the bulk vapor.

Start instead from the vapor phase. Stand on an infinitesimally thin plane. Assume the vapor is a vacuum. Click your fingers and stop all molecular motion in the system. Move the plane to go toward the bulk liquid. At some point, we will start to see a few molecules appear on our plane. We associate those molecules with the "surface" liquid. Keep moving the plane to the bulk liquid. We see more molecules. As we continue to move the plane, we may momentarily see more molecules per area than we eventually see in the bulk. Why? Because we reach a point where some of the molecules from what would be the entire surface are sitting deeper but not fully deep into what will be the bulk liquid and perhaps also because our plane is infinitesimally thin.

Let's make the plane have a finite thickness in dynamic equilibrium with the layers below it and plot a density distribution (mass per unit volume) moving from vapor to liquid. When we make the plane as thick as a molecule or perhaps somewhat thicker, we should eventually see a smooth density increase going from vapor to liquid. The shape and width of the transition will depend on the extent of the asymmetry in the bond potential and on the temperature.

Finally, let's induce or allow formation of a macroscopic curvature in the otherwise infinitely flat, planar liquid surface. In regions with macroscopic curvature, surface tension forces act as macroscopic stresses with vector components resolved inward (positive curvature) or outward (negative curvature) to the bulk liquid. This drives the need to incorporate surface curvature in phenomena such as the vapor pressures in drops.

For further reading, consult the references given in the original comments to your posting as well as this reference on molecular modeling for density distributions in surfaces of liquids. https://pubs.acs.org/doi/10.1021/la403421b.

Answer 5

The water molecules on the surface have a vast empty space to move in on one side. This causes them to move further apart than inside the bulk molecules. This larger separation causes a force between surface molecules that makes the surface act like an elastic container. Hence the droplets on a window. Introducing pollution in the water might decrease the forces playing between the surface molecules and let the droplets decay into shapeless blobs.