0
$\begingroup$

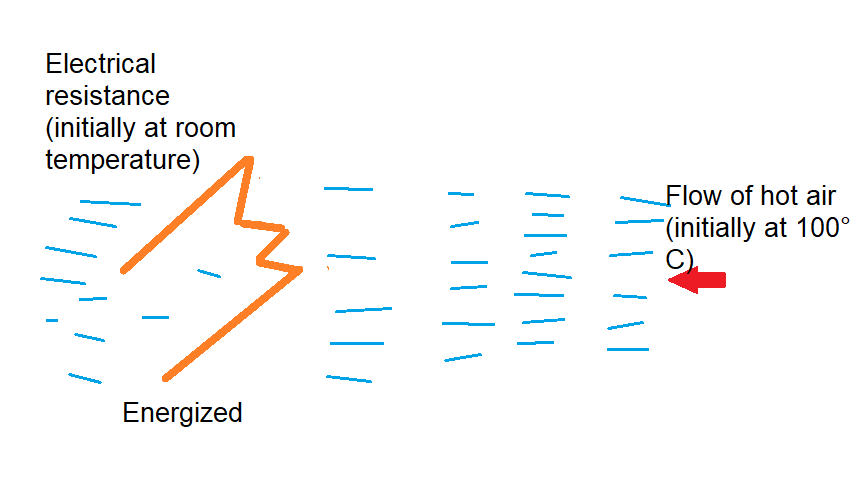
We know that electrical resistors give off heat due to the Joule effect. But what would happen if, at an instant of time t=0, I connect an electrical resistance (to a power socket) that is initially at room temperature, but I place it in the middle of a flow of hot air (that is, for example at 100°C).

enter image description here

We know that heat always flows from a body of higher temperature to a body of lower temperature. What would happen with the power dissipated by the Joule effect? Would it absorb or something else? I have that doubt. If you could please help me...seems like a simple question...that would be great. Thanks in advance.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ What would be the temperature of the electrical resistance with no hot air flow passing it? $\endgroup$
    – David White
    Commented Feb 21, 2022 at 17:35
  • $\begingroup$ I imagine that, without the flow of hot air, the temperature of the resistance would increase from room temperature until it reaches an equilibrium temperature; this equilibrium would be due to the fact that the Joule effect would come into equilibrium with the losses due to convection. $\endgroup$
    – ilich qynn
    Commented Feb 21, 2022 at 17:43
  • 1
    $\begingroup$ @ilichqynn, yes, that's what would happen. But you can choose the resistance of the heating element, its diameter and length, etc, to choose what that final temperature will be. What temperature do you want to choose? In particular, will it be more or less than 100 C? $\endgroup$
    – The Photon
    Commented Feb 21, 2022 at 17:45
  • $\begingroup$ @ThePhoton Good question. I made this post because I am currently working on controlling the temperature of a small oven. Different temperatures can be chosen, but all are greater than 100°C. The lowest can be 150°C. And the behavior that those resistors would have, I humbly described it above. $\endgroup$
    – ilich qynn
    Commented Feb 22, 2022 at 11:27

1 Answer 1

Reset to default
2
$\begingroup$

This is a fairly straightforward heat transfer problem. You have volumetric heat generation in the resistor and convective heat transfer at the surface of the resistor. Perform an energy balance. The Joule heating always contributes heat; convection adds heat if the resistor is colder than the air and removes heat if the resistor is hotter than the air. If the Biot number of the resistor is large, we can assume uniform temperature of the resistor and perform a simple lumped-component analysis to determine the transient temperature. (Otherwise, we have to consider temperature variations within the resistor.)

We know that heat always flows from a body of higher temperature to a body of lower temperature.

This is true for bodies at internal equilibrium. Note that a power supply is not at equilibrium (put another way, its thermodynamic temperature is greater than its actual temperature); thus, this rule cannot explain why a resistor plugged into a room-temperature power socket would ever grow hotter. A better broad strategy is to apply the heat equation to the resistor, as described above.

Does this get at what you're asking about?

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.