1
$\begingroup$

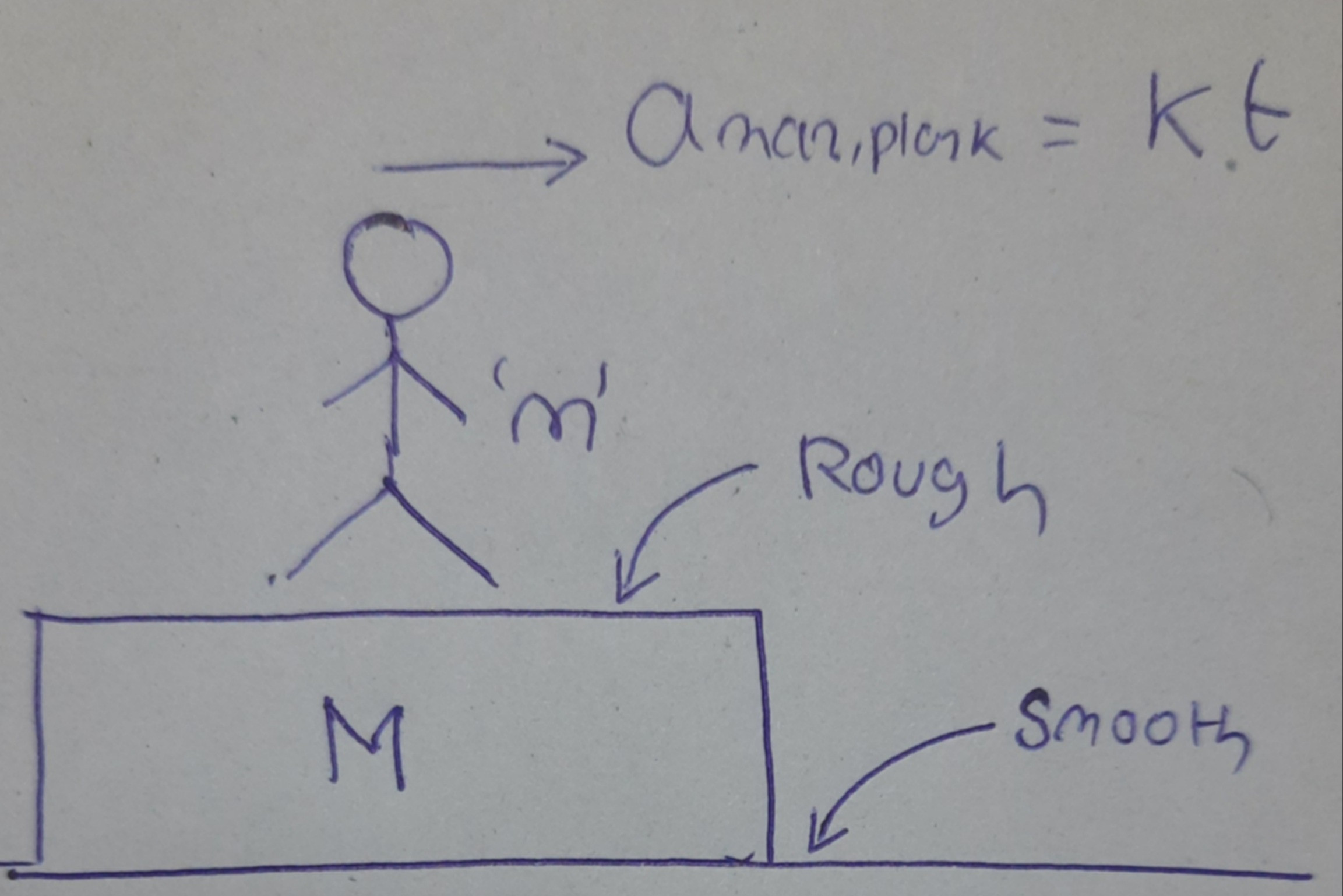
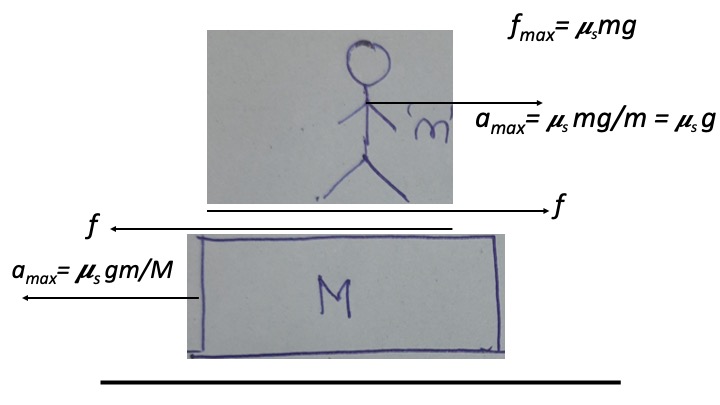
enter image description here

A man of mass $m$ is standing on a plank of mass $M$. There is no friction between the plank and the ground but there is friction between ground and man. The man starts with an acceleration $a=kt$ relative to the plank. The question says that there is no slipping between the man's feet and the plank then what is the work done by the friction on man as a function of time? My question is how can there be no slipping and relative acceleration between the man and the plank at the same time?

EDIT: I found Bob D's answer helpful and explanatory. Now my 2nd point of confusion. In the question since there is no external force i thought in horizontal direction the center of mass will be stationary.So if wrt ground the man moves rightwards then plank should move leftwards. But in the answer it says both man and plank would be moving leftwards wrt ground. How is this possible?

Improve this question
$\endgroup$
2
  • $\begingroup$ Sorry, can you make a diagram to show what is happening? I am having difficulty following your explanation. The man is on the plank, has no friction with the plank, but does have with the ground? Please make that a little more clear. By the way, if you make the diagram I am asking for, in many such cases, you are more than half way to getting the answer yourself. $\endgroup$
    – Dan
    Commented Feb 10, 2022 at 19:29
  • 1
    $\begingroup$ @Dan image uploaded $\endgroup$
    – Arpan
    Commented Feb 10, 2022 at 19:55

3 Answers 3

Reset to default
1
$\begingroup$

My question is how can there be no slipping and relative acceleration between the man and the plank at the same time?

No "slipping" simply means that the maximum possible static friction force between the man's feet and the plank is not exceeded so that the mans feet do not slide on the plank. It does not mean there is no relative motion between the man and the plank. Just that the relative motion does not involve slipping. It's like when a car attempts to accelerate too quickly, the drive wheels lose their grip and start to skid on the road.

The reason for stipulating no slipping is that the accelerations of both the man and plank are due to the equal and opposite static friction forces each exerts upon the other per Newton's third law. The work done by the plank on the man (and vice versa) is due to static friction. If slipping were to occur, then static friction becomes kinetic friction which which can only do negative work dissipating energy in the form of heat.

The free body diagrams of the horizontal forces on the man and plank below show the maximum possible static friction forces, and thus maximum possible accelerations relative to the ground of both the man and plank.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$
1
$\begingroup$

You know the equation for work: $W = Fd$.

When you watch someone run from the ground frame, the point of contact on the ground is at rest. Since it is at rest, there is no displacement during the force and work done is zero.

But the plank in the scenario is not at rest. The force from the plank is displaced in the frame of reference. Since you have both force and displacement, you have work being done.

The fact that there is "no slipping" between the man and the plank means that there are no frictional losses you have to worry about. All of the force of friction goes into acceleration. None goes into heat loss.

When you take a step forward on the ground, normally your feet do not slip, but you still gain forward velocity (acceleration). This shouldn't be remarkable.

Improve this answer
$\endgroup$
0
$\begingroup$

how can there be no slipping and relative acceleration between the man and the plank at the same time?

The man is not a rigid body. He is a deformable object. So there can be relative motion of the center of mass even while there is no relative motion at the point of contact.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Can you plz elaborate $\endgroup$
    – Arpan
    Commented Feb 10, 2022 at 19:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.