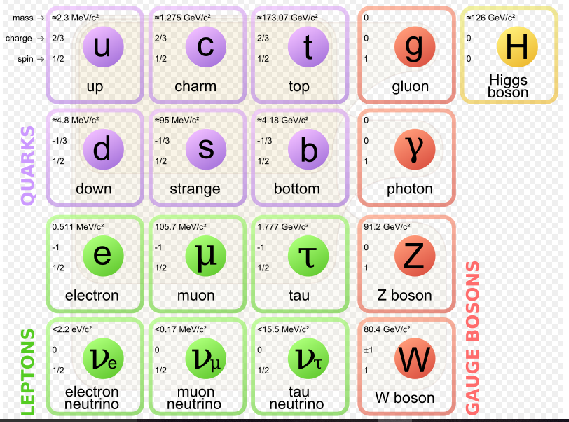
Basic question about the Standard Model: Is it accurate to say that all of the particles defined by the SM can be categorically distinguished entirely by discrete properties (eg, spin, color, charge units, interaction type, etc), as opposed to also requiring continuous properties (eg, rest mass, etc)? I'm wondering if the discrete/continuous property distinction somehow reflects a fundamental distinction between quantum and classical "objects".
-
1$\begingroup$ I don't think this is a well-defined question. Given a finite set of particles, we can just invent an operator that assigns 1 to the first particle, 2 to the second, etc. Then all these particle are "distinguished by a discrete property" (the number assigned to them), but that doesn't really tell us anything. Also, quantum mechanics is not about discreteness (see: position and momentum operators in QM). $\endgroup$– ACuriousMind ♦Commented Dec 4, 2021 at 20:02
-
$\begingroup$ @ACuriousMind Well quantum mechanics isn't only about discreteness but it is one of its distinguishing features. A classical harmonic oscillator can change its energy by any amount you want. A quantum HO can only change energy in discrete packets. A field theory can have arbitrary excitations while QFT has particles as smallest possible excitations. $\endgroup$– AccidentalTaylorExpansionCommented Dec 4, 2021 at 21:31
1 Answer
Is it accurate to say that all of the particles defined by the SM ...
The standard model is a quantum field theoretical model. The elementary particles of the standard model are axiomatic, they are not defined by the model, but constrain the model:
There is nothing continuous in the definitions in the table, the particles are also, for the model, point particles, they have no extent in space.
Over the years the table has been expanded as more and more particles were found necessary in order to fit the data, and as accelerators open up higher mass possibilities this will probably go on, as the theoretical model will be expanding to fit the newer data.
-
$\begingroup$ Perhaps my question is as much philosophical as physical. The table above seems to distinguish quarks by mass (which I was calling a continuous property) rather than only by charge or spin alone (discrete property values). Alternately, my physics text distinguishes all quarks & antiquarks in terms of only discrete properties: charge (+1/3,-1/3,+2/3,-2/3), baryon number (+1/3,-1/3), strangeness (+1,-1), charm (+1,-1), bottomness (+1,-1), and topness (+1,-1). These discrete properties seem able to classify all quark types, without reference to mass. But what about the rest of the table? $\endgroup$ Commented Dec 4, 2021 at 22:52
-
$\begingroup$ all attributes are attributed from experimental numbers. The symmetries seen in the system of hadronic resonances led to the quark model en.wikipedia.org/wiki/Quark_model and the strong interaction SU(3). SU(2) fitted the weak and u(1) the electromagnetic. en.wikipedia.org/wiki/Electroweak_interaction . That mass assigned for the particles comes from the field of continuous real numbers, but there is no continuity , i.e. possibility of having a different value. it is one off assigned from measurements. $\endgroup$– anna vCommented Dec 5, 2021 at 5:04
-
$\begingroup$ Thanks for the discussion anna, although we're not quite connecting. As a particular case, can you briefly clarify how physicists formally (ie, in terms of specific properties) distinguish between gluons and photons? Since (from the table) they both have the same mass, charge, and spin; there must be some other relevant distinguishing property which is part of the formal Standard Model. $\endgroup$ Commented Dec 5, 2021 at 17:32
-
1$\begingroup$ @davypough by the interactions they couple to. hyperphysics.phy-astr.gsu.edu/hbase/Forces/funfor.html To understand what is going on one has to understand Feynman diagrams . $\endgroup$– anna vCommented Dec 5, 2021 at 17:43
-
1$\begingroup$ @davypough conservation laws, lepton number, during interactions, quarks have baryon number conservation $\endgroup$– anna vCommented Dec 5, 2021 at 20:02