I believe the best way to visualize physically your question is in the case of the electromagnetic field of a current carrying wire conductor.
The explanation given must be fundamental and must include the dressed electron field of the bare electron's mass which is the origin and source of electromagnetism phenomenon. The discrete free electron drifting inside the wire, its electromagnetic flux envelope is cascaded with that of the other electrons inside and along the wire as illustrated below in fig.1:
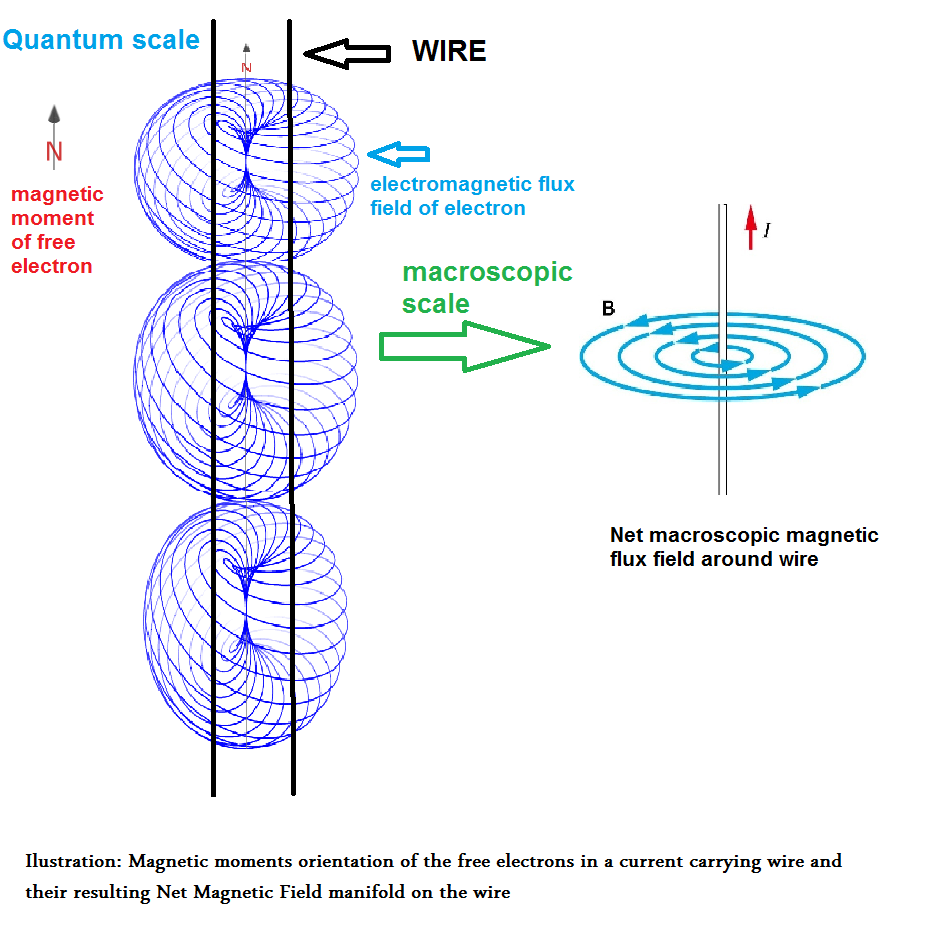
Important here is to understand shown in fig.1 that the single electron does not have separate electric and magnetic flux but a unified electromagnetic flux manifold. The electromagnetic quantum flux (i.e. EM flux of the electron quanta) of all the cascaded coherently electrons inside the current carrying wire generate the uniform macroscopic electric field inside along the wire E and magnetic B field envelope outside and along the wire. Both constitute the electromagnetic macroscopic field of the current carrying wire.
You can clearly see in the above illustration (on the right illustration of fig.1, conventional flow of current is used), that axially the electric field vector E inside the wire which is in the same direction of current I Poynting vector in the case of a current carrying wire (conventional flow of current is used), is perpendicular to the magnetic B field vectors outside the wire.
This perpendicular characteristic of the B and E components of the electromagnetic field travelling along a wire generating electric current is an inherent property of the dressed electron electromagnetic flux envelope.
On the left side of the fig.1 illustration we see the cascaded electron's field manifolds central electromagnetic flux (see horn tube flux formation diametrically on each electron manifold) clearly forming the E field inside the wire and the outside periphery electromagnetic flux of each electron manifold forming the B uniform macroscopic field outside the wire.
Notice also importantly, how the interchangeable between the electric and magnetic components nature of the electromagnetism phenomenon is demonstrated in fig.1?
The cascaded quantum flux of each electron manifold vertical segment (see diametrical horn tube formation of each manifold) which represents the magnetic moment of each electron becomes the macroscopic net electric field E inside the wire conductor and the periphery quantum flux of each electron manifold which represents the electron charge, becomes the macroscopic magnetic field B outside the wire.
The bare electron mass is positioned at the center of the dressed electron field manifold shown in fig.1, at a dimensionless point. Therefore the bare electron model is characterized by the known literature as an elementary dimensionless-point massive particle which has created in the past much confusion and non-intuitive understanding. The actual physical form of the electron particle is dressed meaning it has a charge radius.
This concludes IMO the physical explanation you particular asked in your question, besides the other more formal correct answers given.