5
$\begingroup$

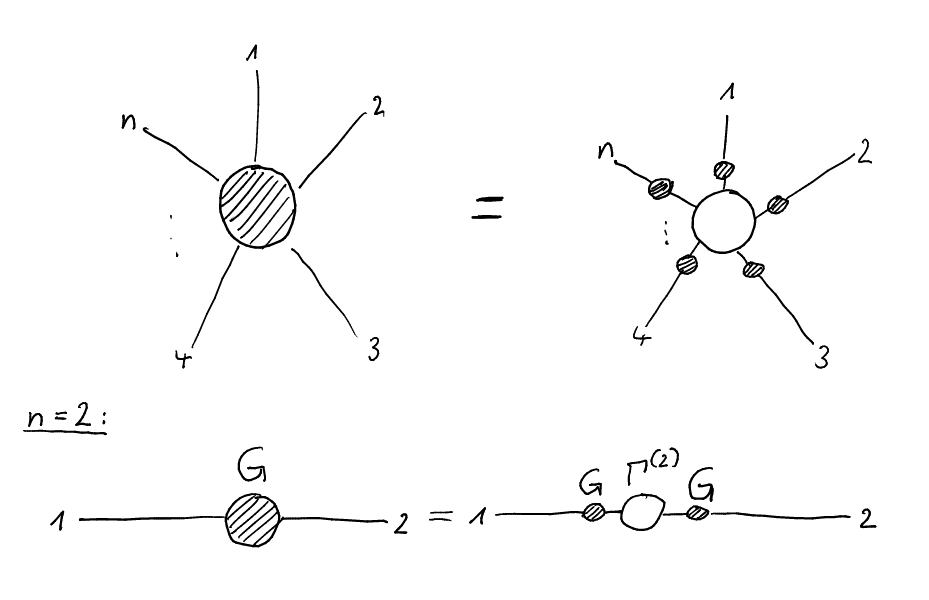
I know that the 2-vertex $\Gamma^{(2)}$ is the second derivative of the effective action, but I fail to see what it is diagrammatically: is it the truncated 1PI diagram? The non-truncated one?

If this helps, the trouble comes from the identity, in massless $\lambda\phi^4$ theory, that states $$\Gamma^{(2)}=G^{-1}=P^{-1}-\Sigma\tag{1}$$ where $G$ is the propagator, $P$ is the free propagator, and $\Sigma$ is the self-energy. I understand why $$G^{-1}=P^{-1}-\Sigma\tag{2}$$ (using the geometric series), but I fail to see what $$\Gamma^{(2)}=G^{-1}\tag{3}$$ means in terms of diagrams.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

The 1PI effective action $\Gamma[\phi]$ generates the 1PI connected amputated Green's functions. Let us denote the connected fully resummed non-amputated vertices by shaded circles, and the 1PI amputated vertices by empty circles.

Diagrammatically:First row: A shaded circle with n legs equals an empty circle with n legs which each have a shaded circle in their middle. Second row: A line with a shaded circle in its middle equals a line with a shaded circle, an empty circle and then a shaded circle in its middle

Note that the row for $n=2$ is $G = G\Gamma^{(2)}G$, which is the same as $\Gamma^{(2)} = G^{-1}$.

If you think about it, this is just drawing what amputated means: $\Gamma^{(n)}$ is the fully resummed vertex with all the fully resummed propagators $G$ removed from the legs.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ 6 months later, I see that I don't fully understand your answer. Our objects are $G$, $\Gamma^{(2)}$, $\Sigma$, and $P$. $P$ is the free propagator. $G=P+P\Sigma P+P\Sigma P\Sigma P + \dots$ is the full propagator, which means that $\Sigma$, the self-energy, is the sum of all 1PI amputated diagram. For now, no "inverse operator" was mentioned (and this, in my opinion, keeps things clear). Can $\Gamma^{(2)}$ be written as a series like $G$, without using inverse operators? If so, what are its first few terms? $\endgroup$
    – Mauro Giliberti
    Commented May 18, 2022 at 21:42
  • $\begingroup$ @MauroGiliberti Both your initial question and my answer have ignored a minus sign $\Gamma^{(2)} = - G^{-1}$ that becomes relevant if you want to understand what $\Gamma^{(2)}$'s diagrammatic expansion is. See this answer by Qmechanic for an explanation of the minus and chapter 7.8 of these notes for the proof that "$\Gamma^{(2)}$ is the sum of amputated 1PI diagrams at 1-loop and higher minus the tree-level propagator". $\endgroup$
    – ACuriousMind ♦
    Commented May 18, 2022 at 22:36
2
$\begingroup$

  1. OP is correct that the diagrammatic interpretation of the self-energy $\Sigma$ as (a sum of) amputated diagrams comes from the geometric series (2), cf. e.g. this Phys.SE post.

  2. OP is also correct that the diagrammatic interpretation of eq. (3) is less clear. Eq. (3) is inherit from the Legendre transformation$^1$ between the generator $W_c[J]$ of connected diagrams and the effective action $\Gamma[\phi_{\rm cl}]$. Note that eq. (3) gets modified in the presence of tadpoles, cf. e.g. my related Phys.SE answer here.

References:

  1. S. Weinberg, Quantum Theory of Fields, Vol. 2, 1996; eq. (16.1.21).

--

$^1$ Eq. (3) often contains a minus, cf. e.g. Ref. 1.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.