-1
$\begingroup$

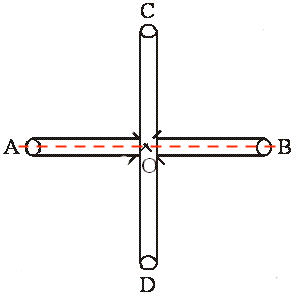
So I have two rods that are equal in mass and length. I join them together and form a cross. What is the moment of inertia of this object now in the $x$ axis?

So I attached an image, where the red line shows the axis of rotation. So at that axis, what is the moment of inertia of the cross?

enter image description here

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Please show us what you've tried so far. $\endgroup$
    – Gert
    Commented Nov 2, 2021 at 18:24
  • $\begingroup$ @Gert I do not even know from where to start actually $\endgroup$
    – Blue Green
    Commented Nov 2, 2021 at 18:32
  • $\begingroup$ The MMOI of the rod along the axis is ZERO so you are left with the MMOI of a rod about its center, the most basic of calculations, $\frac{m}{12} \ell^2$. $\endgroup$
    – John Alexiou
    Commented Nov 3, 2021 at 2:58

1 Answer 1

Reset to default
1
$\begingroup$

If your rod is treated as very thin, or radius of cross-section is :$r\rightarrow0$, then rod along x-axis isn't really hindering (providing inertia) to rotational motion. So here only moment of inertia of other rod about centre will be counted.

Now if your rod is treatable as cylinders, then you would have to individually find moment of inertias of both cylinders about your x-axis (calculus will be required), and then add them to get final moment of inertia

Improve this answer
$\endgroup$
12
  • $\begingroup$ Hi! Thank you so much!! $\endgroup$
    – Blue Green
    Commented Nov 2, 2021 at 18:31
  • $\begingroup$ Welcome! If you are new, you can upvote answers/questions which you find useful, and can select answers which you think are best by clicking tick button. $\endgroup$
    – Kshitij Kumar
    Commented Nov 2, 2021 at 18:35
  • $\begingroup$ So I know that the inertia of a rod about center is simply 1/12 ml^2. Also, the inertia of a rod around axis is 1/2 ml^2. So does this mean that the inertia of the whole thing would be the inertias added? Check this out for what I mean: cdn.kastatic.org/ka-perseus-images/… $\endgroup$
    – Blue Green
    Commented Nov 2, 2021 at 18:37
  • $\begingroup$ What do you mean by 'around axis'? $\endgroup$
    – Kshitij Kumar
    Commented Nov 2, 2021 at 18:38
  • $\begingroup$ So around the x-axis. Please check out the image that I linked :) $\endgroup$
    – Blue Green
    Commented Nov 2, 2021 at 18:39

Not the answer you're looking for? Browse other questions tagged or ask your own question.