Are current loop and magnets 100% equivalent?

Question

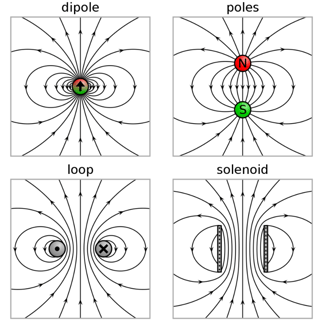
let's consider this picture taken from Wikipedia,

It is written that:

A magnetic dipole is the limit of either a closed loop of electric current or a pair of poles as the size of the source is reduced to zero while keeping the magnetic moment constant. It is a magnetic analogue of the electric dipole, but the analogy is not perfect. In particular, a true magnetic monopole, the magnetic analogue of an electric charge, has never been observed in nature.

and then

Because magnetic monopoles do not exist, the magnetic field at a large distance from any static magnetic source looks like the field of a dipole with the same dipole moment.

Now I have some basic doubts about the sentences in bold:

1. It is not clear to me how can the magnetic moment be the same as the size of the source becomes zero (which would mean the couple of poles becomes like a permanent magnet, where the North and South poles are inseparable, as shown in the highest picture on the left). As written there, the magnetic moment of a magnet can be defined as the product of the magnetic pole strength p and the distance l between the poles. To keep the magnetic moment constant and finite the the pole strength must be infinite. What does it mean physically?

2. What does it mean that the magnetic field generated from any of the four previous sources looks like the same at high distance? I understand that the fieldlines, apart from the inner region of the source, looks like the same. But, is it just a similarity of fieldlines, or, given one of those sources, is it possible to generate the same magnetic vector field in space (outside the inner region of the source) with one of the other sources if properly sized? Briefly: is it possible to generate the magnetic field vector of a magnet with a current loop in a 100% exact way?

Answer 1

For the physical interpretation of the limit: imagine you had a current loop whose size you could decrease easily, like pulling on a drawstring. If you just make it smaller, you decrease the magnetic moment $\mu = IA$; to keep the field the same, you'd have to increase the current. If you cut the area $A$ of your loop in half, but doubled the current $I$, you'd have the same magnetic field far from the loop.

The ideal dipole source has zero size and infinite current, and the ideal dipole field is therefore infinitely strong at the origin. That's annoying. But we have the same problem with the monopole field, like the electric field from a point charge, which is proportional to $1/r^2$ and is infinite at the origin. For the electric field we get around this by inventing quantum mechanics and discovering that a "point charge" is not actually a thing that exists. The proton has a finite size; while the electron is a structureless "point particle," for computing its electric field you actually care about the finite charge density described by its wavefunction.

I might rephrase your second question as "what do we mean by 'large distance'?" Suppose you have a real cylindrical solenoid, made out of wires, with length $L$ and radius $R$. The dipole approximation is only good if your distance $r$ from the solenoid is much larger than $L$ or $R$. If you're inside the core of the solenoid you see a uniform field; if you are a tiny gnat tunneling through the wall you might prefer to treat the local field as due to a locally-flat sheet of current. The local field is complicated.

For a complicated field, it's helpful to describe it using a multipole expansion. I've already hinted at this by reminding you about the monopole field, which is produced by a point charge, and gets weaker like $1/r^2$ as you move away. If two opposite-sign charges are near each other, their monopole fields approximately cancel, and most of what's left over is described by the dipole field. The dipole field gets weaker with distance like $1/r^3$ — that's sort of what we mean when we say that the monopole fields approximately cancel out. The dipole field also has the more complicated shape, which leaks information about the orientation of the charges at the source.

Two back-to-back dipoles also approximately cancel out. What's left there is called a "quadrupole field," which gets weaker like $1/r^4$ and has an even more complicated shape than the dipole field. There's an infinite series of these higher-order corrections, which get weaker more rapidly as you move away.

A current loop with radius $R$ produces a magnetic field with nonzero dipole moment, but also nonzero quadrupole, octupole, hexadecapole, etc. moments, all of which are parameterized by $R$. If you move from $r$ to $2r$, the dipole field gets weaker by a factor of $2^3=8$, but the quadrupole field gets weaker by $2^4=16$. If you move many $R$ away (or equivalently, rebuild your current loop so that $R$ is very small), eventually only the dipole shape of the field will be measurable.