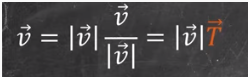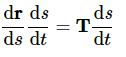0
$\begingroup$

When trying to find the tangential velocity, many textbooks define the tangent direction as one of the following:

enter image description here

or

enter image description here

Intuitively, why is the tangent vector the derivative of the position with respect to its modulus? Or the velocity with respect to its modulus? Why necessarily in the tangential direction?

Improve this question
$\endgroup$
3
  • $\begingroup$ What is dr and ds representing? $\endgroup$
    – The Space Guy
    Commented Sep 23, 2021 at 8:19
  • $\begingroup$ @TheSpaceGuy dr is the infinitesimal variation of the position vector and ds the infinitesimal variation of the position vector's modulus $\endgroup$
    – XXb8
    Commented Sep 23, 2021 at 8:20
  • $\begingroup$ Well, then dr/ds is representing unit vector along the tangent(direction of the motion). $\endgroup$
    – The Space Guy
    Commented Sep 23, 2021 at 8:24

1 Answer 1

Reset to default
1
$\begingroup$

Symbol $s$ is not the modulus of $\mathbf r$, it's the distance travelled. You can see that as $\Delta s\to0$, then $|\Delta\mathbf r|\to \Delta s$ as well. Intuitelvely, it's because the arc of a sufficiently smooth curve can be better and better approximated with the line as you move ends together.

Since, $|\Delta\mathbf r|\to \Delta s$, then $|d\mathbf r/ds|=1$, in other words $\mathbf t = d\mathbf r/ds$ is a unit vector tangential to the curve

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ This makes sense. However can't there be motion where |\Delta\mathbf r|\ remains conctant while distance travelled changes? Wouldn't this make |\Delta\mathbf r| \ =0 while the distance continues to change? $\endgroup$
    – XXb8
    Commented Sep 24, 2021 at 7:21
  • $\begingroup$ Also could you further explain how the line segment on the graph relate to your answer? $\endgroup$
    – XXb8
    Commented Sep 24, 2021 at 7:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.