Magnetic Charge through conducting loop

Question

There's a problem in Griffith's book that I don't understand his solution :

Here's the solution :

What's the justification for $\varepsilon = -L\frac{\mathrm{d} I}{\mathrm{d} t}$ ? $$$$ This equation comes from the Biot-Savart law which obviously does not hold in a world with magnetic charge. I do understand the justification of this equation in a quasi-static situation but in this problem - the divergence of the magnetic field is obviously not zero everywhere and we can't really reconstruct the Biot-Savart law from the modified Maxwell's equations (including magnetic charge)...

Answer 1

It's the definition of inductance, from here

Inductance

"Inductance is defined as the ratio of the induced voltage to the rate of change of current causing it"

Answer 2

I definitely share your unease at this derivation. Let's start with the field configuration. A stationary monopole is surrounded by a spherical radial B field. The extended Faraday law quoted by Griffiths tells us that both rate of change of B and magnetic current are associated with curl E, in exactly the same way as curl B for an electric charge, except for the sign of the induced E field. Thus a moving monopole has in addition an E-field circulating around the line of motion, in the opposite sense to a B-field around a moving electric charge. If we integrate around a loop in free space in the vicinity of a moving monopole we get a line integral of E as in the equation derived by Griffiths.

However, if the integration loop lies in a resistanceless metal then the resistanceless condition tells us that the loop integral of E must be zero at all times. The electric field must vanish inside the metal. Electric fields with zero curl can be cancelled out by electrostatic fields created by charges on the surface of the wire, but this can't work for this field because it is not irrotational. So in Griffiths equation $\cal E$ must always be zero.

If we write the magnetic current as the rate of change of charge passing through the loop $q_m$ then the remaining two terms in Griffith's equation can be integrated: $$ \mu_0q_m+\phi=\hbox{constant} $$ Suppose the monopole passes through the loop at $t=0$, Then at large negative times the constant is zero, and must remain zero. The $\phi$ of the monopole rises from zero to $\mu_0 q_m/2$ at $t=0$. We then get at step of $\mu_0 q_m$, while the linked flux suddenly changes to $-\mu_0q_m/2$. The whole expression is thus continuous, and as $t$ increases it rises to $\mu_0 q_m$. BUT the total expression must be zero, so there must be an additional flux linkage due to the induced current in the wire of (eventually) $\mu_0 q_m/2$. This is created by a current $I$ given by the Griffiths expression $$ I=\frac{\mu_0q_m}{L} $$ using the definition of $L$ as $\phi=LI$. I leave it to the reader to decide if this incorporates the same physics as Griffiths answer.

Answer 3

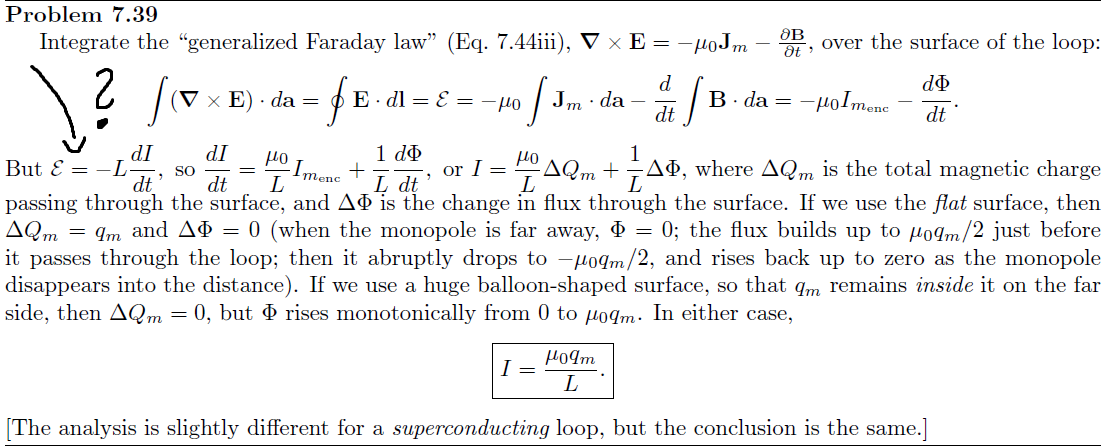
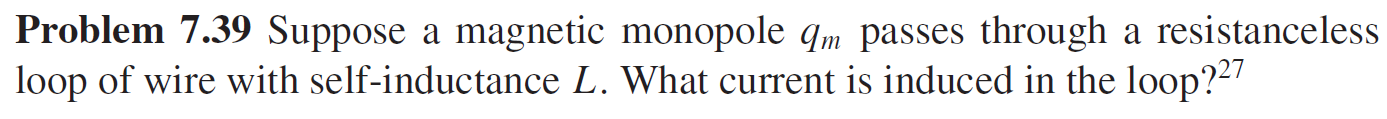If you want a more "foundational" derivation, you can start from Maxwell's third equation:

$$ \nabla \times \mathbf{E} = -\frac{\partial \mathbf{B}}{\partial t} \quad \Longleftrightarrow \quad \oint \mathbf{E}\cdot\mathrm{d}\boldsymbol{\ell} = -\frac{\mathrm{d} \Phi_{\mathbf{B}}}{\mathrm{d}t}, $$ where $\Phi_{\mathbf{B}} = \int\int \mathbf{B}\cdot \mathrm{d}\mathbf{S}$ is the magnetic flux.

Because $\mathbf{E}=-\nabla V$, $V$ being the potential: $$\oint \mathbf{E}\cdot\mathrm{d}\boldsymbol{\ell} = \sum_{\mathrm{whole\,\,circuit}} V.$$

In electrostatics, $\mathrm{d}/\mathrm{d}t = 0$, so $$ \oint \mathbf{E}\cdot\mathrm{d}\boldsymbol{\ell} = \sum_{\mathrm{whole\,\,circuit}} V =0,$$ which is Kirchhoff's Voltage Law.

In electrodynamics, $\mathrm{d}/\mathrm{d}t \neq 0$ so: $$ \oint \mathbf{E}\cdot\mathrm{d}\boldsymbol{\ell} = \sum_{\mathrm{whole\,\,circuit}} V = -\frac{\mathrm{d} \Phi_{\mathbf{B}}}{\mathrm{d}t},$$ where you would call the net effective voltage $V = \mathcal{E}$ the electromotive force:

$$ \mathcal{E} = -\frac{\mathrm{d} \Phi_{\mathbf{B}}}{\mathrm{d}t}. $$ The minus sign is referred to as Lenz's law.

Finally, in the same way as one defined capacitance $C$ and $C = Q/V$, one can also define inductance as $L = \Phi / I$. That is because both $C$ and $L$ just capture the geometry of the system, while the sources (charge $Q$ and current $I$) store energy in electric (potential $V$) or magnetic (flux $\Phi$) fields.

So:

$$ \mathcal{E} = -L\frac{\mathrm{d} I}{\mathrm{d}t}.$$