Can someone explain what this picture is about please?

Question

From https://www.feynmanlectures.caltech.edu/I_51.html

just to illustrate a point, we will try to analyze the speed of such a so-called bore, in a channel. The point here is not that this is of any basic importance for our purposes—it is not a great generalization—it is only to illustrate that the laws of mechanics that we already know are capable of explaining the phenomenon.

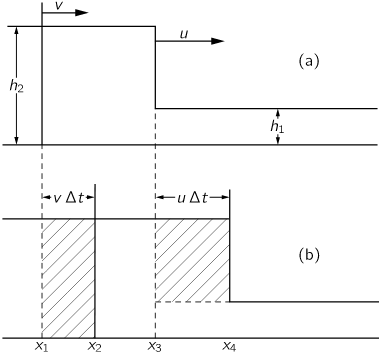
Imagine, for a moment, that the water does look something like Fig. 51–5(a), that water at the higher height $h_2$ is moving with a velocity $v$, and that the front is moving with velocity u into undisturbed water which is at height h1. We would like to determine the speed at which the front moves. In a time $\Delta t$ a vertical plane initially at $x_1$ moves a distance $v\Delta t$ to $x_2$, while the front of the wave has moved $u\Delta t$.

I can't understand a single thing about this picture.

1. What do $h_1$ and $h_2$ represent?
2. What does the shaded area represent?
3. What do the non-shaded areas represent?

Answer 1

Quoting from the very same link you posted at the beginning of the question:

1: What do h1 and h2 represent?

Heights.

[...] that water at the higher height $h_2$ is moving with a velocity v, and that the front is moving with velocity u into undisturbed water which is at height $h_1$.

2: What does the shaded area represent?

Volumes of water that have moved/displaced, i.e. that were not previously in that place.

[...] we see that the amount $h_2vΔt$ of matter that has moved past $x_1$ (shown shaded) is compensated by the other shaded region, which amounts to $(h_2−h_1)uΔt$.

3: What do the non-shaded areas represent?

Water that has not been displaced.

Answer 2

h1 is the height in front of the front, in the direction of the front's travel

h2 is the height behind the front

The shaded area is the area of water that has moved past point x1 in time $\Delta{}t$

Non-shaded areas show where the water is. Shaded areas show how 'where the water is' has changed.

The point here is that the water between x1 and x3 has changed shape and location. It now occupies $h_{2}v\Delta{}t$ less area on the left, and $(h_{2}-h_{1})u\Delta{}t$ more area on the right. But it's the same amount of water, so the two should be equal.