1
$\begingroup$

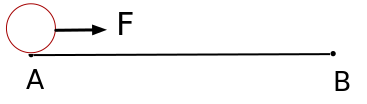
Consider the following diagram:

enter image description here

The force $\mathbf{F} = 1 \textrm{ N} \hat{\imath}$ is being applied all time as the ball goes from A to B (assume positive $x$ to the right.) Now, there are a few equivalent definitions for 'conservative force', e.g. a force whose work is $0$ for any closed path. Let me use this definition.

It is clear that if the ball went from $A$ to $B$ and then back from $B$ to $A$, the work done by $\mathbf{F}$ is 0. Of course this is only one possible closed path. We could also think of a triangular path $ABC$ with $\mathbf{r}_C = 0.5(\mathbf{r}_A + \mathbf{r}_B) + 1 \textrm{m } \hat{\jmath}$, and the same reasoning will apply, provided that $\mathbf{F}$ stays the same all along this path.

Essentially I am saying that if $\mathbf{F}$ is a constant field, then it is conservative, no big deal. This could be a Coulomb field $\mathbf{E}$ by two infinite charged planes and the ball a charge between them.

The thing is, if $\mathbf{F}$ is actually a pulling force (by someone's hand) on a ball, then we always say this is a non-conservative force, but why? What is the difference between $\mathbf{F}$ and $\mathbf{E}$ with respect to the definition I have used, that makes one conservative and the other non-conservative?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
2
$\begingroup$

A constant force is an example of a conservative force (weight is also a constant force). When you pull with your hand, what are the odds that you are going to be able to maintain the same level of force? The force applied will have a ramp-up period where it is not constant.

Taking the block-wire as one system, we can exploit conservation of energy to solve this problem:

enter image description here

Here is a relevant excerpt from O'Reilly (Intermediate Dynamics for Engineers):

enter image description here

Improve this answer
$\endgroup$
9
  • $\begingroup$ If it was actually an ideal constant force done by my hand, as often appears in beginner's excercises, would you say that it is conservative? $\endgroup$
    – Javi
    Commented Aug 6, 2021 at 4:25
  • 1
    $\begingroup$ It doesn’t fail. Since a constant force is conservative, it has an associated potential energy. The total energy of the system then is conserved. That’s kinetic plus potential. $\endgroup$
    – Evan
    Commented Aug 6, 2021 at 5:07
  • 1
    $\begingroup$ By the way, this is a very common method in pulley problems where a constant force is applied at one end of a cable. $\endgroup$
    – Evan
    Commented Aug 6, 2021 at 5:08
  • 1
    $\begingroup$ I can try and find you one tomorrow. If you can get the text Engineering Dynamics by Meriam Kraige and Bolton you can find some examples in there for pulley systems where they exploit conservation of energy in the presence of a constant load. $\endgroup$
    – Evan
    Commented Aug 6, 2021 at 5:34
  • 1
    $\begingroup$ As promised, see my edit. $\endgroup$
    – Evan
    Commented Aug 6, 2021 at 21:41
0
$\begingroup$

Recall that a force $\bf\vec F$ is conservative iff for any closed path $C$, $\displaystyle\oint_C\mathbf{\vec F}\cdot\mathrm d\mathbf{\vec r}=0$. The key word here is any, and you can use your hand to move an object in a closed path by accelerating its speed, such that $\mathrm{KE}_i\neq\mathrm{KE}_f$. So, $\bf\vec F$ cannot be conservative. There is no associated potential $U$ such that $\mathbf{\vec F}=-\nabla U$ since a pull force does not depend on position, but rather depends on how you choose to pull.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I understand that the work must be zero for any path and I thought I was clear about this. I don't understand your argument why $\mathbf{F}$ cannot be conservative: of course that if it moves in a closed path there must be another force that makes the ball turn around, but that doesn't mean I can't compute the work done by $\mathbf{F}$ alone, which is zero. On the other hand, arguing that there is no potential for $\mathbf {F} $ is kind of cheating since that is equivalent to saying that it is not conservative. $\endgroup$
    – Javi
    Commented Aug 6, 2021 at 4:47
  • $\begingroup$ @Javi if we start from the "any path," then to show that the force is not conservative, all that's needed is to find one such path where the work by the force on a closed loop is non zero $\endgroup$
    – user256872
    Commented Aug 6, 2021 at 5:10
  • $\begingroup$ if you take your block and accelerate it over a closed loop, then the work you do on the box is non zero. so your force is not conservative. or consider path independence: suppose you lift a box vs. lifting a box then dropping it then lifting it again. you do much more work during the latter process. start/end points are the same, but work differs according to the path. so, in either case, you see that your force is not conservative. hope this helps @javi $\endgroup$
    – user256872
    Commented Aug 6, 2021 at 5:14
  • $\begingroup$ thx for you reply. It seems to me that you are not taking into account the fact the $\mathbf {F} $ is constant, therefore if the ball moves around any closed loop the work will be indeed zero. The lifting example is different because when you drop the box you are not applying any force during the fall. In my case, the force $\mathbf {F} $ is being constantly applied, even when the ball loops around in any path. After all I guess this is a conservative force. $\endgroup$
    – Javi
    Commented Aug 6, 2021 at 5:28
  • 1
    $\begingroup$ well, if you manage to construct an applied force that is always constant then yes, it would be conservative (it would act just like a uniform electric field, for instance). however, when we talk about pulling forces (especially those provided by humans), we can't really assume that the force is always constant. @Javi $\endgroup$
    – user256872
    Commented Aug 6, 2021 at 5:36
-1
$\begingroup$

You need to make a distinction between work done by the overall system vs. work done on the ball. When the ball moves from A to B and then back to A (regardless of the path taken) the work done by that system is zero. That is, the ball is exactly where it started and therefore no work was done. However, when you look only at the ball, work is done in moving it. If you were to pull it from A to B and then back to A you would feel as if you had done some work. However, even in this case the work done by the system (not by you personally as the one pulling the ball) will be zero and by your definition it too will be a conservative force.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I don't get your point. What is the 'overall system' you are defining? What do you mean 'feel some work'? $\endgroup$
    – Javi
    Commented Aug 6, 2021 at 4:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.