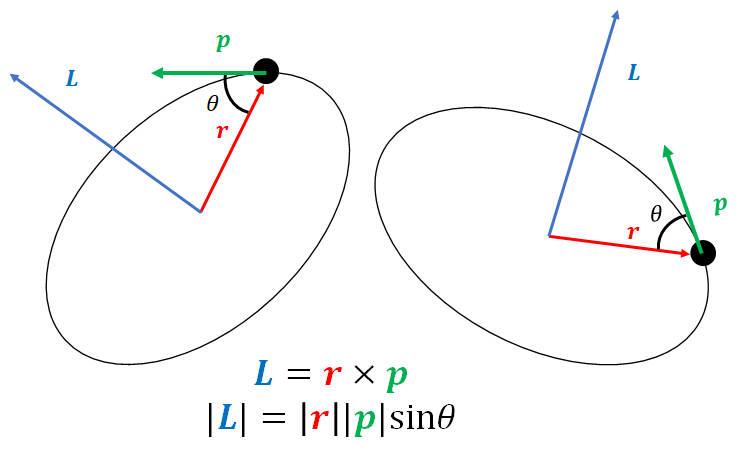Why is angular momentum a vector?

Question

I have read a bit about this and am still wondering why we actually care about the direction of angular momentum, because that the vector representing angular momentum is perpendicular to the momentum vector and the position vector doesn't really have any deeper meaning. Is it defined as a vector to keep it consistent with the definition of linear momentum or what is the deeper explanation behind this?

Answer 1

This answer elaborates on the answer-in-a-comment by Chiral Anomaly.

The precursor to the concept of angular momentum was Kepler's law of areas.

As we know, to define an area - using vectors as elements - you need two vectors.

As pointed out by Stack Exchange contributor Chiral Anomaly, if the motion is in a space with 4 spatial dimensions, or 5, or any higher number of dimensions then the only way to specify angular momentum at all is with two vectors.

A space with three spatial dimensions has a property unique to space-with-three-spatial-dimensions: every plane has a single direction that is perpendicular to that plane.

So: the convention of using a single vector to represent angular momentum is a hack, a hack that only works in a space with three spatial dimensions.

The convention:
The direction of the angular momentum vector expresses the plane of rotation, and the magnitude of the vector represents the magnitude of the angular momentum.

There is a problem though: the direction of the rotation is ambiguous. This problem would not be there with a notation using two vectors, but when you use a single vector to represent angular momentum then there is not enough capacity to represent all the information that needs to be represented.

Because of that ambiguity there is an extra rule: the right hand rule. The right hand rule exists because the single vector notation for angular momentum is a hack. The single vector doesn't have the capacity to represent all the information that needs to be represented.

Answer 2

In physics nothing is a vector, really. This expression is just a shortcut to say: "this quantity behaves like a mathematical vector", or even better: "it is useful to model this quantity as a mathematical vector".

In the specific case of angular momentum: you have to keep in mind that angular momentum is defined through rotations, and rotations behave a lot like vectors: rotations have a magnitude (how fast the rotation is) a direction (the plane on which the rotations happens) and a verse (clockwise or anticlockwise). So makes sense, it's useful, to define rotations as vectors, and so makes sense to define angular momentum as a vector as well.

And in fact, through this definitions, we get a lot of simple and elegant formulations of physical laws.

Answer 3

At the highest level, you can just treat it as a simple, mathematically compatible way to keep track of the handedness of the rotation. After all, it's a easiest to handle "lines" and it's easier to handle one "line" than a bunch of tangential "lines" which make up a "circle".

The direction along line and a circle both only have two opposite degrees of freedom and just happen to work. Math is like that sometimes. You can transform things so they are not directly comparable to the real thing but still mathematically equivalent. The closest simplest description is that the vector is the axis of rotation but the fact that the direction that it points in corresponds to direction around which the axis rotates is just something that happens to work out mathematically, and if explainable probably requires delving into some crazy math. Not unlike how positive is inherently opposite of negative and you can assign physical meaning to each polarity however you like (i.e. we screwed up with the negative charge of electrons but the math doesn't care or how you can orient the positive and negative directions when placing a Cartesian plane for your mechanics problem however you want).

Technically, it's a kind of model. It's just that math can be very abstract and we humans don't have much intuition for it so we don't take things for granted and end up noticing peculiarities like this. But this exists in all models. The only difference is that the more intuition and experience we have with something, the more we take it for granted and the less we notice peculiarities in the model. A bit like how we tend not to think the same thing about the Pythagoras Thereom because can just pull out a tape measure and measure the sides of something. If measurement tools that were like a big rod on a rotary encoder existed, which were used by pointing it to align it with the axis of rotation to measure rotation around that axis were more commonplace, it wouldn't feel so strange to us.

Answer 4

I note you say: "I have read a bit about this and am still wondering why we actually care about the direction of angular momentum, because that the vector representing angular momentum is perpendicular to the momentum vector and the position vector doesn't really have any deeper meaning."

Angular momentum is not, in general, perpendicular to momentum and position. This is the formula for individual point particles, and doesn't apply to collective systems of particles like rigid bodies, fluids, elastic bodies, or machines and mechanisms. A billiard ball can spin about an axis through the origin, and move through space along the same axis. It's momentum and angular momentum are aligned. The angular momentum of a multi-particle body is divided into internal and external components - the internal for motion relative to the centre of mass (with zero net internal momentum), the external for motion of the centre of mass. The external angular momentum is perpendicular to momentum and position, or zero if momentum is parallel to position, but the internal angular momentum added to it can be in any direction.

The point is that for central forces and for isolated systems, angular momentum is conserved - not just the magnitude, but also the direction. The position and momentum can change over time, but both magnitude and direction of angular momentum stay the same. It's this property that makes it interesting and useful, and we pay attention to the direction precisely because it being a constant helps us figure out the motion. It doesn't matter what forces individual parts of an isolated system exert on one another, the total magnitude and direction remains the same. We are thus able to simplify the properties of a system containing billions of individual particles, each with their own position and momentum, and represent it all as a body with a particular momentum and angular momentum, and compute its effect on other bodies.

Answer 5

The angular momentum possessed by a particle in some coordinate system is given by the cross product of the position vector, $r$ (vector from the origin to the particle), and the linear momentum of the particle, $p$. Based on the two systems in the diagram, even if the angular momenta have the same magnitude, the motion of both systems are very different since the conserved angular momenta point in different directions - the particles rotate about the origin of their coordinate systems differently on different trajectories.

By analogy, if you think about linear momentum, a particle moving upwards with a momentum of magnitude $p$ and a particle moving to the left with a momentum of magnitude $p$ have the same magnitude of momentum, but you'd be able to distinguish their motion as one is moving upwards and one is moving to the left.

In fact the vector nature of these quantities is very important in determining how they add. Just like how you add velocities or momenta as vectors, angular momenta can be added vectorially too. Phenomena like precession occur precisely because angular momentum is a vector - it has an associated direction and they have to be added up using the rules of vector addition.

Answer 6

The set of possible rotations about a single axis has three degrees of freedom, so a bijection can be established between this set and three-dimensional vectors. An obvious bijection is to associate a rotation about an axis with a vector pointing that direction, represent the magnitude of the rotation with the magnitude of the vector, and the direction of the rotation with the direction of the vector. This leaves open the question of which direction of the vector to associate with what direction of the rotation. Which association to use is arbitrary, but once you choose one, the direction of the vector is meaningful. Rotation in one direction is quite different from rotation in another.

While each possible rotation is represented by a vector, it isn't really a vector. If you add two rotations about the same axis, the resulting rotation is represented by the sum of the vectors that represent the constituent rotations, but that doesn't work for rotations about different axes. That is, if $v(r)$ is the vector that represent rotation $r$, then $v(r_1+r_2)=v(r_1)+v(r_2)$ is true if $r_1$ and $r_2$ are about the same axis. But if you have rotations about two different axes, the total motion is not rotation about the sum of the two axes. A more accurate term would be Clifford Algebra rather than vector space.

Answer 7

One more point: when you have radial forces in pairs between three or more bodies, individual angular momenta aren't conserved because angular momentum can transfer between bodies, but there is an overall conserved angular momentum. (Laplace showed this explains why planets orbit in roughly the same plane.) Obviously, we can't ignore the fact we're dealing with vectors when adding them up to get something conserved.

Let's spell it out. Suppose body $i$ has position $\vec{r}_i$ an momentum $\vec{r}_i$, and exerts a radial force $F_{ij}(\vec{r}_i-\vec{r}_j)$ on body $j$, so by Newton's third law $F_{ii}=0,\,F_{ij}=F_{ji}$. By Newton's second law,$$\frac{d}{dt}\sum_i\vec{r}_i\times \vec{p}_i=\sum_i\vec{r}_i\times\sum_jF_{ij}(\vec{r}_j-\vec{r}_i)=\sum_{ij}F_{ij}\vec{r}_i\times\vec{r}_j,$$where we don't need to manually ban $j=i$ terms because $F_{ii}=0$. Since $\vec{r}_i\times\vec{r}_j$ is antisymmetric, this sum is $0$.

Answer 8

Here's a late answer that you may need to file under lies to children but I used to tutor this concept and developed an explanation that helped students who were struggling with the switch from 2D angular momentum (and torque) to 3D. I know this isn't an educators site but please bare with me as I walk you through a thought experiment.

I started with what some found to be a shocking claim: In 3 dimensions there is no such thing as clockwise, without an observer.

To back up this claim I asked them to imagine I had two clocks standing upright on the desk between us. They have moving hands but no numbers. And you can see right through them. All you can really see are the hands. I rotated my fingers simulating the hands of the clocks and asked, "Which is rotating clockwise?".

Almost everyone pointed at the one that, to me, was rotating counter clockwise. I would then ask them to step over to my side of the desk and I'd make exactly the same motions.

"The truth is, they're both rotating clockwise, and counter clockwise, at the same time. Just depends on where you stand when you look at them".

"Now our task is to deal with that when we come up with a way to model this rotation." Then I'd show them something like this:

wikipedia - animated version

And I'd say, "This purple arrow here, the one that looks like it's pointing up, is telling you two things: How much torque and what direction to look at this thing from so it will seem to rotate clockwise. The observer here would by lying underneath and looking "up".

Now sure, we can go on about how that "up" isn't really "up" because this isn't really a spatial dimension, (for the magnitude at least). Or how this is a bivector like when you calculate area. But when students are at this level I simply say, "The magnitude here certainly isn't about anything physically being this distance away. It just looks like that because we decided to use this unused dimension for something else. In this case, torque."

Which was my sneaky way of letting them know everything they learned in 2D still works here in 3D. Once you're standing in the right place to look at it.

Answer 9

Angular momentum is about rotations the same way momentum is about translations. If I try to translate an object for a while by a applying a force it will generally acquire some momentum. Similarly if I try to rotate an object for a while by applying a torque it will generally have some angular momentum.

To describe rotations you need to know two things: the angle to rotate by and the axis to rotate around. Similar to how $$\text{momentum}=\text{rate of translation } \times\text{ how hard it is to change rate of translation}$$ we can say that $$\text{angular momentum}=\text{rate of rotation} \times\text{ how hard it is to change rate of rotation}$$ The rate of rotation has an axis and the direction of this axis can be nicely represented using a vector. Both the direction and magnitude have meaning so nothing is wasted.

Answer 10

This is a (multi)-linear algebra perspective as to why encoding angular velocities by vectors and cross-products is natural and not just some ad hoc, arbitrary convention.

Consider a rigid body rotating arbitrarily about the origin. We can encode its motion by a family of maps $A_t$, taking a vector $v$ (the initial position of a point in a body) as an input and outputting another vector $A_t(v)$ (the position of that point in a rotated body at time $t$). At each $t$, the map $A_t$ is linear, meaning that it respects addition of vectors and scaling: $A_t(\alpha v)=\alpha A_t(v)$ and $A_t(v+w)=A_t(v)+A_t(w)$; e. g., the latter follows from the fact $A_t$ just rigidly rotates a parallelogram $(0,v,w,v+w)$ to $(0,A_t(v),A_t(w),A_t(v+w))$ which thus must also be a parallelogram. Similarly, since $A_t$ rigidly moves a triangle $(0,v,w)$ to $(0,A_t(v),A_t(w))$, it also preserves scalar products: $A_t(v)\cdot A_t(w)=v \cdot w$ for any vectors $v,w$. Such linear maps are called orthogonal.

The angular velocity of the rotating body (say, at $t=0$) can be defined as the derivative of the map $A_t$: $$ A'|_{t=0}(v)=\lim_{t\to 0}\frac{A_t(v)-v}{t}. $$ The physical meaning of this is clear: $A'(v)$ is simply the velocity of the point $v$ (at $t=0$; I will henceforth drop it from notation). But note that since $A'$ is a limit of linear maps, it is also linear.

Can it be just any linear map? No. We can write $$ v\cdot w= A_t(v)\cdot A_t(w)=(v+tA'(v)+...)\cdot(w+tA'(w)+...)=v\cdot w+t(A'(v)\cdot w+A'(w)\cdot v)+\dots, $$ where dots denote terms negligible compared to $t$. Thus, we must have $$A'(v)\cdot w=-A'(w)\cdot v,\quad \forall v,w.$$

The linear maps with this property are called skew-symmetric (or skew-adjoint) linear maps, or bivectors. Notice that the bivectors themselves form a vector space, that is, they can be added and multiplied by scalars. E. g., if $A,B$ are two bivectors, then $A+B$ is a linear map defined by $(A+B)(v)=A(v)+B(v)$ for all $v$. This operation has a natural physical meaning. Let $A_t$ describe a rotation of the body with respect to a frame of reference which is itself rotating, and whose rotation is described by $B_t$. Then, the angular velocity of a point $v$ is given by \begin{multline} \lim_{t\to 0}\frac{A_t(B_t(v))-v}{t}=\lim_{t\to 0}\frac{A_t(B_t(v))-A_t(v)+A_t(v)-v}{t}\\ =\lim_{t\to 0} A_t\left(\frac{B_t(v)-v}{t}\right)+\lim_{t\to 0}\frac{A_t(v)-v}{t}=A_0(B'(v))+A'(v)=B'(v)+A'(v), \end{multline} quite similalry to the addition rule for usual velocities.

So far, we have never used that our space is three-dimensional. It turns out that by coincidence, in the 3D case, the bivectors also form a 3D space. Recall that a linear map $A$ from a vector space to itself can be uniquely described by its matrix: if $e_{1},e_{2},e_{3}$ is a standard basis, then any vector $v$ can be decomposed as $v=v^1e_1+v^2e_2+v^3e_3,$ and therefore $A(v)=v^1A(e_1)+v^2A(e_2)+v^3A(e^3)$. The vectors $A(e_i)$ in turn can be decomposed as $A(e_j)=a_{1j}e_1+a_{2j}e_2+a_{3j}e_3$; taking the scalar product with $e_i$, we get that $a_{ij}=e_i\cdot A(e_j)$. If we know the 9 numbers $a_{ij}$, we thus know the vectors $A(e_j)$ and the value of $A(v)$ on any vector $v$.

In the case of the bivector, we need just three numbers rather than $9$: indeed, by skew-symmetry, $a_{ij}=-a_{ji}$, hence $a_{11}=a_{22}=a_{33}=0$, and $a_{12}$, $a_{23}$, $a_{31}$ uniquely determine A (we could alternatively use $a_{13}$ but this one leads to more symmetric formulae). Let us compute \begin{multline} A(v)=v^1A(e_1)+v^2 A(e_2)+v^3A(e_3)=v^1(a_{21} e_2+a_{31}e_3)+v^2(a_{12} e_1 +a_{32}e_3)+v^3(a_{13} e_1 +a_{23}e_2)\\ =(v^2a_{12}-v^3 a_{31})e_1 - (v^1a_{12}-v^3 a_{23})e_2 + (v^1a_{31}-v^2 a_{23})e_3 =\hat{a}\times v, \end{multline} where $\hat{a}$ is a vector with coordinates $(a_{23},a_{31},a_{12})$! In fact, if you wish, we just invented the cross product from the first principles, by just looking at how the points in rotating rigid bodies move.

There's more to this story. The identification of a bivector with $A$ with a vector $\hat{a}$ goes through matrices and thus appear do depend on the choice of the orthogonal basis. But in fact, it only depends on the orientation of the basis, and can be defined without using coordinates at all. What's more, if $A,B$ are two bivectors, also $[A,B]=AB-BA$ is a bivector, and it so happens that the corresponding vector is given by $\hat{a}\times \hat{b}$. So, two different natural operations (one taking two bivectors and producing a bivector, and another taking a bivector and a vector and producing a vector) give rise to the cross product. This discussion becomes techincal, and I refer to this answer.