5
$\begingroup$

In Feynman Lectures on Physics, Volume III: Quantum Mechanics, Feynman says

"The uncertainty principle 'protects' quantum mechanics. Heisenberg recognized that if it were possible to measure the momentum and the position simultaneously with a greater accuracy, the quantum mechanics would collapse."

What is the logical paradox that we would arrive at if we could know both the momentum and position of an electron. (Specifically, in the dual slit experiment.)

Could we write a rough, informal proof by contradiction that the uncertainty principle is necessary?

Improve this question
$\endgroup$
1

2 Answers 2

Reset to default
6
$\begingroup$

Let $f(x)$ be a wave function, $P:f\mapsto f'$ the momentum operator and $X:f\mapsto xf$ the position operator. Then

$$(PX)f=f+xf'$$

$$(XP)f=xf'$$

Thus if $PX=XP$ (i.e. if the uncertainty principle fails) then $f=0$, so $f$ is not a wave function after all. Therefore there are no wave functions. I think that counts as a collapse of quantum mechanics.

Improve this answer
$\endgroup$
2
$\begingroup$

One way to look at it is the notion of distribution (Fourier analysis):

The more precisely an object is located in space, the closer its spatial distribution resembles a Delta distribution (a very narrow peak).

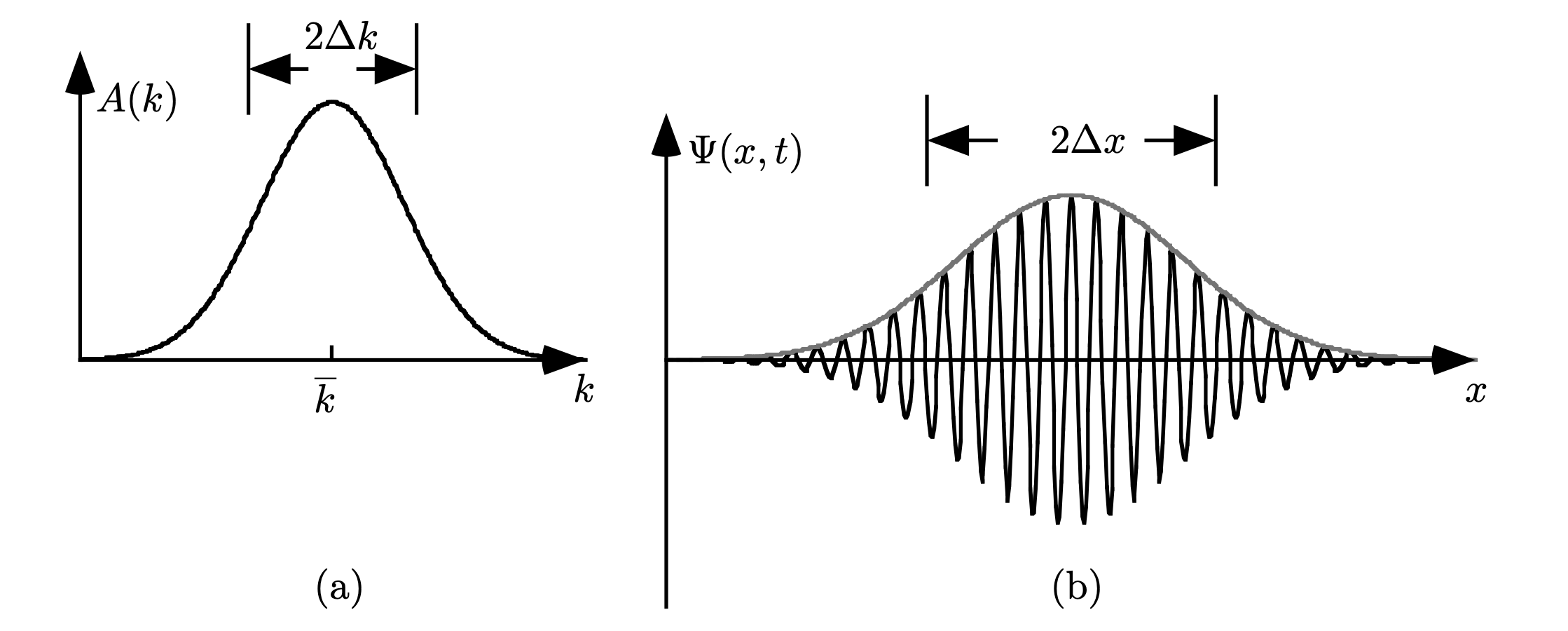
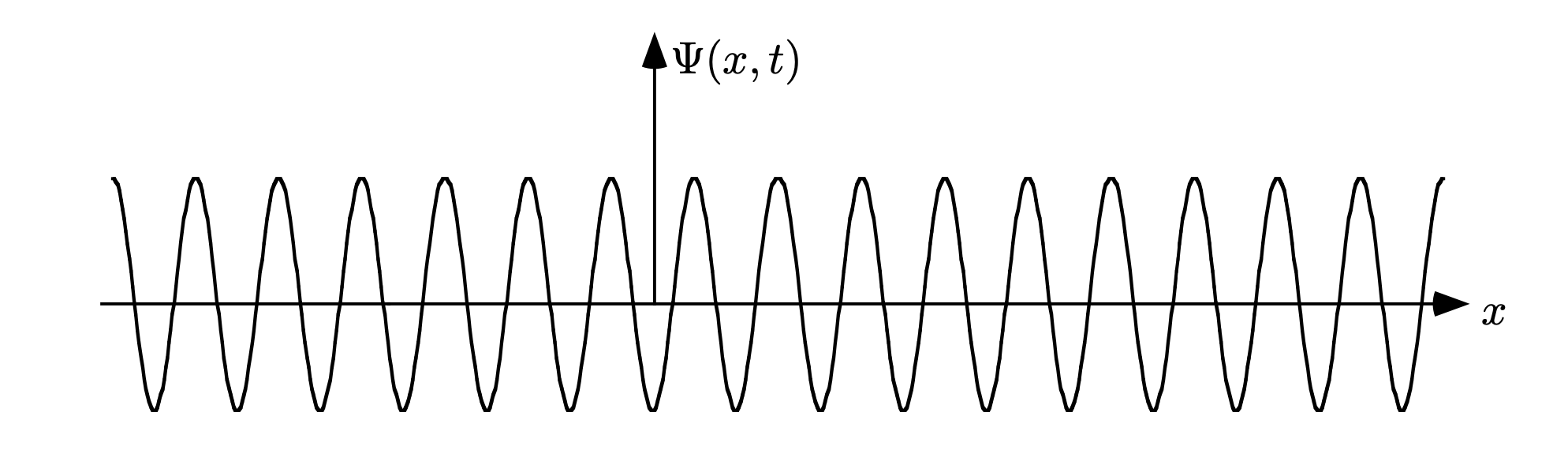
enter image description here

Conversely, the less precisely an object is located in space, the more it looks like like a plane wave (the probability of finding it is the same everywhere in space).

enter image description here

To build a peak-like distribution using waves, one has to add to together a large amount of individual waves of different frequencies.

Conversely, a plane wave has only one specific frequency.

So, the more a wave-like object is narrowly located in space, the looser it is located in the frequency domain.

Conversely, the narrower the frequency distribution of of an object, the larger its spread in ordinary space (cf. a plane wave).

If one considers that the frequency of a wave is related to its momentum, then then the considerations above translate as:

"The more precise the location of a particle is in space, the more uncertainty there is regarding its momentum."

Since quantum mechanics obeys the Schrödinger equation, the considerations above apply to all particles (i.e. to matter in general).

Here is a formal description:

http://physics.mq.edu.au/~jcresser/Phys201/LectureNotes/WaveFunction.pdf

Conclusion:

If quantum mechanics is correct, then it implies that the Heisenberg principle is true, conversely, if the Heisenberg principal was proven to be wrong, then it would prove wave functions not to be an accurate description of reality, Schrödinger's equation would be proven to be an inaccurate description of the evolution of a physical system and the whole of quantum mechanics would be invalidated by the same token.

Improve this answer
$\endgroup$
5
  • 3
    $\begingroup$ Hmm, sounds to me more like an explanation of the uncertainty principle but not an answer to the question why the uncertainty principle is an indispensable prerequisite for QM to work. $\endgroup$
    – Hartmut Braun
    Commented Jun 9, 2021 at 16:16
  • $\begingroup$ Look at the conclusion: it is a demonstration ad absurdum... $\endgroup$
    – Serge Hulne
    Commented Jun 9, 2021 at 16:26
  • 1
    $\begingroup$ In other words, the Heisenberg principle derives from Schrödinger's equation. No Heisenberg, no Schrödinger, no quantum mechanics. QED. $\endgroup$
    – Serge Hulne
    Commented Jun 9, 2021 at 16:27
  • 1
    $\begingroup$ I see. (Schrödinger => Heisenberg) <=> (No Heisenberg => No Schrödinger). Still, I feel (like OP in his/her question) that Feynman was pointing at something different, a more abstract argument that does not require a specific representation of QM, like Schrödingers equation does. Remember that Heisenberg and Schrödinger derived different representations of QM that only later have been shown to be equivalent. $\endgroup$
    – Hartmut Braun
    Commented Jun 10, 2021 at 5:13
  • $\begingroup$ Yes, perhaps he did... $\endgroup$
    – Serge Hulne
    Commented Jun 10, 2021 at 9:03

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.