3
$\begingroup$

I was inspired by comments under this answer to ask this question.
In the context of AdS/CFT, one often finds an embedding diagram of the $10d$ spacetime that I don't find particularly enlightening, showing only the $r$ coordinate of what will be, in the low-$r$-limit AdS, and one angular coordinate of $S^5$:
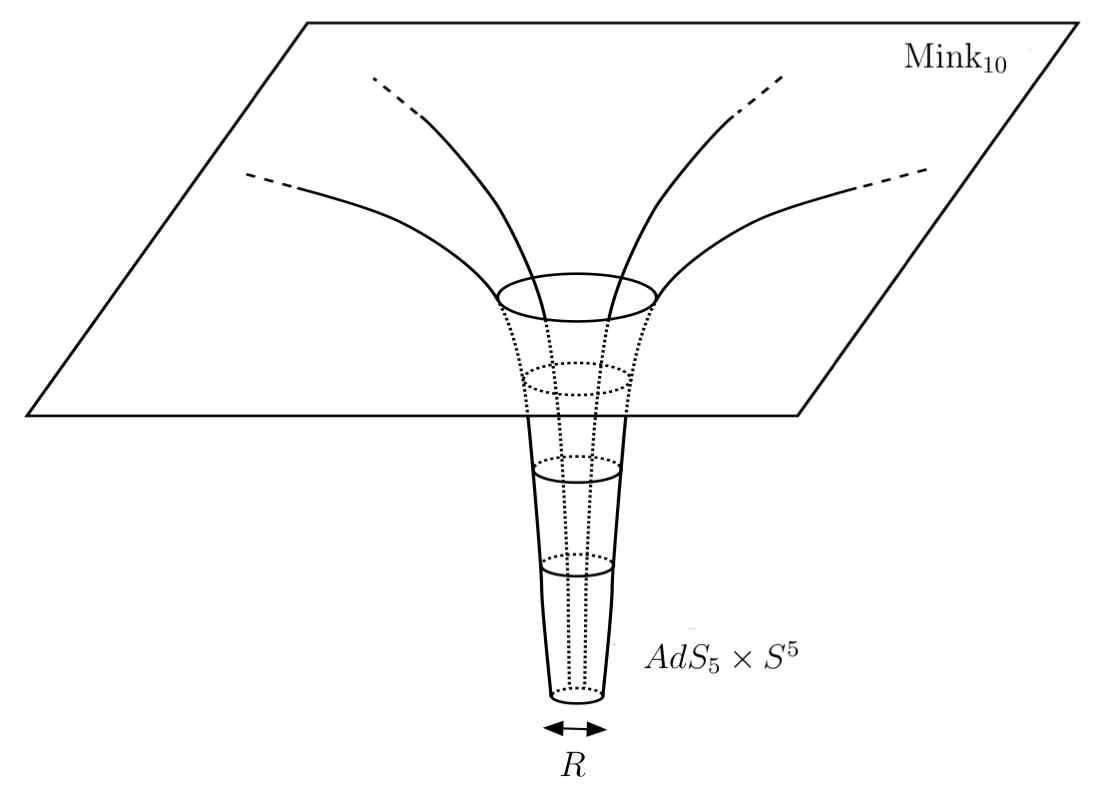
enter image description here
with the source (N D$_3$-branes) sitting at $r=0$. From what I understand, it shows that, under the influence of N D$_3$-branes, an observer starting from the $\mathbb{M}^{10}$ at $r=\infty$ going towards them, finds that the geometry curves and approaches $AdS_5\times S^5$, but the proper distance to reach $r=0$ (and therefore the $AdS_5\times S^5$ metric) is infinite, so it will never get there but only approach $r=R$. Obviously, the interesting limit is for low $r$, and therefore one studies the AdS metric and its compactification. One then usually finds this other picture:
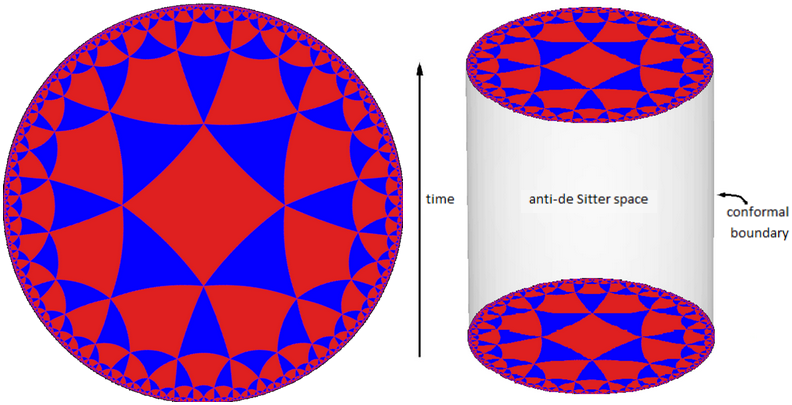
enter image description here
where the coordinates shown are the time $t$, the compactified radial coordinate $\rho=\arctan(r)$, and one angular coordinate.

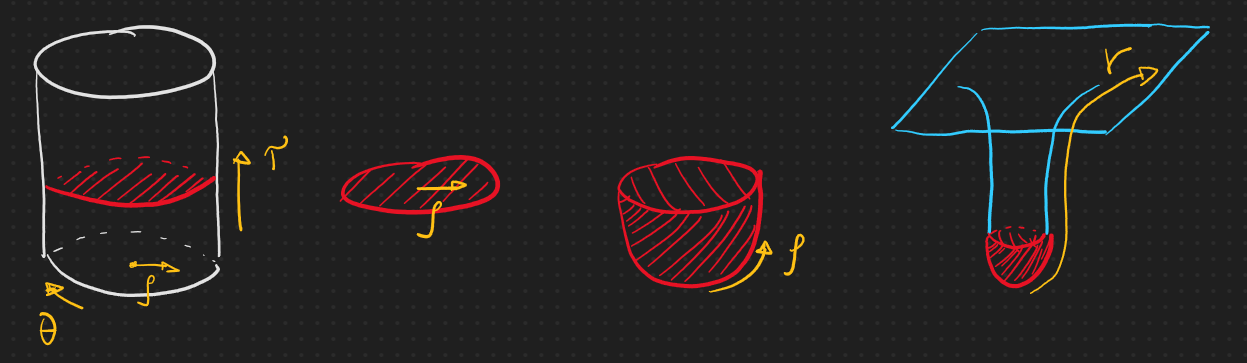
Now for the question: dropping the time dependence in the second picture (and possibly the angular coordinates), is there a way to put them together, to see both the "flat-to-AdSxS" behavior and the "AdS-with-boundary"? My best bet is this, but I'm pretty sure it is wrong:enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ This purely a visualization matter, but I would like to stand a little bit on the physics behind it. You say that you don't find it particularly elucidating to represent the holographic r-coordinate. I strongly disagree with that. It is the expansion with respect to that particular coordinate that gives much information about the system under consideration and has many implications. For example in the case of D$3$-branes there exists the flat space limit, which is suggestive for the study of scattering amplitudes. Other brane configurations do not enjoy such a limit. $\endgroup$
    – user172341
    Commented Jun 8, 2021 at 9:48
  • $\begingroup$ As you see, I also disagree with your statement that only the near-horizon limit is interesting. It might appear so in the AdS$_5 \times$S$^5$ vacuum that has been extensively studied but even in that case it's not a true statement. Finally, the r-dimension maps to the energy of the CFT... $\endgroup$
    – user172341
    Commented Jun 8, 2021 at 10:02
  • $\begingroup$ @DiSp0sablE_H3r0 I didn't say that I don't find the r-coordinate elucidating, I said that I don't think that showing only the r-coordinate is useful to visualize what's going on! My point, as you can read in the last paragraph, is that the other coordinate (the angular one of $S^5$), under which there is a symmetry, is not useful to see in such a diagram. $\endgroup$
    – Mauro Giliberti
    Commented Jun 8, 2021 at 10:53
  • 1
    $\begingroup$ Maybe I misunderstood your post. Apologies. I wanted to stress, though, why "the interesting limit is for low r" this statement is misleading at least to me. $\endgroup$
    – user172341
    Commented Jun 8, 2021 at 10:58
  • $\begingroup$ @DiSp0sablE_H3r0 you're probably right. I meant that, for high r, what's going on is (at least for me) easily understandable: flat space, slowly curving. When r is low though, you got some compactification, a negative cosmological constant, and an infinite throat, that I can really picture well in my mind (hence, the question). $\endgroup$
    – Mauro Giliberti
    Commented Jun 8, 2021 at 11:59

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.