Transforming Metric from (position-dependent) Polar Coordinates to (position-independent) Cartesian Coordinates

Question

The following example is taken form Relativity, Gravitation and Cosmology, Ta-Pei Cheng, 2nd edition, page 89.

Fig. 1

$ds^2=(dx^1, dx^2) \begin{pmatrix}g_{11} & g_{12} \\ g_{21} & g_{22}\end{pmatrix}\begin{pmatrix}dx^1\\ dx^2\end{pmatrix} \tag{1}$

Here are further examples of metric tensors for two dimensional surfaces, calculated by using the fact the any surface in the small can be approximated by a plane having Cartesian coordinates. We also take this occasion to discuss the possibility of using the metric tensor to determine whether a surface is curved or not. For a flat surface, we can find a set of coordinates so that the metric tensor is position-independent; this is not the case in a curved surface as $g_{ab} = \mathbf{e}_a\cdot\mathbf{e}_b$ in a curved space must change from point to point.

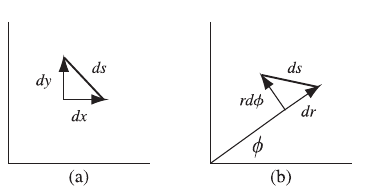
1. A plane surface with Cartesian coordinates For the coordinates $(x^1,x^2)=(x,y)$, we have the infinitesimal length $ds^2 = dx^2 + dy^2$ Fig. 1(a). Comparing this to the general expression in (1), we see that the metric must be

$g_{ab} = \begin{pmatrix} 1 && 0 \\ 0 && 1 \end{pmatrix} \tag{2}$
which is of course position-independent. This is possible only if the space is not curved.

2. A plane surface with polar coordinates For the coordinates $(x^1, x^2)=(r,\phi)$, we have the infinitesimal length $ds^2 = (dr)^2 + (rd\phi)^2$, Fig. 1(b), thus according to (1), a metric

$g_{ab}=\begin{pmatrix}1 & 0 \\ 0 & r^2\end{pmatrix} \tag{3}$

which is position dependent! But we can find a coordinate transformation $(x^1, x^2) \to (x'^1, x'^2)$ so that the metric in the new coordinates is position independent, $g_{ab}'=\delta_{ab}$. Of course, the new coordinates are just the Cartesian coordinates $(x'^1, x'^2)=(x,y)$:

$x = r\cos\phi, \quad y=r\sin\phi \tag{4}$

Now while the whole thing makes intuitively sense I fail to use the given transformation in 2. to transform (3) into a position independent metric.

What exactly would be the correct transformation of $ds^2 = (dr)^2 + (rd\phi)^2$?

Answer 1

The metric tensor is just like any other tensor. Its components transform as $$\bar{g}_{ij} = \frac{\partial x^k}{\partial \bar{x}^i} \frac{\partial x^l}{\partial \bar{x}^j} g_{kl}$$ Where $\bar{x}$ and $x$ are the new and old coordinates respectively. Tensors themselves always remain the same regardless of the choice of coordinates. It's the components that depend on the choice of coordinates.

Answer 2

It is simpler than you think. The metric $$ g_{\mu\nu}=\begin{pmatrix}1 & 0 \\ 0 & r^2\end{pmatrix}\,,\quad\text{ i.e., }\quad ds^2=dr^2+r^2\,d\phi^2 $$ has one position dependent component $g_{\phi\phi}$ because it is expressed in the coordinate frame $\{\partial_r,\partial_\phi\}$ who's coframe are the one-forms $dr$ and $d\phi\,.$

If we switch to the moving frame (non coordinate basis, tetrad, zweibein) $$ \textstyle\{\partial_r,\frac{1}{r}\partial_\phi\} $$ who's dual one-forms are $$ \boldsymbol{\omega}^1=dr\,,\quad \boldsymbol{\omega}^2=r\,d\phi $$ then the metric obviously takes the form $$ ds^2=\boldsymbol{\omega}^1\otimes \boldsymbol{\omega}^1+\boldsymbol{\omega}^2\otimes \boldsymbol{\omega}^2 $$ and has constant components: $$ \eta_{ab}=\begin{pmatrix}1 & 0 \\ 0 & 1\end{pmatrix}\,. $$ It is a good exercise in tetrad formalism to check that the curvature is zero as it should be the case for the plane in any frame. In polar coordinates the Christoffel symbols are $$ \textstyle\Gamma^r_{\phi\phi}=-r\,,\quad \Gamma^\phi_{r\phi}=\Gamma^\phi_{\phi r}=\frac{1}{r} $$ which leads to a zero Riemann tensor. In the tetrad the connection one-forms ${\boldsymbol{\omega}^a}_b$ lead to a metric compatible and torsion-free connection when they are anti-symmetric in $a$ and $b\,.$ Further, they must satisfy the first structure equation $$ d\boldsymbol{\omega}^a=\boldsymbol{\omega}^b\wedge{\boldsymbol{\omega}^a}_b\,. $$ It is easy to see that the solution is ${\boldsymbol{\omega}^1}_2=-{\boldsymbol{\omega}^2}_1=-d\phi\,.$ Therefore the curvature two-form is \begin{align} {{\mathscr R}^{\,a}}_b=d{\boldsymbol{\omega}^a}_b+{\boldsymbol{\omega}^a}_c\wedge {\boldsymbol{\omega}^c}_b=0 \end{align} as expected.