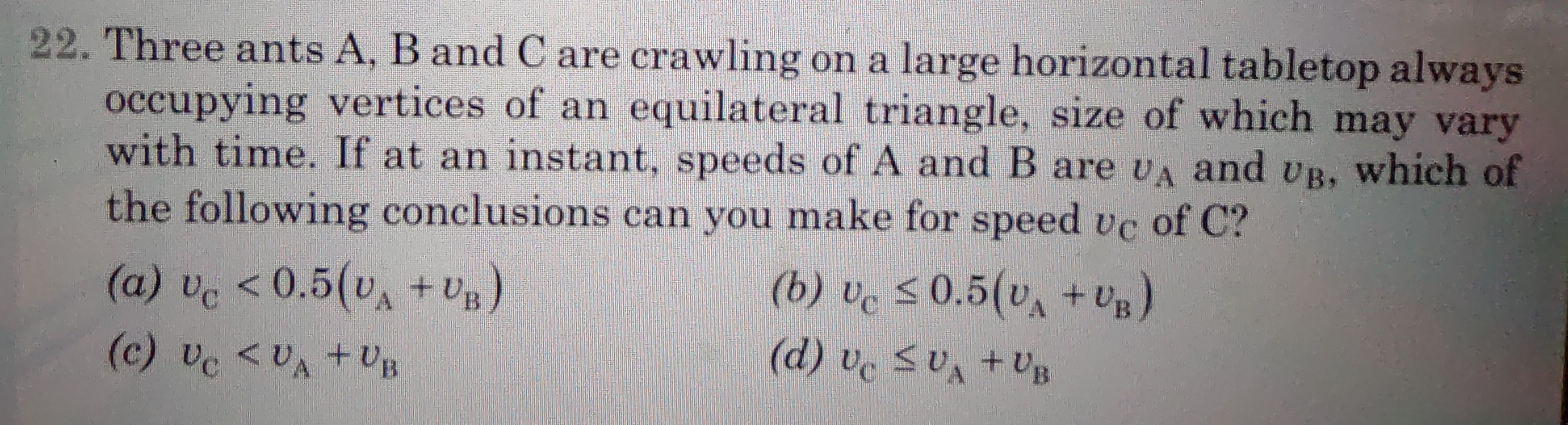My Approach:For any arbitrary motion of A and B, C must move in such a way that it forms a new equilateral triangle A'B'C'. Now how do I derive a relation between their displacement vectors so as to conclude about C's speed?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ Seems $v_n$ is supposed to be a magnitude and not a velocity. I was just thinking about pure translation, rotation, and expansion required to maintain the equilateral shape and in all those cases it seems to me the magnitudes of the velocities have to be identical or else distortion would occur. And if it's linear, then superposition you could get combinations of all three motions and the magnitudes still wouldn't change. But that doesn't match any the answers. That's my thinking. This actually sounds more like a math question than a physics question to me. $\endgroup$– DKNguyenCommented May 30, 2021 at 4:57
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
As it's a homework question here is a hint:
Imagine Ant A is stationary (it could be)...
The let's say B moves a small distance, along the line AB. How would this change distance AB?
Then, what would this mean for how distance AC has to change, and in what direction must C move - then what would that mean for what $V_C$ has to be (in terms of $V_B$)?
This seems to rule out three of the options.
answered May 30, 2021 at 20:55