Analytically proving that the yo-yo rolls to the right (angular kinematics) [closed]

Question

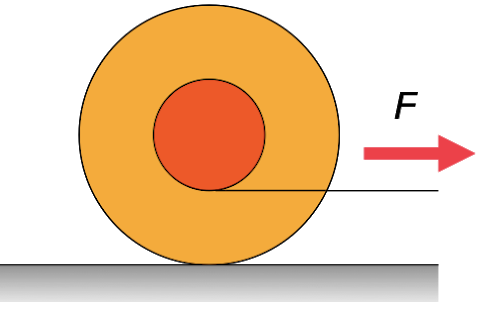
Assume that the yoyo is always rolling without slipping.

Physical experiments shows that the yoyo would roll to the right.

I'm aware of some ways to show this without analytically solving the system (e.g. by changing the axis of rotation to be the point of contact with the yoyo and the floor). However, as an exercise I am trying to prove this result analytically.

To show this, my intuition would be that the final goal is to show that the torque generated by the force $F$ on the red wheel is smaller in magnitude than the torque generated by the static friction force (at the point of contact with the floor) on the larger orange wheel.

Some initial equations I laid out were:

$\tau_1 = F \cdot r_1$

i.e. equation for torque $\tau_1$ generated by the force $F$, where $r_1$ is the radius of the red disk

$\tau_2 = F_f \cdot r_2$

i.e. equation for torque $\tau_2$ generated by friction force $F_f$, where $r_2$ is the radius of the orange disk

Next, I try to find a relationship between $F$ and $F_f$, but I am getting confused here. Since we are not slipping, this implies the only friction force is static. But this would imply $F = F_f$. But then this would imply the centre of mass of the yoyo is not accelerating. Hence the yoyo is stationary?

If the yoyo were already in motion, then this would be fine. We would have $F = F_s$, and then since $r_2 > r_1$, we have that $|\tau_2| > |\tau_1|$ as required. But if the yoyo is stationary, what happens?

Thank you!

Answer 1

You've sort of proven your own answer in the statement of the question. If it is moving, then the force to the right must be greater than friction. Since you assume it is rolling without slipping, it will roll to the right, done.

The more interesting question (which I think is conceptually what you're getting at) is under what conditions it will roll to the right without slipping and when would it unravel the other direction. Since that is the boundary where it goes from rolling without slipping to sliding, that will be when $F \cdot r_1 \geq \mu_s N \cdot r_2$. When that condition is met, the friction will switch to kinetic and the yo-yo will start spinning to the left while it slides (since $\mu_k < \mu_s$). Interestingly, there isn't a specific value of $F$ where it just slides (provided you start from rest) since the friction changes discontinuously.