What would you see dropping a sufficiently strong chain with substantial length into a black hole?

Question

Here's a visual representation of the scenario

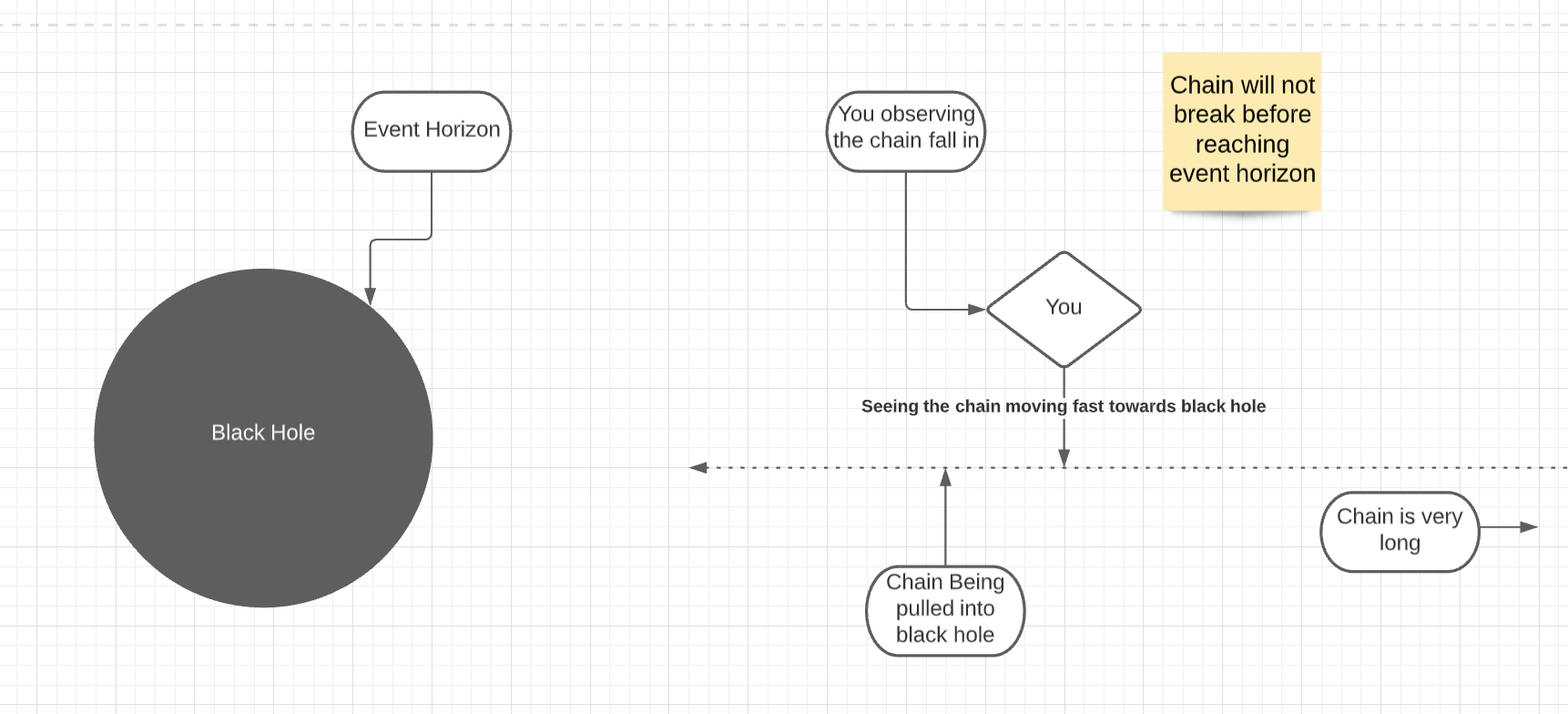
Here you can see we have a black hole on the left. The event horizon is the edge of the black hole. You are far away from the event horizon, and a chain is passing you by fast heading toward the black hole. Due to the size of the black hole and the makeup of the chain, the chain will not break before reaching the event horizon.

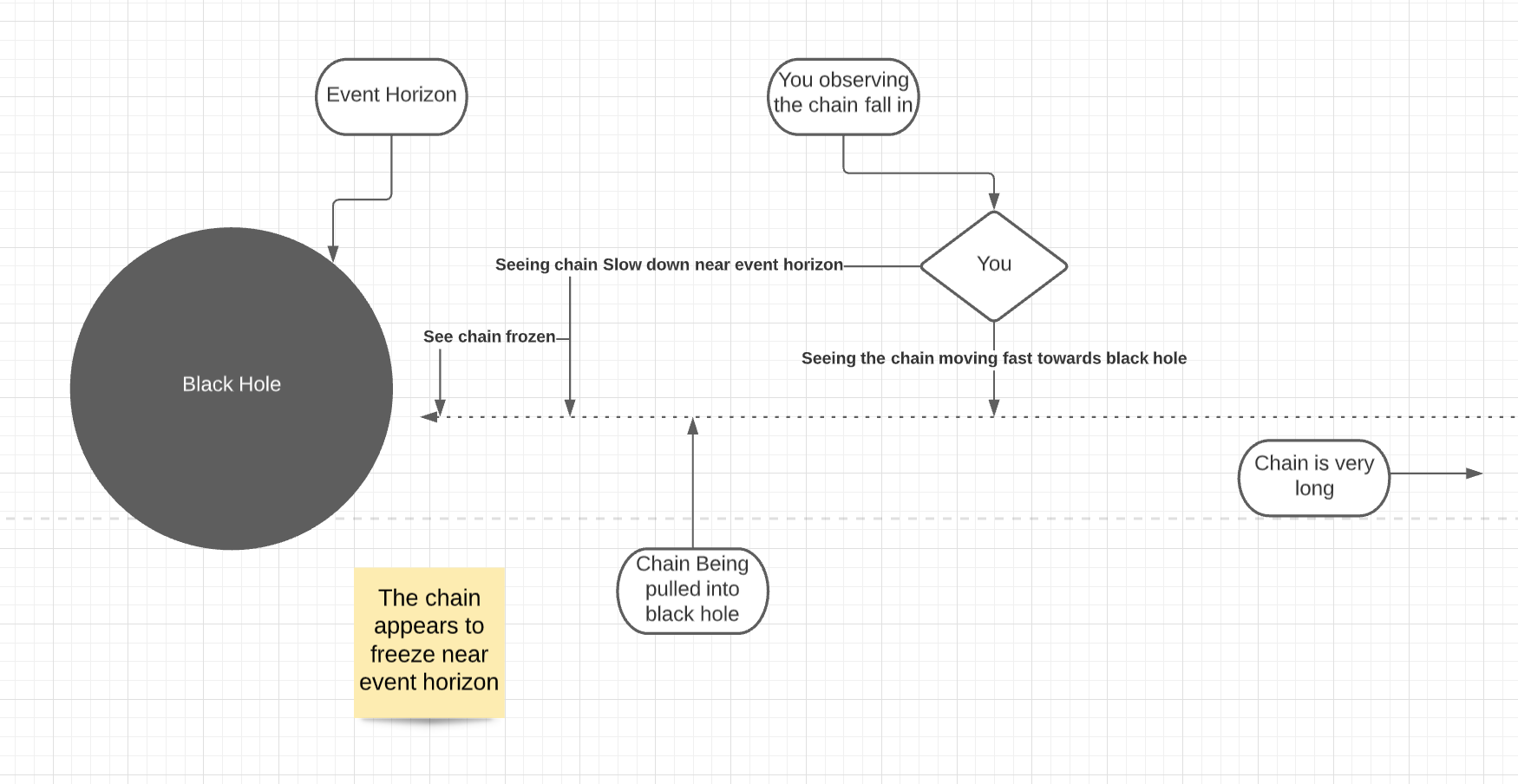
After a while, the following scene happens:

As the end of the chain approaches the event horizon, the chain slows down due to the immense gravity as it approaches a frozen state.

As I show in the diagram, you can observe this phenomenon as well as observe the chain moving fast by you toward the black hole.

As for my question..

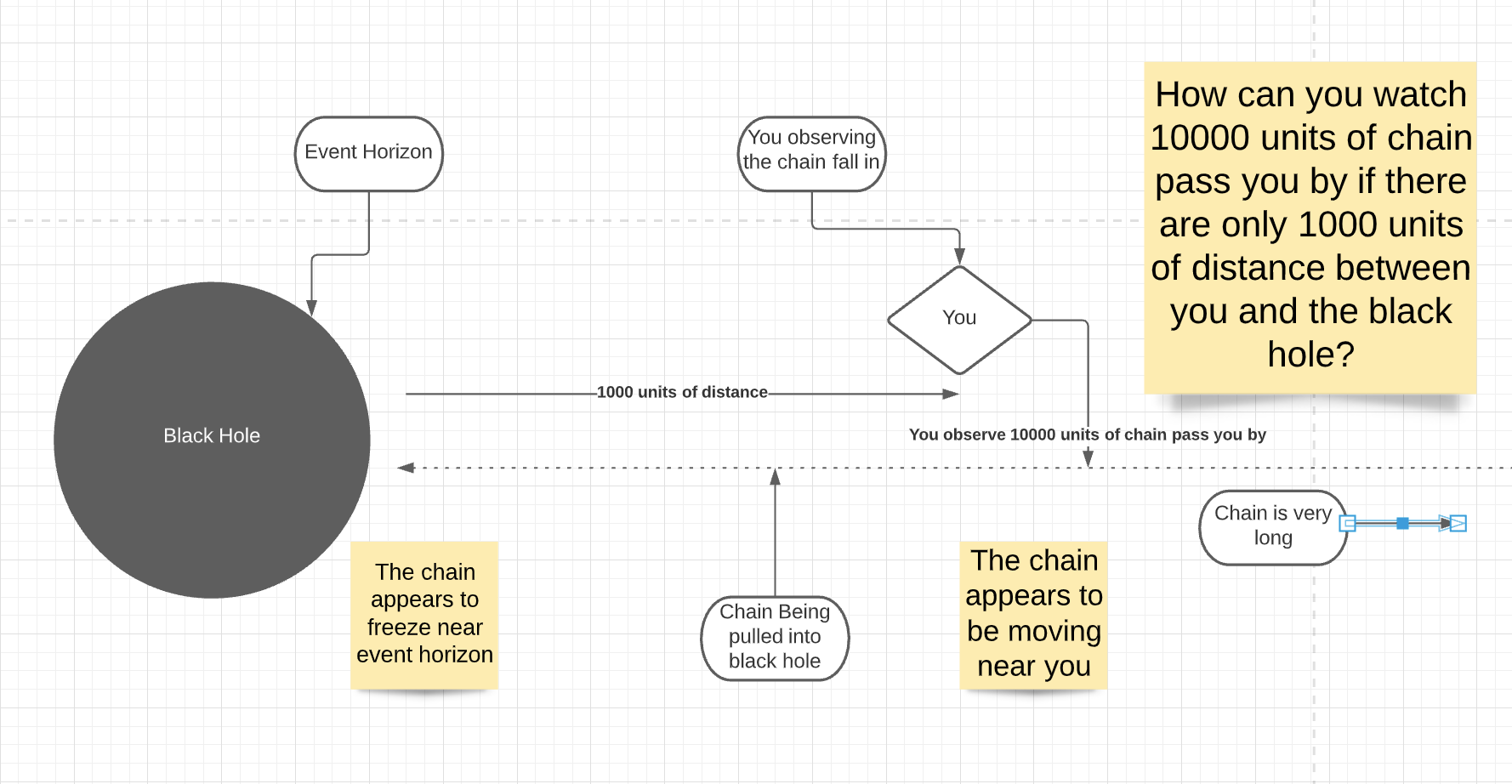
How can the part of the chain near you appear to be moving quickly toward the black hole, while the end near the black hole is frozen (or close to it)? Where does all that chain go?

Let me ask the same question in another way..

If the distance between you and the black hole is 1000 units, and the chain appears to be almost frozen 1000 units away from you, how could you reconcile watching 10000 units of chain speed past you? How does that 10000 units appear to fit within a distance of 1000 units from your perspective?

Answer 1

I would like to add something that the other answers don't address. Redshift.

Your question is a tricky one because you from the observer's view cannot have a moving chain and a frozen chain at the same time.

Arbitrarily close hovering observers will never see anything cross the horizon because of the extreme redshift

When looking for a black hole, will we always find a collapsing star instead?

The answer to your question is extreme redshift. Not only is there extreme time dilation at the horizon, but also extreme redshift. So the links that are closest to the horizon will disappear because the photons coming from them are redshifted so that we cannot detect them any more.

So there is no discrepancy, the observer sees the chain moving, and the links that are closest to the horizon will disappear (because the photons coming from them are redshifted so that we cannot detect them).

Answer 2

As the end of the chain approaches the event horizon, the chain slows down due to the immense gravity as it approaches a frozen state.

The chain doesn't actually slow down. It just appears to, because light from it takes increasingly long to climb out of the gravitational well and return to you.

You could argue that your question doesn't need to be answered because the appearance of the chain slowing down is just an optical illusion and needn't have an interpretation as a picture of what's actually happening near the event horizon.

However, if the hole is large enough and the area of the experiment small enough that curvature can be neglected, you can treat the problem special-relativistically. In that case your paradox is resolved by Lorentz contraction. The farther below you a link of the chain is, the more it's redshifted; the more it's redshifted, the faster it's moving away from you; and the faster it's moving, the more it's contracted in the direction of motion, with respect to your instantaneous rest frame. As a link approaches the horizon, the redshift goes to infinity, and so does the Lorentz contraction factor, so there's room near the horizon for arbitrarily many "frozen" links to stack up.

Answer 3

This never got a very good answer, although the questioner did his best to make a clear question. I asked a similar question about Lorentz contraction when approaching a black hole. My simple thinking had been that if a bowling ball was thrown at us near lightspeed, we'd calculate that the ball shape was squished flat into a 2D disk (I know, it would not appear that way to our eyes). So would a black hole be similarly squished flat? The answer I got was no, that the lorentz contraction is offset by the expansion of the schwarzschild coordinates.

I am interpreting that answer visually, but I think correctly; please don't down vote just because I don't use math, there are different types of thinkers. I use the concept of path increase associated with the Shapiro Time Delay. As a photon passes by the sun, it appears to slow to us, although not to a person in the vicinity of the photon. If we had a giant ruler laid out on the photon's path with the near point to the sun at 6cm, it would look to us on Earth like this:

That is, the ruler would have constant width markings for a small person traveling down its length, but the markings seem to be getting closer together to the observer standing back from it.

At a non-spinning black hole, if you tried to push this ruler into the event horizon, the appearance to you would be as if the end of the ruler were getting ever more squished. You could push from your end (or feed out a chain as in the original question) and it would appear to reel out to a sort of 2D zone, kind of like pouring paint into a flat puddle that magically doesn't spread out. But if you were to climb down the chain, you'd find every link the same length locally! And you could climb down the chain all the way to the end, and then climb back up, without ever having reached the event horizon.

Answer 4

because at the even horizon light cant escape we will see that end disappear as there is no more light for us to see so it is still going into the black hole we just can't see it, as for it looking like it slows down that is just the was space-time has distorted the light, not from the physics being applied to the chain so it is still going at speed.

Answer 5

The first thing to remember is that at the event horizon of a black hole, time dilation is so extreme that, from our frame of reference here on Earth, time has essentially stopped. So you would see nothing happening at all. You could wait for your lifetime, and for the lifetime of all your decedents and nothing would have happened. Not a single link of the chain would have moved by the smallest amount. Even before the chain got to the horizon, time dilation would have slowed the movement so greatly that movement would be extremely slowed.

Answer 6

One end of a chain crosses the event horizon and then that's it. Period. You can't get any information back regarding what's happening inside since geodesics will lead you to singularity regardless of how fast you're moving or on the event horizon since gravitational time dilation will be infinite on event horizon.

Problems regarding your assumptions

• Assuming that your chain is unbreakable and infinite...then even if each block of your chain has incredibly small mass, it's gonna have infinite mass. This will screw up all your calculations.

• You assumed that one can observe the end of the chain, however based on your assumption the length of the chain was infinite so there's actually no end and no beginning. So no-observer can observe the end of the chain. You put the testability of your question beyond anyone's reach.

• You are creating a similar paradox to "what happens when an unstoppable train meets unbreakable wall" (singularity and unbreakable chain in your example).