0
$\begingroup$

MOND is controlled by

$\mu=\mu(\frac{a}{a_0})$

and

$\mu\rightarrow1$ when $a\rightarrow0$

But on my table $a=0$ so shouldn't I see MOND by just placing some weight on my weighter?

If it is wrong statement, then what do I need in my hypotherical lab to see MOND in table experiments?

The gravitational acceleration of a mass of one kilogram, induced on a probe mass at a distance of 1 meter is G, which is $10^{-11}$, i.e. much less than acceleration from a Galaxy, induced on a Sun, which is suffering from MOND.

I.e. MOND should be directly visible by measuring of attraction of two kilograms at 1 meter from each other.

Isn't it?

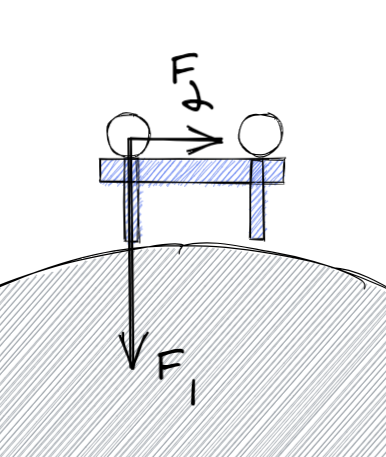
Regard two bodies of 1 kg, laying on table.

enter image description here

I agree, that the force $F_1$ is due to big gravity from Earth and hence MOND effects should be negligible. This force should be indistinguisheable from conventional gravity. But force $F_2$, induced by attraction of second body is very small. It is smaller, than attraction of a Sun from the Galaxy. Since Sun is the subject of MOND, then $F_2$ should also be MOND-affected. Once we measure it, we should immediately see, if MOND is correct or not.

Is there any error in this reasoning?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

On your table, the acceleration due to gravity is $a=9.8\ {\rm m/s^2}$, which you can't ignore in this context, and which is a lot larger than the MOND acceleration $a_0\sim 1.2 \times 10^{-10} {\rm m/s^2}$.

Even if you were in free fall around Earth, you couldn't ignore the acceleration of the Earth around the sun $\sim 6 \times 10^{-3}\ {\rm m/s^2}$ unless you could get outside of the Earth's gravitational field.

On the other hand, the acceleration of the sun around the center of the galaxy $\sim 1.7 \times 10^{-10}\ {\rm m/s^2}$. So if you could escape the Sun's gravitational field and do a high-precision gravity experiment, you could try to probe MOND this way. This fact was behind attempts to explain the Pioneer anomaly using MOND. However, the Pioneer anomaly is currently understood as due to the thermal recoil force.

Improve this answer
$\endgroup$
15
  • $\begingroup$ So, since g is gravitational field intensity, can we say tha MOND works in weak fields? Since fields of any mesoscopic bodies are weak, ergo any experiment measuring attraction of two kilograms (for ex) or smaller bodies will immideately confirm or reject MOND, right? $\endgroup$
    – Dims
    Commented May 17, 2021 at 12:29
  • $\begingroup$ In the context of GR, weak field usually means you are well outside the Schwarzschild radius, and $ v \ll c $, or post newtonian is good enough. MOND is nano-gravity, or 1 billionth of a g. $\endgroup$
    – JEB
    Commented May 17, 2021 at 17:46
  • $\begingroup$ @Dims If you could measure the attraction accurately between two kg masses that were outside of the gravitational influence of the Earth and Sun, then I suppose you could in principle look for MOND. But just to be clear this is very hard and unrealistic, and is not something you can do in a lab. $\endgroup$
    – Andrew
    Commented May 17, 2021 at 19:31
  • $\begingroup$ @Andrew do you mean MOND violates basic mechanical principles for example vector decomposition of forces and accelerations? I.e. if attraction of two kilograms acts horizontal it doesn't show MOND effects due to Earth acting, despite it acts vertical? $\endgroup$
    – Dims
    Commented May 18, 2021 at 9:47
  • $\begingroup$ @JEB the acceleration, produced by a mass of 1 kilogram at a distance of one meters is of order of G, i.e. 10x-11, i.e. one hundredth of one billionth. So it is enough to see MOND. $\endgroup$
    – Dims
    Commented May 18, 2021 at 9:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.