0
$\begingroup$

I am reading AP French's Special Relativity, and I found an interesting problem there.

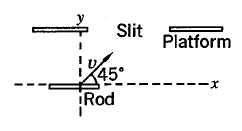
A rod of proper length L points along the $x$ axis but moves in a direction making an angle of $45^°$ to this axis (see the figure). A platform, also parallel to the $x$ axis, lies in the rod's way, but a slit of proper length $1.1L$ has been cut out of it so that the rod can easily fit through if it travels at a nonrelativistic speed. What happens if its speed is $0.9c$? Analyze the problem from both reference frames.
enter image description here
Ans- It fits through

As it is given that at non-relativistic speed , the rod fits into the slit. This means that length of rod=$1.1L$ (no length contraction). Also the centre of slit lies on the centre of rod when rod reaches the slit.

Consider a frame in which the rod appears to move with velocity $0.9c$ at $45^o$ to the $x-$ axis.
In its rest frame, the length of rod in the direction along the velocity is $\frac{1.1L}{\sqrt{2}}$ and perpendicular to the velocity is $\frac{1.1L}{\sqrt{2}}$. These are the rest lengths of the rod.
So the length of rod along the direction of velocity of rod becomes
$l_{||}=\frac{1.1L}{\sqrt{2}}\sqrt{\Big(1-\frac{(0.9c)^2}{c^2}\Big)}=\frac{1.1L}{\sqrt{2}}\sqrt{(1-0.81)}=\frac{1.1L}{\sqrt{2}}\sqrt{0.19}<\frac{1.1L}{\sqrt{2}}$

$l_{\perp}=\frac{1.1L}{\sqrt{2}}$

So from the second figure of the diagram given below, we can see that the dashed line is the $l_{||}$ which is less than actual length parallel to the velocity. $l_{\perp}$ remains the same. Thus the observer observes the tilting of the rod as shown by the red line.

enter image description here

Similarly in the frame in which rod appears to be at rest the slit becomes tilted.
But the answer is given that the rod will fit in the slit in both the frames.
How is it possible?

Please help me in clarifying the doubt.

Improve this question
$\endgroup$
4
  • $\begingroup$ Please show your work, this way it will be easier to notice your mistake. I don't have access to pen and paper, yet what I got in my head based on a few lines of computation agrees with the answer provided by the book. $\endgroup$
    – Monopole
    Commented May 17, 2021 at 5:58
  • $\begingroup$ I have included the diagram of what I think. You can see that from the diagram, which I have made. the rod is not horizontal when it is moving at speed $0.9c$ at $45^o$ with $x-$ axis. So how will it fit the slit? Because slit remains horizontal. That is the question? $\endgroup$
    – Iti
    Commented May 17, 2021 at 6:05
  • $\begingroup$ Without showing how you got the length of the red line and etc. I think diagram itself isn't self explanatory. $\endgroup$
    – Monopole
    Commented May 17, 2021 at 6:14
  • $\begingroup$ I have updated the question showing my calculations. Please see it. $\endgroup$
    – Iti
    Commented May 17, 2021 at 6:29

1 Answer 1

Reset to default
0
$\begingroup$

You have said in your reasoning that the length of the rod is 1.1L- that is a mistake, as the original question clearly states that the rod has a proper length of L.

Otherwise, your reasoning is correct. From the perspective of the gap, the component of the approaching rod's length perpendicular to the direction of motion will remain unchanged, but the component parallel will be reduced, so the rod will appear rotated and shortened.

An entirely reciprocal effect will be seen by the rod- the component of the length of the slit parallel to the direction of motion will be reduced, while the component normal to it will remain unchanged, so the platform will appear tilted and the slit reduced in length.

By drawing some diagrams you should be able to convince yourself that only the component of lengths normal to the direction of motion determine whether the rod passes through the slit, and since they remain unchanged by speed, the rod will always pass through the slit.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Can you please give some hint why the component of length perpendicular to the rod will determine whether the rod will pass through or not? $\endgroup$
    – Iti
    Commented May 17, 2021 at 8:54
  • 1
    $\begingroup$ Indeed. Imagine if you are carrying a box through a doorway. Provided the box is narrower than the doorway, it will fit through it, regardless of how long the box is. Only the component of the box's size parallel with the doorway determines whether the box fits- the component parallel to the direction in which you are walking through the doorway is irrelevant. $\endgroup$
    – Professor Sushing
    Commented May 17, 2021 at 9:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.