If you sliced the moon in half perfectly, would it hold together?

Question

Just a big ol' slice down the middle. Would it drift apart over time, or eventually fuse back together?

Answer 1

Assuming you don't separate the halves to any great extent, it would immediately fuse back together due to its self-gravity. The tidal forces exerted by the Earth on the Moon are only sufficient to overcome this gravity if it were much closer (about the radius of the Earth actually).

This is actually how the Moon is held together, rather than atomic or molecular bonds. However a rough calculation would be to assume the "centres of gravity" of the two halves are separated by $\sim R_M/2$. This gives a gravitational acceleration between them of $2GM_M/R_M^2$. The tidal acceleration, assuming it is at its greatest, with the halves separated along the Earth-Moon line, would be $2GM_E R_M/d^3$, where $d$ is the Earth-Moon distance.

The ratio of self-gravity to tidal force is $$\frac{g}{g_{\rm tidal}} = \left(\frac{M_M}{M_E}\right) \left(\frac{d}{R_M}\right)^3\ .$$

Putting the numbers in, the ratio is $1.5 \times 10^5$.

EDIT: To distinguish my answers from others, let me point out that if you sliced the Moon in half and then separated the halves by a distance much greater than $R_M/2$, let's call it $s$ for separation, then the ratio calculated above changes to something like $$\frac{g}{g_{\rm tidal}} = \left(\frac{M_M}{M_E}\right) \left(\frac{d^3}{2s^3}\right)\ .$$ Thus if you separated the halves by $\sim 40 R_M$ along the line from the Earth to the Moon, then tidal forces would overcome self-gravity.

EDIT2: This calculation should be regarded as very rough and is more accurately a condition for the fragments to form a stable, bound binary system. The problem of a low-mass binary orbiting a larger mass does have approximate criteria for long-term stability (e.g. Donnison 1988). It does depend a bit on eccentricity and on whether the two fragments orbit in retrograde or prograde fashion. For a binary separation $s$ and distance from the Earth $d$, the results of Donnison suggest ratios of $d/s > 12$ (prograde) or $>22$ (retrograde), for something with the mass ratio of the Moon/Earth - so $s<20R_M$ or $<11 R_M$ respectively.

Inevitably, what will happen and whether the two halves would fall back together, would depend on the initial conditions. If you just magically separate them along a radial line from the Earth but with each fragment keeping the original angular velocity, then that necessarily means you inject a large amount of angular momentum around the centre of mass and the fragments would just orbit each other. Further speculation is not worth the effort without a specific set of initial conditions.

Answer 2

General relativity is a more accurate theory than Newton's mechanics/gravity for calculating gravitational effects. But here, since we are dealing with a low-speed problem in the weak-field limit, the context of Newtonian mechanics/gravity is sufficient for our purposes.

A general conclusion

They certainly will join together if the distance between the halves (hemispheres) is not too large. In order to prove this, first we have to compute the gravitational force between two halves and then evaluate the time needed for reconnecting. And, the tidal forces do not play a crucial role here unless the distance is large (see a justification in the appendix).

This problem is an interesting exercise in Classical Mechanics course and it can be computed using the Poisson's equation for gravity. For example, in this article, different cases are explicitly calculated. However, there is an easy, more intuitively way to approximately calculate the gravitational attraction between hemispherical masses. So, the discussion here is simplified in my answer to provide a good estimation.

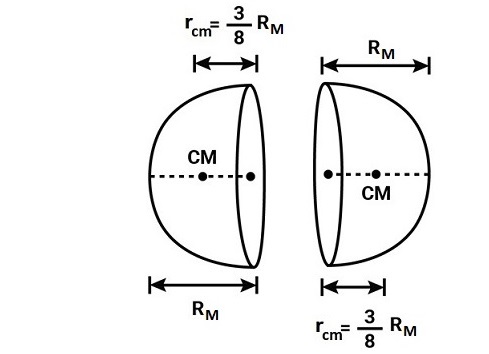
Suppose we divide the Moon (with the radius $R_{\rm{M}}=1737.4 \,{\rm{km}} \simeq 1.74 \times 10^{6} \, \rm{m}$ and the mass $M_\rm{Moon}=7.342 \times 10^{22}$ $\rm{kg}$) into two halves and the distance between these halves is small, e.g., $1$ $\rm{meter}$ (after reading this answer, you can even try $1$ $\rm{km}$). For each hemisphere, we have to find the center of mass point, the point to which the total gravitational force is applied (see WikiPedia about center of mass). For a hemisphere the location of center of mass¹ is computed as ${r_\rm{cm}} = \frac{3}{8}{R_\rm{M}}$. See the following figure.

The distance between the hemisphere (center of) masses is $\Delta r = {r_c} + {r_c} = \frac{3}{4}{R_M}$ (the distance between them is too small in comparison with the radius of Moon). Now, the gravitational force between two hemispheres can be obtained by use of the Newton's gravitational law as

$$\begin{array}{l} F = G\frac{{{m_1}{m_2}}}{{{\Delta r^2}}}\\ \,\,\,\,\,\, = G\frac{{\left( {\frac{{{M_{{\rm{Moon}}}}}}{2}} \right)\left( {\frac{{{M_{{\rm{Moon}}}}}}{2}} \right)}}{{{{\left( {\frac{3}{4}{R_{\rm{M}}}} \right)}^2}}}\\ \,\,\,\,\,\, = \frac{4}{9}G\frac{{M_{{\rm{Moon}}}^2}}{{R_{\rm{M}}^2}} = 5.3 \times {10^{22}}\,{\rm{N}}, \end{array}$$ which is large enough to easily pull the halves towards each other ($G =$ gravitational constant).

The acceleration of one half to the other can also be obtained from Newton's second law, yielding

$$a = \frac{F}{{\left( {\frac{M_\rm{Moon}}{2}} \right)}} \simeq 1.45\frac{{\rm{m}}}{{{{\rm{s}}^{\rm{2}}}}},$$

and compare this to the surface gravity of the Moon, $\sim 1.62$ $\rm{m}/\rm{s²}$.

Finally, assuming that the acceleration is constant during fusion (which indeed is a good approximation since the distance between the halves is small), the net time it takes for the halves to come together is simply obtained from the following equation

$$y = \frac{1}{2}a{t^2}\, \to \,t = \sqrt {\frac{{2y}}{a}} \simeq 1.18 \, {\rm{s}}.$$

So, according to this simple discussion, the hemisphere masses at a distance of one meter from each other will collide with each other quickly, a little over a second. If you take the distance between the two hemispheres to be one centimeter, you will obtain $0.118$ $\rm{s}$. Of course, the shorter the distance, the much less severe the collision.

Finally, it should be emphasized that, because of the Moon's spin, the time it takes for the halves to come together increases (and some new details should be considered). However, the Moon's spin is small since it also takes approximately $27$ days for the Moon to rotate once on its axis. But, simply assuming that the two halves are very close to each other, the problem of the Moon's spin can be ignored with a very good approximation.

Why tidal forces are not important for small separations?

Supposing that the hemispheres are separated along the Earth-Moon line, the nearest hemisphere to Earth is attracted more strongly than the farthest hemisphere. This is the well-known tidal force. In this problem, we obtain the total force of the Earth at the center of gravity of each Moon’s hemisphere as

$${{\vec F}_{{\rm{E,M}}}} = - G\frac{{{M_{{\rm{Earth}}}}\left( {\frac{{{M_{{\rm{Moon}}}}}}{2}} \right)}}{{{d^2}}}\vec r\underbrace { {}\pm G\frac{{2{M_{{\rm{Earth}}}}\left( {\frac{{{M_{{\rm{Moon}}}}}}{2}} \right)}}{{{d^2}}}\frac{{\Delta r}}{d}\vec r}_{{\rm{Tidal}}\,{\rm{forces}}} + {\rm{higher}}\,{\rm{order}}\,{\rm{corrections}}, $$

where $d$ is the distance from the Moon to the Earth. So, the tidal force of the Earth at the center of gravity of each Moon’s hemisphere is computed as

$${F_{{\rm{Tidal}}}} = \frac{3}{2}G{M_{{\rm{Earth}}}}{M_{{\rm{Moon}}}}\frac{{{R_{\rm{M}}}}}{{{d^3}}} \simeq 1.3 \times {10^{18}}\,{\rm{N}},$$

meaning that each hemisphere experiences an acceleration equivalent to $3.6 \times 10^{-6}$ $\frac{\rm{m}}{\rm{s}^2}$ under the gravitational influence of the Earth. Comparing the obtained value for the tidal force with the gravitational attraction between the halves (i.e., $F=5.3 \times {10^{22}}\,{\rm{N}}$ which causes an accelerati equivalent to $\sim 1.45\frac{{\rm{m}}}{{{{\rm{s}}^{\rm{2}}}}}$) shows that why tidal forces for small distances are not important in this problem.

---

Thanks to @NuclearHoagie for checking these calculations and pointing out a mistake in my computation. Also, thanks to @ProfRob for his valuable comments.

Answer 3

The moon's radius is considerably higher than the "potato radius" of some 200-300km depending on material (https://arxiv.org/ftp/arxiv/papers/1004/1004.1091.pdf), so even if you take the two halves, and flipped each so their domed sides faced each other, the moon would just flow together and re-form as a sphere.

If you merely slice it and push the two halves apart by a fraction of an inch, they'll just snap back together again.

Answer 4

The important part is that the Moon is not a ball of steel-reinforced concrete which can withstand tension. It is not even a single piece of solid rock. For example, it has a partially molten inner mantle.

The main force holding a celestial body together is its own gravity which would not change at all if it were cut in two halves. You can as well consider the Moon as consisting of two halves, or a gazillion boulders, already; the molecular forces "gluing" it together are very small.

Consequently, celestial bodies reliably disintegrate if the tidal forces (inner stress exerted by external inhomogeneous gravitational fields) exceed their inner gravitational forces. This happens at the Roche limit, which is entirely independent of the inner makeup of the bodies. Whether it is rocky or gaseous, for example, is irrelevant.

With respect to the Moon I suspect that cutting it with an imaginary cheese wire would be essentially unnoticeable, whether you "suspend gravity" during the process or not. I don't even think there would be much volcanic activity because the metal-rich partially molten inner mantle is heavier than the outer mantle.

Answer 5

I don't know about fusing, but each half would have a mass of 35,000,000,000,000,000,000 tons, or thereabouts, which is about 7,000,000,000,000 times the mass of the Great Pyramid at Giza. I don't need to calculate the gravitational force between the two halves to know they would be held together far more firmly than the pyramid is held to the surface of the Earth. Since the Pyramid has not yet drifted away from the Earth, I am tempted to imagine that the two halves would stay put.

Let is imagine the plane of the cut is normal to the radius of the Moon's orbit around the Earth, so that the farther half is subjected to a lower gravitational pull from the Earth, owing to tidal effects, than the nearer half. Even then the tidal forces would be small compared to the gravitational attraction between the two halves, so I cannot see what would make them separate.

Answer 6

Just like a dust particle stays on the moon due to its gravity, so will all other particles remain with the moon regardless of any slice-through. Without any mass being removed, its gravity is unaffected. Everything else is irrelevant.

(the only thing that could have significant different influence would be the fact that you want gravity to turn off during the slicing. Turning off gravity for a while might have dramatic consequences with the matter of the moon being spread out into space (or into separate dust orbits around the earth) due to the centrifugal forces.)

Answer 7

I would like to add something the other answer do not address, that is the impact's effect on the moon itself and whether that could cause the moon to disintegrate.

Also, this collision will crack Earth into more pieces (bounded by gravity).

https://worldbuilding.stackexchange.com/questions/118073/what-are-the-effects-of-slicing-the-earth-in-half-with-a-particle-beam

There is actually a calculation on worldbuilding (it is for the Earth but the moon is in the ballpark range too) in which they take 40meters for the separation.

Just at this small separation, the gravitational acceleration caused by the stress-energy (not mass) of the two halves is so enormous, that it could crack the moon (the halves) into more pieces and could (if the separation is larger) cause pieces to fly off against gravity.

So the ultimate answer to your question is, that even at this small separation after the halving, the moon might not be intact like it was before, and the effects of the impact of the two halves would greatly depend on the separation (so you better use a sharp cutter).

Answer 8

Of course, it will fuse back. It is held together by gravity.

... and pretty much not only the Moon.

Any celestial body larger than the average comet will fuse back.

Comets sometimes disintegrate roughly in half because of centrifugal force overcoming gravity, but in order to do that, they try very hard to spin themselves up (by asymmetric outgasing).

Answer 9

If you slice it into its 1st and 3rd quarter (visible) parts, then the tidal forces will act to bring the 2 halves together. So, the slice's normal should be aligned with Earth-Moon line...that is, slice it into "full" and "new" halves.

The tidal forces act to pull the halves apart. Following the nominal lunar orbit, the near half has too much gravity and is being pulled inward. The far half has too much centrifugal force, and is flung outward.

The Moon's orbit (385k km) is much larger than the fluid Roche limit (https://en.wikipedia.org/wiki/Roche_limit) of the Earth (18.381k km), so the Moon's self gravitation keeps it together.

Answer 10

First we need to establish the physical difference that we want to cause by our "perfect slice". Since a perfect slice will not displace any material, physically it would not be distinguishable from doing nothing at all. So let's stipulate an actual change in behavior that would be consistent with not displacing material. One such change would be to forego friction on the boundary created by the "slice", meaning that our two half-spheres can frictionlessly move against each other rather than being secured against displacement by friction proportional to the gravitational pressure pulling them together.

Then we would, depending on the orientation, expect tidal forces to be able to displace the sphere halves that would otherwise resist displacement due to friction. Would they fall apart? We can just see what will happen by letting go of frictional shear forces altogether and turning the moon into liquid. It turns out that even a liquid moon would, in its current orbit, be kept in one piece (cf its "Roche limit"). So the "less liquid" case of two frictionlessly joined semispheres would not end up any different.

Answer 11

If the moon were entirely solid then right after you sliced it the two sliced surfaces won't fuse back (if by fusing back you mean that both halves will form one solid whole again), assuming that the high pressure around the core can't refuse the surfaces together around the core. By slicing you basically take the glue away that holds the two pieces together. While gravity holds them in place (as is shown in the other answers), the two halves will not stay at rest relative to each other if a big asteroid hits the moon. In that case, there are three bodies involved in the (inelastic) collision (instead of two), assuming the two halves will globally stay the same before and after the collision. So the two halves will move away from each other initially. After which they damped-oscillating return to each other. This obviously wouldn't have happened if the two halves were still glued together.

What if the moon is not entirely solid? this is suggested by this article from which this picture is taken:

An artist's rendering of the lunar core as identified in new findings by a NASA-led research team. (NASA/MSFC/Renee Weber)

In this case, if you arrive at the fluid part when slicing, the fluid part will refuse shortly after you've sliced it. So there is a fluid connection left. This fluid will not be able though to keep the two halves at rest wrt each other when an asteroid hits one part. So also in that case would the impact of an asteroid will have the result that the two halves initially move away upon which a dampened oscillation follows.

So, if you were living on the moon and the moon was hit by a big asteroid, it could save your life if the moon were cut in half and you would find yourself on the lucky halve.

What no answer has addressed here: Will the two halves refuse again in time? Will two halves of a piece of metal refuse again if you press them together for a long time? No. Will this happen for the two halves of the sliced moon? Likewise, in principle no. You can't make a one-to-one correspondence with two pieces of metal though. Depending on what happens between the two half-moon pieces they will refuse or not. Clearly, the liquid part will always form a whole.