11
$\begingroup$

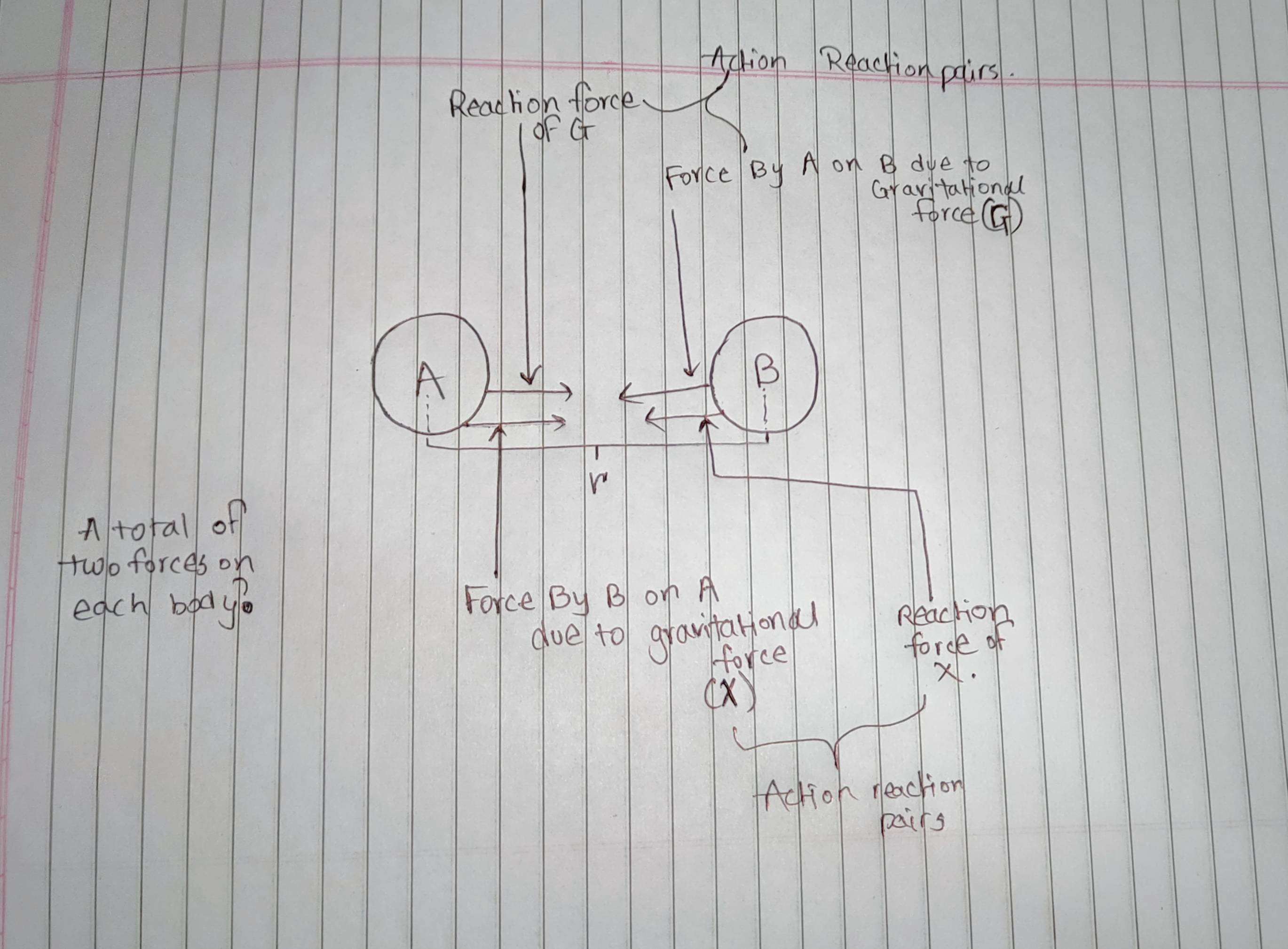
What is the meaning of the word "between" in the law that the force between two masses at separation $r$ is given by $\frac{GM_1M_2}{r^2}$? I am confused about how can a force be in-between, either it is on body A or on body B, or on both.

Suppose body A exerts force $F$ on Body B, so according to Newton's 3rd law of motion B should also exert a force on A.

Let's consider this case for gravitational force between two bodies. If body A exerts force $g$ on Body B, then B body should also exert a force $g$ on A, but B is also exerting the gravitational force $X$ on A, hence A will also exert force $X$ on B. So, how are two forces acting? I have given the representation in this diagram.

The question is, how are Two forces g and X acting on a body

Improve this question
$\endgroup$
6
  • 3
    $\begingroup$ "Between" here is used more along the lines of the first definition listed here, and not the second as you seem to be thinking. So the force between the two bodies is the equal-but-opposite force that either body exerts on the other. $\endgroup$
    – user170231
    Commented May 4, 2021 at 14:55
  • $\begingroup$ What exactly is your question? How does paragraph 2, 3 realte to 1st. Can you express your question more systematically in different way? $\endgroup$
    – user238719
    Commented May 5, 2021 at 2:02
  • $\begingroup$ @HiterDean,Q1)what is meaning of "between" in gravitation law,Q2)how are two forces acting on each body according to intuition in the diagram?..2 one is answered pretty well by all the helpers $\endgroup$
    – Dheeraj Gujrathi
    Commented May 5, 2021 at 5:53
  • $\begingroup$ The word "between" should here be interpreted as "mutual" i.e. "on each other". It doesn't mean "in the space separating the two objects". $\endgroup$
    – md2perpe
    Commented May 5, 2021 at 17:41
  • $\begingroup$ I’m voting to close this question because it is a question of language, not physics. $\endgroup$
    – Jon Custer
    Commented May 6, 2021 at 12:48

6 Answers 6

Reset to default
18
$\begingroup$

Newton's Third Law tells us that the force on A due to B is equal (in magnitude, with opposite direction) to the force on B due to A. Therefore, in any interaction between a pair of objects it is sufficient to describe the force on just one of them, since the other can be deduced by Newton's Third Law. For this reason, it is common to refer to force acting on either object simply as the force "between" the objects. Thus, there should only be one pair of forces, with magnitude $\frac{Gm_Am_B}{r^2}$, to describe the gravitational interaction between a pair of masses.

Improve this answer
$\endgroup$
6
$\begingroup$

**If A body Exerts force G on Body B,then B body should also exert A force G on A,

but B is also exerting the gravitational force X on A,hence A will Also exert Force X on B **

Like you said, A experiences a force G towards B , given by $\frac{GM_1M_2}{r^2}$. B experiences the same force towards A. These are the 2 forces in this scenario.

Where does X come into the picture. There is no other force X.

In your diagram " the reaction force of G " and X are not two distinct forces. They are the same thing.
Similarly, in your diagram " the reaction force of X " and G are the same thing

Improve this answer
$\endgroup$
7
  • $\begingroup$ How are they same thing?as two different forces are acting so they both will have two different reaction pairs? $\endgroup$
    – Dheeraj Gujrathi
    Commented May 4, 2021 at 11:00
  • 2
    $\begingroup$ @DheerajGujrathi No. the 2 forces are the reaction pairs of each other. $\endgroup$
    – silverrahul
    Commented May 4, 2021 at 11:21
  • 3
    $\begingroup$ @silverrahul I think what Dheeraj is struggling with is that the forces on either side are action and reaction at the same time. Typically, when we start to think about forces, we have the mental concept of an active "action" (I "actively" push a ball), and a "passive" "reaction" (the ball's inertia resists my pushing, so that I feel the same force back). The case of two masses is perfectly symmetrical already in the story-telling: it becomes apparent that the perceived asymmetry in the human example is an illusion; cf. the tug-of-war where one party is replaced by a tree. $\endgroup$
    – Peter - Reinstate Monica
    Commented May 4, 2021 at 14:49
  • 4
    $\begingroup$ The modern meanings of "action" and "reaction" are not the same as when Newton wrote about them. We think of these two words in a cause-and-effect relationship, much like the pain that results from getting hit by a stone. That is NOT what the words meant to Newton. Rather they speak of two different actions which are simultaneous and coincident with each other, the prefix "re" meaning "another of the same type." $\endgroup$
    – Bill N
    Commented May 4, 2021 at 17:26
  • $\begingroup$ @Peter-ReinstateMonica,yes,that is the point I am getting confused at,that each force will have their own reaction pair!!,according to your example,for the tug of war,with one side replaced by tree,force is applied from one side only,but in game of tug of war,force is applied from bith sides!that is where the confusion lies $\endgroup$
    – Dheeraj Gujrathi
    Commented May 5, 2021 at 5:59
5
$\begingroup$

One way to look at this is that forces always come as pairs. For example, you start with a universe with only one object in it, then you add another object and nature will immediately create a pair of forces. It’s not like the Moon feels that the Earth is tugging at it, and retaliates by tugging at Earth itself.

You can’t take such a pair of forces and label one the action and the other the reaction, or one the cause and the other the effect. Rather, both are manifestations of inner workings of nature, and to the best of our current knowledge, those workings aren’t best described in terms of force, but rather as “if things were moving like this before, they’ll be moving like that afterwards”.

Despite not being fundamental, forces are very useful mathematical objects, and the symmetry they exhibit in Newton’s third law is but one among many symmetries the universe has.

Improve this answer
$\endgroup$
1
  • $\begingroup$ ohh thanks,that was a better example of starting universe though,and then adding objects,thanks a lot $\endgroup$
    – Dheeraj Gujrathi
    Commented May 5, 2021 at 6:14
3
$\begingroup$

The meaning of the word "between" in this case is the same as the meaning of between in the sentence:

The love between two people

Of course it is understood that love does not exist in air. One person loves another. Air has no brain and thus has neither emotions nor feelings.

The word between in the sentence above means that person A loves person B and person B loves person A.

Thus the phrase: "the force between two bodies" means body A exerts a force on body B (this force is on body B) and body B exerts a force on body A (this force is on body A).

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Yes, English is weird often preferring to overload a single word with multiple meanings (or worse multiple shades of similar meanings). On the other hand my native language is weird in different ways though often having specific words for each meaning $\endgroup$
    – slebetman
    Commented May 5, 2021 at 4:11
  • $\begingroup$ it was quite a simple explaination though,thanks $\endgroup$
    – Dheeraj Gujrathi
    Commented May 5, 2021 at 6:08
  • 2
    $\begingroup$ This runs counter to the assertion "love is in the air." :P $\endgroup$
    – DKNguyen
    Commented May 5, 2021 at 20:21
2
$\begingroup$

It is not a individual force that exists in the space between them, it is rather saying between in the case that both bodies exert a force on each other, which gradually pulls them to a point between them as they are pulled towards each other, they ultimately simply are counterparts to each other, the equal and opposite reactions.

Improve this answer
$\endgroup$
1
  • $\begingroup$ @yes,i got clearance by reading all answer,once again thanks $\endgroup$
    – Dheeraj Gujrathi
    Commented May 5, 2021 at 6:09
1
$\begingroup$

A (non-fictitious) force is a description of an interaction between 2 objects. So of course, the interaction acts upon both objects with equal magnitudes (Newton's 3rd Law). $A$ acts on $B$ with the same force as $B$ acts on $A$. Gravity is the same. It's an interaction between $A$ and $B$. You could separate it into 2 parts: force by $B$ on $A$ and force by $A$ on $B$. But really, they are 1 interaction.

Sidenote: Gravity as an interaction between $A$ and $B$ is only valid in the most basics of Newtonian mechanics. It gets described as other things later on, but I don't want to confuse you.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Could you please explain how is it described later on!though I am not confused now,well it might give more info. $\endgroup$
    – Dheeraj Gujrathi
    Commented May 14, 2021 at 3:18
  • 1
    $\begingroup$ @DheerajGujrathi For example, you can describe it as the 2 pairs of interactions. One between $A$ and an object call a gravitational field and another between $B$ and the gravitational field. Also, you can refer to general relativity, where gravitational effect is warping of space-time (it's a fictitious force that exist due to the warped space-time). $\endgroup$
    – Michael Huang
    Commented May 16, 2021 at 2:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.