Understanding quantum hard-sphere scattering

Question

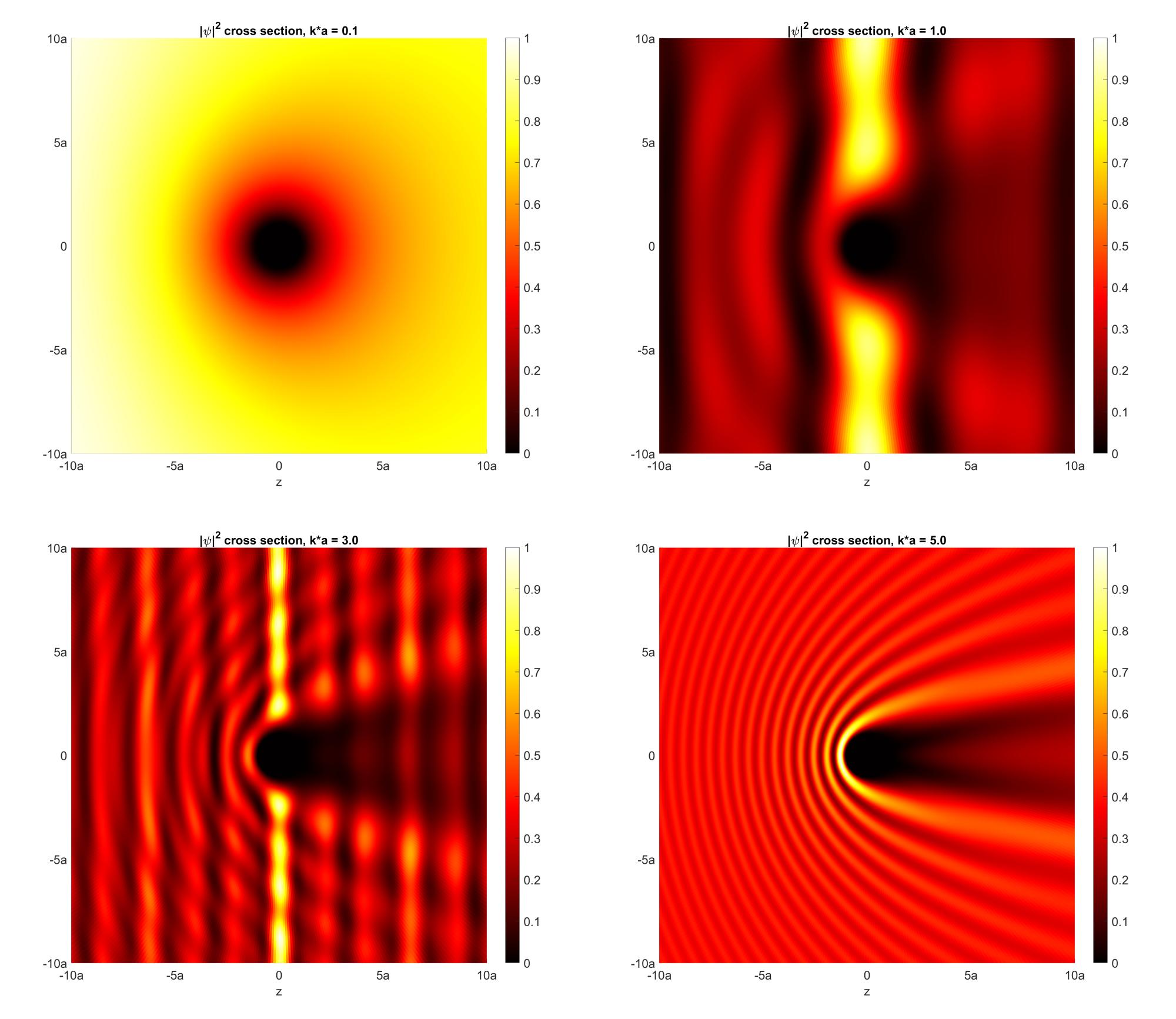
In his chapter on scattering, Griffiths derives the wave function of a scattering particle in terms of partial waves as \begin{equation} \tag{1} \label{wf} \psi(r,\theta) = A\sum_{\ell = 0}^\infty i^\ell (2\ell + 1) \Big[ j_\ell(kr) + ika_\ell h_\ell^{(1)}(kr) \Big] P_\ell(\cos{\theta}) \end{equation} where $k$ is the wavenumber $j_\ell$ is a spherical Bessel function, $h_\ell$ is a Hankel function (first kind), $P_\ell$ is a Legendre polynomial and $a_\ell$ is the scattering amplitude, which is given as \begin{equation} a_\ell = i \frac{j_\ell(ka)}{kh_\ell^{(1)}(ka)} \end{equation} for the simple special case of scattering from a hard sphere (infinite spherical potential) of radius $a$. I've been studying this special case trying to make sense of what the wave function (\ref{wf}) represents and as an aid I've plotted cross sections of the probability density (the first 101 $\ell$-terms) for various values of $ka$ (see below), which I figure is basically the ratio of the radius of the sphere to the wavelength of the particle.

My understanding is that (\ref{wf}) is a superposition of a plane wave traveling in the $z$-direction (representing the incoming particle) and a spherical wave diverging from the potential (representing the scattered particle). I take this to mean that the particle "interferes" with itself during the collision.

What I don't understand is what these images might represent. I would like to think of them as snapshots of the probability distribution (its cross section) for the particles' positions during the scattering process, but I have a feeling this might be the wrong interpretation. Shouldn't a free particle be described as a wave packet rather than a single plane wave? This would then mean that the actual wave function for a scattering particle is a linear combination of (\ref{wf}) with different values of $k$. I guess the images would then not have any physical meaning.

Any help to figure out what I am looking at would be greatly appreciated.

Answer 1

You are solving the time-independent Schrodnger equation (at least approximately). So what you are finding are stationary states of the potential, with boundary conditions specifying that the wavefunction asymptotically far away is a plane wave plus an outgoing spherical wave.

Just like normal, it is useful to find the stationary states because they can form a basis for other solutions. You can create wavepackets as you suggest, by superposing the stationary states you compute.

But you can in fact extract a lot of useful information from the stationary states even without going through the trouble of looking at wavepackets. You can interpret an incoming plane wave as an incoming particle with momentum in the $z$ direction. You can then follow radial paths out from the potential, and think of the amplitude of the outgoing wave as being proportional to the probability that an incoming particle with momentum in the $z$ direction will be scattered in that direction. So, for instance, in your bottom left figure, there is a relatively large amplitude for the particle to be scattered in a direction perpendicular to the $z$ axis represented by the gold vertical line, but the "shadow" behind the scatterer means that it is unlikely for the particle to end up behind the scatterer.