1
$\begingroup$

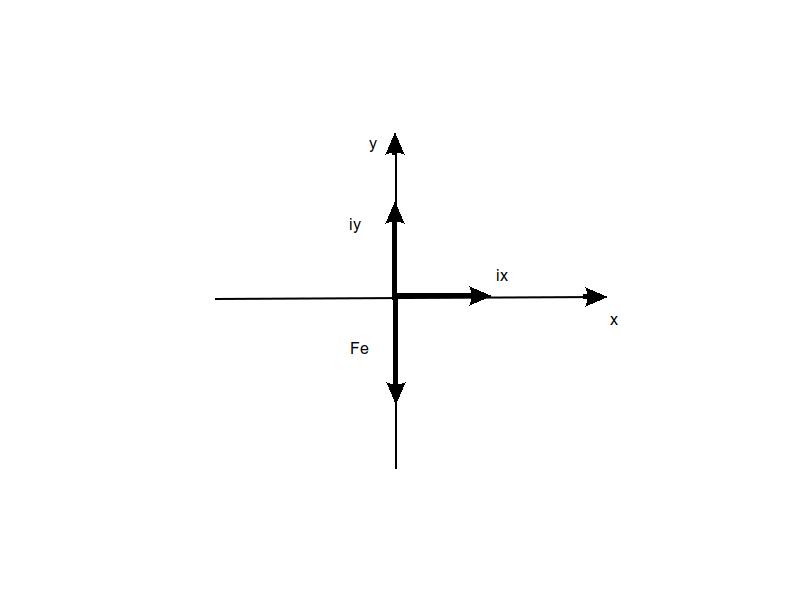
I have a vector $F_e$ and I would like to graph it to its corresponding $X$ and $Y$ components. I know that the $i_y$ component is negative, and there is no $i_x$ component.

$\vec{F_e} = F_x\vec{i_x} + F_y\vec{i_y}$

$\vec{F_e} = F_y\vec{(-i_y)}$

And to get there we have:

$F_{ex}=|\vec{F_{ex}}|\cos(270^\circ)$

$F_{ey}=|\vec{F_{ey}}|\sin(270^\circ)$

My question is when do we use the $\sin(\cdot)$ and when the $\cos(\cdot)$ to find its magnitude. Intuitively $\cos (270^\circ)$ equals $0$ and $\sin (270^\circ)$ equals $-1$. But why didn't we set $F_{ex}$ with $\sin (270^\circ)$. I know that the angle between the $X$ axis and the vector is $270^\circ$.

enter image description here

Improve this question
$\endgroup$

3 Answers 3

Reset to default
5
$\begingroup$

If a point is at a unit distance from the origin, and it makes an angle $p$ with the x-axis, then $\cos p$ is defined as the x co-ordinate of that point. $\sin p$ is defined as the y co-ordinate of that point.

If a point is a distance $r$ from the origin and makes an angle $p$ wih the x-axis, then its x and y co-ordinates are $r \cos p$ and $r\sin p$ respectively.

Since you have to find the x-component of the force here, you have to use $|\vec{F}| \cos p$

Improve this answer
$\endgroup$
4
  • $\begingroup$ This is the thing i was bothered with, it seems simple but why is cos defined with x and sin with y ? $\endgroup$
    – Edmond Dantes
    Commented Apr 21, 2021 at 5:05
  • $\begingroup$ @MarkoMajstorovic Historically, the x co-ordinate has been named cos, and the y has been named sin. Naming is arbitrary. $\endgroup$
    – Ryder Rude
    Commented Apr 21, 2021 at 5:39
  • 1
    $\begingroup$ @MarkoMajstorovic You can think of $\cos {p}$ to mean the sentence: "The x co-ordinate of the point which is at a unit distance from the origin, and makes an angle $p$ with the x-axis". The name $\cos{p}$ has been assigned to this sentence. $\endgroup$
    – Ryder Rude
    Commented Apr 21, 2021 at 5:43
  • 1
    $\begingroup$ @MarkoMajstorovic the standard notation measures angles anticlockwise from the x axis. That is an arbitrary decision, but the definition of "cos" and "sin" then mean "use cos for the x axis and sin for the y axis". Note, the angle convention also works for graphing complex numbers. The formula $e^{i\theta} = \cos\theta + i\sin\theta$ is true by definition, so if you graph complex numbers with the real axis horizontal and the imaginary axis vertical, that fixes how $\theta$ is defined (i.e. measure it anticlockwise from the x axis). $\endgroup$
    – alephzero
    Commented Apr 21, 2021 at 12:53
2
$\begingroup$

So to clarify, you're just trying to

  1. plot the vector using it’s x-y components, or
  2. or are you asking how to get the $X$ or $Y$ components of a vector?

If it’s # 1 it seems like you already did it since there’s no $X$ component and the $Y$ component is in the negative direction. If it’s # 2 then you're probably looking for $x=R\cos\theta$ and $y = R\sin\theta$ where $R$ is the force vectors magnitude and theta is the angle between the vector and the $X$ axis.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Its the first one, but my question is more of a trigonometric one, why didnt we use sin(270) for the x axis of the magnitude $F_{ex}$ $\endgroup$
    – Edmond Dantes
    Commented Apr 21, 2021 at 4:21
  • 1
    $\begingroup$ @MarkoMajstorovic imagine a right triangle with its bottom side on the x-axis. That bottom side is the adjacent and the long side is the hypotenuse. The other end is the opposite, now you can see since it’s aligned, the adjacent is the x and the opposite is the y. The right triangle forms an angle theta with the x axis and by definition sin(theta) =opposite/hypotenuse or y/r $\endgroup$
    – Railmints
    Commented Apr 21, 2021 at 4:31
2
$\begingroup$

Recall the triangle law of vectors.

To find the x and y components, construct a triangle with the vector $F_e$ as the hypotenuse.

This is where trigonometry comes in- since $F_{ex}$ is the base of the triangle and $F_e$ is the hypotenuse, you can now figure out the trigonometric relation between $\theta$, $F_e$ and $F_{ex}$.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I mean this is not a homework question more of a how did they get that type of question, and how can i make Fe to be the hypotenuse ? $\endgroup$
    – Edmond Dantes
    Commented Apr 21, 2021 at 4:36
  • $\begingroup$ @MarkoMajstorovic in regular triangles the hypotenuse will be made in a regular form, but in this case it will overlap with y-axis(as you can see) and the x-axis will be reduced to zero. $\endgroup$
    – Sid
    Commented Apr 21, 2021 at 4:42
  • $\begingroup$ To visualise: Imagine a regular right-angled triangle with $\theta = 45 \circ$. Now increase the angle between x-axis and hypotenuse, while keeping the hypotenuse constant. You can see that the length of the base would decrease, right? And at 90 deg., it would be just a line along the y-axis. Increasing it further, the triangle would go into the second quadrant.At 180 deg., it would be just a straight line along the negative x- axis. At 270, just a straight line along negative y-axis. $\endgroup$
    – Sid
    Commented Apr 21, 2021 at 4:47
  • $\begingroup$ @MarkoMajstorovic mathsisfun.com/algebra/trig-interactive-unit-circle.html is a good visualisation $\endgroup$
    – Sid
    Commented Apr 21, 2021 at 4:56

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.