Is there a loss of mechanical energy when a permanent magnet falls trough a coil?
Given a magnet with mass m and a coil with N windings. The coil is placed vertically so the magnet can fall trough. The magnet is dropped from a height H, at this point the mehchanical energy is equal to mgH. I want to calculate the mechanical energy at the bottom of the coil. I assume energy is conserved so this should also be equal to mgH. Is the energy conserved? if not, would I be able to calculate the loss?
Thanks in advance!

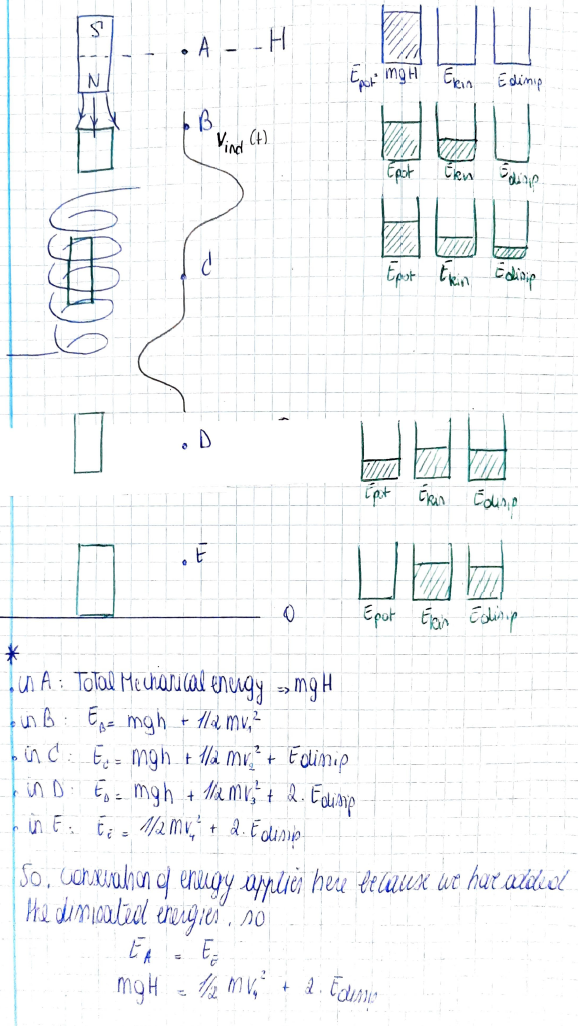
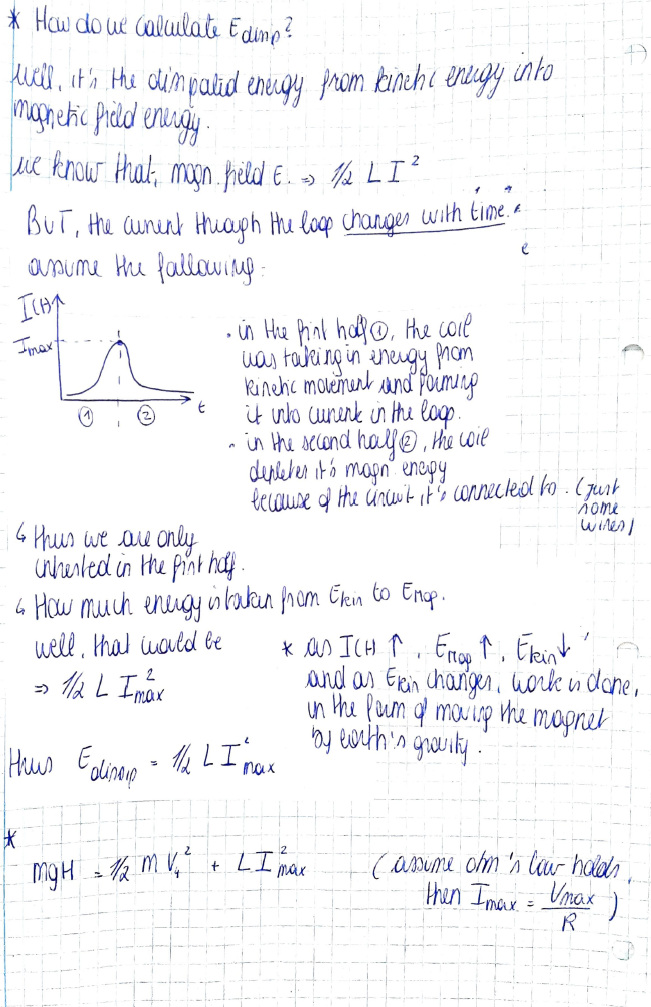 obviously, you would need maxwell's third equation (faraday's law) to calculate the induced voltage on the coil.
I assumed too that there is no air friction and that the energy consversion from one to the other is 100% efficient with no loss whatsoever.
obviously, you would need maxwell's third equation (faraday's law) to calculate the induced voltage on the coil.
I assumed too that there is no air friction and that the energy consversion from one to the other is 100% efficient with no loss whatsoever.