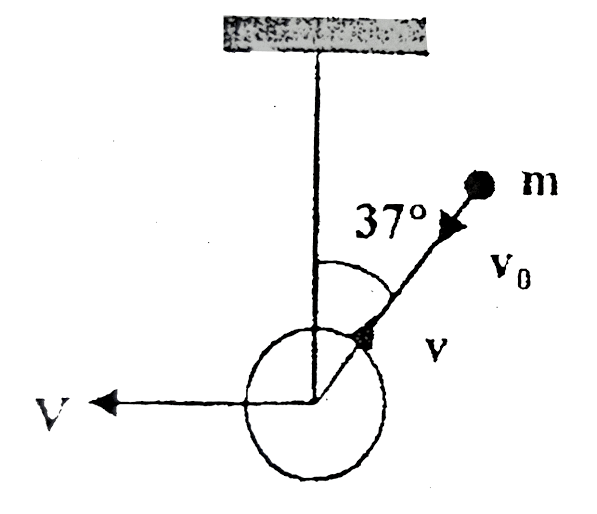
Can someone explain along which direction the linear momentum of these colliding balls should be conserved? Is it conserved along the direction where the net force is zero? Someone please clarify my doubts.
$\begingroup$
$\endgroup$
2
-
$\begingroup$ The diagram is unclear; is the smaller ball moving downwards at 37deg. and the bigger ball moving leftward? Also is the collision inelastic? $\endgroup$– SidCommented Apr 16, 2021 at 11:19
-
$\begingroup$ Yes, it is an inelastic collision . $\endgroup$– Latha PremCommented Apr 16, 2021 at 16:09
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
Typically momentum is conserved along all directions. But it is interesting to look at the contact normal direction, and trivial along any slip plane. So we tend to focus on the contact normal direction because it is more informative (in general).
But because the hanging ball is connected to the ground you cannot use conservation of momentum. At least not the components of momentum tangent to the string.
So for this case look at conservation of momentum along the horizontal plane, as the impact components along this direction would not impart any reaction impulses from the attachment.
What happens during an impact is that there is little morsel of momentum being exchanged between that part through the contact point, and along the contact normal direction. This amount (called an impulse) is added and subtracted in equal amounts between the two bodies so overall the result no change in total momentum.
answered Apr 16, 2021 at 12:04
$\begingroup$
$\endgroup$
Short answer: It is conserved in all directions.
Long answer:
The final momentum is determined by the sum of the momenta of the different objects. You have to understand that momentum has components, along the separate x and y axes. These axes can be anywhere, for the sake of simplicity, let us assume the vertical axis as y, and horizontal axis as x.
The larger ball has momentum only in the x-axis and none in the y-axis(for obvious reasons). However, the smaller ball is moving at an angle to both axes, and it therefore has nonzero x and y components.
In the triangle law of vectors, the velocity $v$ of the ball is taken as the hypotenuse of the triangle formed; the perpendicular is the y-component and the base is the x-component, like this:
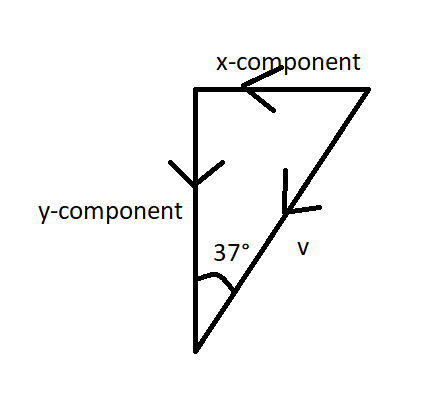
Through basic trigonometry, one knows that the x-component $v_x = v\sin 37°$ and the y component $v_y = v \cos 37°$.
Now the important point is that the components of the momenta are to be added separately. So the x-component of the momentum of the combined mass is the sum of the x-components of the two separate masses. Similarly for the y-component.
So the combined mass has a momentum with both x and y components, and using basic trigonometry one can find the momentum's magnitude and angle.
I hope I answered your question!
