Tension of rope in the gravitational field of earth

Question

Two balls of mass $m$ each one are connected with mass-less rope with the same length as the radius of earth. The system is in free fall. Prove that the tension of the rope when the nearest (to the earth) ball's distance from the earth surface is $R_E/2$ is: $T = \frac{32}{225} mg$

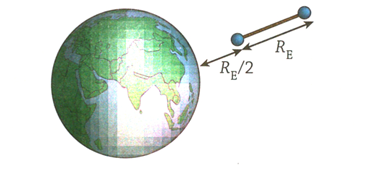
What I did is the following:

$F_1$ is a gravitation force exerted on the nearest ball by the earth: $F_1=G \frac{M_Em}{(1.5R_E)^2}$

$F_2$ is a gravitation force exerted on the farthest ball by the earth: $F_2=G \frac{M_E m}{(2.5R_E)^2}$

$T=F_1-F_2=G \frac{M_E m}{(1.5R_E)^2}-G \frac{M_E m}{(2.5R_E)^2}=\frac{G M_E m}{R_E^2} \left (\frac{4}{9} - \frac{4}{25} \right)=\frac{64}{225} mg$

However, my answer is somehow twice bigger than what is expected. Where am I wrong? What am I missing?

Answer 1

Gotcha covered:

$$F_1=G{M_E m\over(1.5R_E)^2}\mathbf {-T}$$ -T from upwards force of rope. $$F_2=G{M_Em\over(2.5R_E)^2}\mathbf {+T}$$ +T from downwards force of rope.

Then since the rope isn't stretching,

$$F_1~=~F_2$$ $$2T~=~G{M_E m\over(1.5R_E)^2}-G{M_Em\over(2.5R_E)^2}~=~{64\over225}mg$$ $$\therefore~T~=~{32\over225}mg$$